Thermodynamics Questions | JEE Main Chemistry Chapter Wise Question Papers

Q 1 :

Three moles of an ideal gas are compressed isothermally from 60 L to 20 L using constant pressure of 5 atm. Heat exchange Q for the compression is – ________ Lit. atm. [2024]

(200)

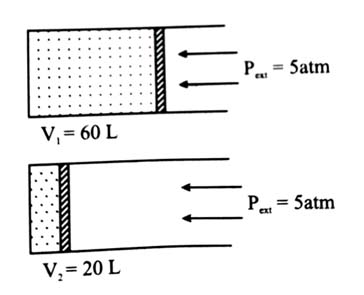
$w = - P_{ext} Δ V = - P_{ext} (V_{2} - V_{1}) = - 5 (20 - 60) atm L = 200 atm L$

By first law of thermodynamics:

$Δ V = - w + q$

For isothermal process, $Δ U = 0,$ so $q = - w = - 200 atm L$

Q 2 :

An ideal gas, ${\vec{C}}_{v} = \frac{5}{2} R,$ is expanded adiabatically against a constant pressure of 1 atm until it doubles in volume. If the initial temperature and pressure is 298 K and 5 atm, respectively then the final temperature is __________ K (nearest integer). [ ${\vec{c}}_{v}$ is the molar heat capacity at constant volume] [2024]

(274)

Work done (W) by gas against a constant pressure is given by:

$W = - P_{ext} (V_{f} - V_{i}) = - 1 (2 V - V) = - V$ ...(i)

Change in internal energy ( $∆ U$ ) for an ideal gas undergoing any process is:

$Δ U = n C_{v} (T_{f} - T_{i}) = n \times \frac{5}{2} R (T_{f} - 298)$ ...(ii)

By first law of thermodynamics:

$Δ U = q + W$

Since q = 0 for an adiabatic process,

$Δ U = W$

Put $∆ U$ from (ii) and W from (i)

$n \times \frac{5}{2} R (T_{f} - 298) = - V$ ...(iii)

Apply ideal gas equation at initial conditions,

$P_{i} V_{i} = n R T_{i}$

$5 V = n R \times 298$

$V = \frac{n R \times 298}{5}$ ...(iv)

Put V from (iv) in (iii)

$n \times \frac{5}{2} R (T_{f} - 298) = - \frac{n R \times 298}{5}$

$\frac{5}{2} (T_{f} - 298) = - \frac{298}{5}$

$T_{f} - 298 = - \frac{298 \times 2}{5 \times 5}$

$T_{f} = 274.16 \approx 274 K$

Q 3 :

A sample of $n$ -octane (1.14 g) was completely burnt in excess of oxygen in a bomb calorimeter, whose heat capacity is 5 kJ $K^{- 1}$ . As a result of combustion reaction, the temperature of the calorimeter is increased by 5 K. The magnitude of the heat of combustion of octane at constant volume is ____ kJ ${mol}^{- 1}$ (nearest integer). [2025]

(2500)

$Number of moles of octane (n) = \frac{Mass of octane}{Molar mass of octane} = \frac{1.14}{114} mol = 0.01 mol$

$Heat evolved (Q) = C Δ T = 5 {kJ K}^{- 1} \times 5 K = 25 kJ$

$Magnitude of heat of combustion = \frac{Q}{n} = \frac{25 kJ}{0.01 mol} = 2500 kJ/mol$

Q 4 :

0.3 g of ethane undergoes combustion at 27°C in a bomb calorimeter. The temperature of calorimeter system (including the water) is found to rise by 0.5°C. The heat evolved during combustion of ethane at constant pressure is ______ kJ ${mol}^{- 1}$ . (Nearest integer) [Given: The heat capacity of the calorimeter system is 20 kJ $K^{- 1}$ , R = 8.3 J $K^{- 1}$ ${mol}^{- 1}$ ]. Assume ideal gas behaviour. Atomic mass of C and H are 12 and 1 g ${mol}^{- 1}$ respectively] [2023]

(1006)

Moles of ethane = 0.01

$Q = C Δ T = - 1000 kJ/mol$

$Δ H = Δ E + Δ n_{g} R T$

$Δ n_{g} = - \frac{5}{2}$

$Δ H = - 1006 kJ/mol$

Q 5 :

When a 60 W electric heater is immersed in a gas for 100s in a constant volume container with adiabatic walls, the temperature of the gas rises by 5°C. The heat capacity of the given gas is ______ J $K^{- 1}$ . (Nearest Integer) [2023]

(1200)

$q_{v} = C_{V} Δ T$

$60 \times 100 = C_{V} \times 5$

$C_{V} = {1200 J K}^{- 1}$

Q 6 :

Given below are two statements:

Statement I: For an ideal gas, heat capacity at constant volume is always greater than the heat capacity at constant pressure.

Statement II: In a constant volume process, no work is produced and all the heat withdrawn goes into the chaotic motion and is reflected by a temperature increase of the ideal gas.

In the light of the above statements choose the correct answer from the options given below: [2026]

Both Statement I and Statement II are true
Both Statement I and Statement II are false
Statement I is true but Statement II is false
Statement I is false but Statement II is true

1

2

3

4

(4)

$C_{P}$ is greater than $C_{V}$ because for the same temperature rise (i.e. same $Δ U$ ) at constant pressure, some of heat is consumed in doing expansion work.

$Q_{P} = Δ U + P Δ V$

$n C_{P} Δ T = Δ U + n R Δ T$ $. . . (I)$

Heat supplied at constant volume is used only to raise the temperature of the system and no work is done.

$Q_{V} = Δ U$

$n C_{V} Δ T = Δ U$ $. . . (I I)$

Put $Δ U$ from II in I

$n C_{P} Δ T = n C_{V} Δ T + n R Δ T$

$C_{P} - C_{V} = R \Rightarrow C_{P} > C_{V}$

Q 7 :

The correct order of molar heat capacities measured at 298 K and 1 bar is: [2026]

$Copper (s) > Bromine (l) > Helium (g)$
$Bromine (l) > Copper (s) > Helium (g)$
$Helium (g) > Bromine (l) > Copper (s)$
$Helium (g) > Bromine (l) = Copper (s)$

1

2

3

4

(2)

Molar heat capacity of liquids is generally greater than solids because liquids have greater degrees of freedom and hence can store more energy for the same temperature rise. Molar heat capacity for monoatomic gases is low as for monoatomic gases only translational degrees of freedom are present. Hence order of molar heat capacities is:

${Br}_{2} (l) > Cu (s) > He (g)$

Topic Question Set

Q 1 :

Three moles of an ideal gas are compressed isothermally from 60 L to 20 L using constant pressure of 5 atm. Heat exchange Q for the compression is – ________ Lit. atm. [2024]

Q 5 :

When a 60 W electric heater is immersed in a gas for 100s in a constant volume container with adiabatic walls, the temperature of the gas rises by 5°C. The heat capacity of the given gas is ______ J $K^{- 1}$ . (Nearest Integer) [2023]

Q 7 :

The correct order of molar heat capacities measured at 298 K and 1 bar is: [2026]

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 1 : Three moles of an ideal gas are compressed isothermally from 60 L to 20 L using constant pressure of 5 atm. Heat exchange Q for the compression is – ________ Lit. atm. [2024]

Q 5 : When a 60 W electric heater is immersed in a gas for 100s in a constant volume container with adiabatic walls, the temperature of the gas rises by 5°C. The heat capacity of the given gas is ______ J K-1. (Nearest Integer) [2023]

Q 7 : The correct order of molar heat capacities measured at 298 K and 1 bar is: [2026]

Want Us to Email you About Special Offers & Updates?

Q 1 :

Three moles of an ideal gas are compressed isothermally from 60 L to 20 L using constant pressure of 5 atm. Heat exchange Q for the compression is – ________ Lit. atm. [2024]

Q 5 :

When a 60 W electric heater is immersed in a gas for 100s in a constant volume container with adiabatic walls, the temperature of the gas rises by 5°C. The heat capacity of the given gas is ______ J $K^{- 1}$ . (Nearest Integer) [2023]

Q 7 :

The correct order of molar heat capacities measured at 298 K and 1 bar is: [2026]