Thermodynamics Questions | JEE Main Chemistry Chapter Wise Question Papers

Q 1 :

Choose the correct option for free expansion of an ideal gas under adiabatic condition from the following: [2024]

$q = 0, Δ T \neq 0, w = 0$
$q = 0, Δ T < 0, w \neq 0$
$q \neq 0, Δ T = 0, w = 0$
$q = 0, Δ T = 0, w = 0$

1

2

3

4

(4)

For adiabatic process $q = 0$ .

$W = - P_{ext} Δ V, for free expansion, P_{ext} = 0, so w = 0$

By first law of thermodynamics:

$Δ U = W + q$

$Δ U = 0 + 0 = 0$

For an ideal gas internal energy is function of temperature, so if $Δ U = 0, Δ T$ is also 0.

Q 2 :

If 5 moles of an ideal gas expands from 10 L to a volume of 100 L at 300 K under isothermal and reversible condition then work, w, is $- x$ J. The value of $x$ is _________ .

(Given R = 8.314 J $K^{- 1}$ $m o l^{- 1}$ ) [2024]

(28721)

For isothermal reversible process, work W is given by:

$W = - 2.303$ $n R T$ $\log$ $\frac{V_{2}}{V_{1}}$

$= - 2.303 \times 5$ $mol \times 8.314 \frac{J}{mol K} \times 300$ $K$ $l o g$ $\frac{100}{10}$

$= - 28720.713$ $J \approx - 28721 J$

Q 3 :

The heat of combustion of solid benzoic acid at constant volume is –321.30 kJ at 27°C. The heat of combustion at constant pressure is (–321.30 – $x$ R) kJ, the value of $x$ is _________ . [2024]

(150)

$C_{6} H_{5} COOH (s) + \frac{15}{2} O_{2} (g) \to 7 {CO}_{2} (g) + 3 H_{2} O (l)$

$Δ n_{g} = Gaseous moles of products - Gaseous moles of reactants$

$= 7 - 7.5 = - 0.5$

$Heat of combustion at constant pressure (Δ H) is related to heat of combustion at constant volume (Δ U) by :$

$Δ H = Δ U + Δ n_{g} R T$

$Δ H = - 321.3 - 0.5 R \times 300$

$Δ H = - 321.3 - 150 R$

Q 4 :

$Δ_{vap} H^{Θ}$ for water is + 40.79 kJ $m o l^{- 1}$ at 1 bar and 100°C. Change in internal energy for this vapourisation under same condition is ________ kJ $m o l^{- 1}$ . (Integer answer)

(Given R = 8.3 $J K^{- 1}$ $m o l^{- 1}$ ) [2024]

(38)

$H_{2} O (l) ⇌ H_{2} O (g)$

$Δ n_{g} = Gaseous moles of products - Gaseous moles of reactants$

$= 1 - 0 = 1$

$Δ H = Δ U + Δ n_{g} R T$

$40.79 {kJmol}^{- 1} = Δ U + 1 \times 8.3 \frac{J}{molK} \times 373 K$

$40.79 {kJmol}^{- 1} = Δ U + 1 \times \frac{8.3}{1000} \frac{kJ}{molK} \times 373 K$

$Δ U = 37.69 {kJmol}^{- 1} \approx 38 {kJmol}^{- 1}$

Q 5 :

If three moles of an ideal gas at 300 K expand isothermally from 30 ${dm}^{3}$ to 45 ${dm}^{3}$ against a constant opposing pressure of 80 kPa, then the amount of heat transferred is _____ J. [2024]

(1200)

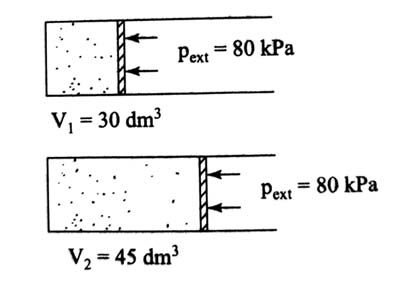
Work (W) against constant pressure is calculated as:

$W = - P_{ext} (V_{2} - V_{1}) = - 80 kPa \times (45 - 30) {dm}^{3}$

$= - 80 \times 1000 Pa \times 15 \times {(10^{- 1} m)}^{3}$

$= - 1200 J$

By first law of thermodynamics:

$Δ U = Q + W$

For isothermal process of an ideal gas, $Δ U = 0$

So, $Q = - W$

$Q = - (- 1200 J) = 1200 J$

Hence 1200 J of heat is absorbed in the process.

Q 6 :

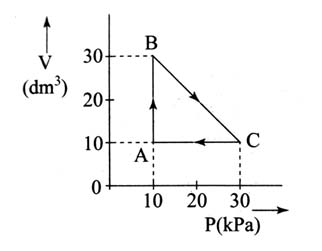
An ideal gas undergoes a cyclic transformation starting from the point A and coming back to the same point by tracing the path $A \to B \to C \to A$ as shown in the diagram above. The total work done in the process is _____ J. [2024]

(200)

Magnitude of work done in a cyclic process = area enclosed by P-V or V-P graph.

$| W |$ $= \frac{1}{2} \times A C \times A B$

$= \frac{1}{2} \times (30 - 10) kPa \times (30 - 10) {dm}^{3}$

$= 200 {kPadm}^{3}$

$= 200 \times 1000 Pa \times {(10^{- 1} m)}^{3}$

$= 200 {Pam}^{3} = 200 J$

Q 7 :

Consider the figure provided.

1 mol of an ideal gas is kept in a cylinder, fitted with a piston, at the position A, at 18°C. If the piston is moved to position B, keeping the temperature unchanged, then ‘x’ L atm work is done in this reversible process.

x = _____ L atm. (nearest integer)

[Given: Absolute temperature = °C + 273.15,
R = 0.08206 L atm ${mol}^{- 1}$ $K^{- 1}$ ] [2024]

(55)

Work done by gas in isothermal reversible expansion is:

$w = - 2.303 n R T \log_{10} \frac{V_{2}}{V_{1}}$

$w = - 2.303 \times 1 \times 0.08206 \times 291.15 \times \log_{10} \frac{100}{10} atmL$

$w = - 2.303 \times 1 \times 0.08206 \times 291.15 \times \log_{10} 10 atmL$

$w = - 55.023 atmL \approx - 55 atmL$

Work done by system = +55 atmL

Q 8 :

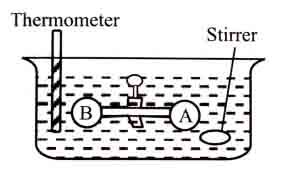
Two vessels A and B are connected via stopcock.

The vessel A is filled with a gas at a certain pressure. The entire assembly is immersed in water and is allowed to come to thermal equilibrium with water. After opening the stopcock the gas from vessel A expands into vessel B and no change in temperature is observed in the thermometer. Which of the following statement is true? [2025]

$d w \neq 0$
$d q \neq 0$
$d U \neq 0$
The pressure in the vessel B before opening the stopcock is zero.

1

2

3

4

(4)

As the process is isothermal, q = 0. As it is free expansion $(P_{ext} = 0),$ $w = - P_{ext} Δ V = 0 .$ By first law of thermodynamics, $Δ U = q + w = 0 .$

Q 9 :

One mole of an ideal gas expands isothermally and reversibly from 10 ${dm}^{3}$ to 20 ${dm}^{3}$ at 300 K. $ΔU$ , $q$ and work done in the process respectively are:

Given: R = 8.3 J $K^{- 1}$ ${mol}^{- 1}$

ln 10 = 2.3
log 2 = 0.30
log 3 = 0.48 [2025]

0, 21.84 kJ, –1.26 kJ
0, –17.18 kJ, 1.718 J
0, 21.84 kJ, 21.84 kJ
0, 1.78 kJ, –1.78 kJ

1

2

3

4

(4)

$w = - 2.303 n R T \log_{10} \frac{V_{2}}{V_{1}}$

$w = - 2.303 \times 1 \times 8.3 \times 300 \log_{10} \frac{20}{10} J$

$w = - 2.303 \times 1 \times 8.3 \times 300 \log_{10} 2 J$

$w = - 2.303 \times 1 \times 8.3 \times 300 \times 0.3 J$

$w = - 1720.341 = - 1.72 kJ$

For an ideal gas undergoing any process: $Δ U = n C_{v} Δ T$

For isothermal process $Δ T = 0$ . So $Δ U = 0$ .

By first law of thermodynamics: $Δ U = q + w$

$0 = q + w$

$q = - w = - (- 1.72 kJ) = 1.72 kJ$

Q 10 :

A liquid when kept inside a thermally insulated closed vessel at 25°C was mechanically stirred from outside. What will be the correct option for the following thermodynamic parameters? [2025]

$Δ U = 0, q < 0, w > 0$
$Δ U > 0, q = 0, w > 0$
$Δ U < 0, q = 0, w > 0$
$Δ U = 0, q = 0, w = 0$

1

2

3

4

(2)

Since work is done on the system, it is positive, $w > 0$ .

Since vessel is thermally insulated, $q = 0$ .

By first law of thermodynamics

$Δ U = q + w$

$Δ U = 0 + w = w$

As $w > 0$ , $Δ U > 0$

Q 11 :

Arrange the following in order of magnitude of work done by the system / on the system at constant temperature:

a) $| w_{reversible} |$ for expansion in infinite stage.
b) $| w_{irreversible} |$ for expansion in single stage.
c) $| w_{reversible} |$ for compression in infinite stage.
d) $| w_{irreversible} |$ for compression in single stage.

Choose the correct answer from the options given below: [2025]

a > b > c > d
d > c = a > b
c = a > d > b
a > c > b > d

1

2

3

4

(2)

Magnitude of work = area under PV graph

Q 12 :

A perfect gas (0.1 mol) having $C_{v}$ = 1.50R (independent of temperature) undergoes the above transformation from point 1 to point 4. If each step is reversible, the total work done $(w)$ while going from point 1 to point 4 is (–) ___ J (nearest integer).

[Given : R = 0.082 L atm $K^{- 1}$ ${mol}^{- 1}$ ] [2025]

(304)

$1 \to 2$ and $3 \to 4$ are isochoric processes. For isochoric processes $W (P Δ V) = 0$

$W_{2 \to 3} = - P (V_{3} - V_{2})$

$= - 3 atm (2 L - 1 L)$

$= - 3 atm L = - 3 \times 101.3 J = - 303.9 J$

$W_{1 \to 4} = W_{1 \to 2} + W_{2 \to 3} + W_{3 \to 4}$

$= 0 - 303.9 J + 0 = - 303.9 J = - 304 J$

Q 13 :

One mole of an ideal monoatomic gas is subjected to changes as shown in the graph. The magnitude of the work done (by the system or on the system) is ______ J (nearest integer) [2023]

Given: log 2 = 0.3
ln 10 = 2.3

(620)

$W_{1 \to 2} = - 1 (40 - 20) = -20 bar lit.$

$W_{2 \to 3} = 0$

$W_{3 \to 1} = - n R T \ln (\frac{V_{2}}{V_{1}})$

$= - P_{1} V_{1} \ln (\frac{V_{2}}{V_{1}})$

$= - 1 \times 20 ln \frac{20}{40}$

$= 20 ln 2$

$= 20 \times 2.3 \times 0.3 = 13.8 bar lit.$

$W_{total} = - 20 + 13.8$

$= - 6.2 bar lit. (1 bar lit.=100 J)$

$W_{total} = - 6.2 \times 100 = -620 J$

Q 14 :

When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is _________ J. (Nearest Integer) [2023]

(0)

$Δ U = \frac{F}{2} n R Δ T (where F = degree of freedom)$

$As process is isothermal, Δ T = 0$

so $Δ U = 0$

Q 15 :

1 mole of ideal gas is allowed to expand reversibly and adiabatically from a temperature of 27°C. The work done is 3 kJ ${mol}^{- 1}$ . The final temperature of the gas is ______ K (Nearest integer). Given $C_{v}$ = 20 J ${mol}^{- 1}$ $K^{- 1}$ . [2023]

(150)

$W = - q = - n C_{V} [T_{2} - T_{1}] = 3 \times 10^{3} J$

$\Rightarrow - 1 \times 20 [T_{2} - 300] = 3 \times 1000$

$\Rightarrow - (T_{2} - 300) = \frac{3000}{20}$

$∴$ $T_{2} = 150 K$

Q 16 :

A cup of water at 5°C (system) is placed in a microwave oven and the oven is turned on for one minute during which the water begins to boil. Which of the following option is true? [2026]

$q = + ve, w = - ve, Δ U = - ve$
$q = + ve, w = - ve, Δ U = + ve$
$q = - ve, w = - ve, Δ U = - ve$
$q = + ve, w = 0, Δ U = - ve$

1

2

3

4

(2)

$\underset{5 ° C}{H_{2} O (ℓ)} \to \underset{100 ° C}{H_{2} O (ℓ)} ⇄ \underset{100 ° C}{H_{2} O (g)}$

due to expansion

$w = - v e$

as heat is given to system so $q = + ve$ and internal energy of gas will be more than internal energy of liquid so $Δ U = + v e$

Q 17 :

20.0 $d m ³$ of an ideal gas ‘X’ at 600 K and 0.5 MPa undergoes isothermal reversible expansion until pressure of the gas is 0.2 MPa. Which of the following option is correct?

(Given: log 2 = 0.3010 and log 5 = 0.6989) [2026]

$w = 9.1 J, Δ U = 9.1 J, Δ H = 0; q = 0$
$w = - 9.1 k J, Δ U = 0, Δ H = 0, q = 9.1 k J$
$w = + 4.1 k J, Δ U = 0, Δ H = 0; q = - 4.1 k J$
$w = - 3.9 k J, Δ U = 0, Δ H = 0; q = 3.9 k J$

1

2

3

4

(2)

$For isothermal reversible process Δ U = Δ H = 0$

$w_{iso} = - p_{1} V_{1} \ln \frac{P_{1}}{P_{2}}$

$w_{iso} = - 0.5 \times 10^{6} \times 20 \times 10^{- 3} \ln \frac{0.5}{0.2}$

$w_{iso} = - 0.5 \times 10^{6} \times 20 \times 10^{- 3} \times 2.303 \times (0.6989 - 0.3010)$

$w \approx - 9.1 kJ$

$q = - w = 9.1 kJ$

Topic Question Set

Q 1 :

Choose the correct option for free expansion of an ideal gas under adiabatic condition from the following: [2024]

Q 2 :

If 5 moles of an ideal gas expands from 10 L to a volume of 100 L at 300 K under isothermal and reversible condition then work, w, is $- x$ J. The value of $x$ is _________ .

(Given R = 8.314 J $K^{- 1}$ $m o l^{- 1}$ ) [2024]

Q 3 :

The heat of combustion of solid benzoic acid at constant volume is –321.30 kJ at 27°C. The heat of combustion at constant pressure is (–321.30 – $x$ R) kJ, the value of $x$ is _________ . [2024]

Q 4 :

$Δ_{vap} H^{Θ}$ for water is + 40.79 kJ $m o l^{- 1}$ at 1 bar and 100°C. Change in internal energy for this vapourisation under same condition is ________ kJ $m o l^{- 1}$ . (Integer answer)

(Given R = 8.3 $J K^{- 1}$ $m o l^{- 1}$ ) [2024]

Q 5 :

If three moles of an ideal gas at 300 K expand isothermally from 30 ${dm}^{3}$ to 45 ${dm}^{3}$ against a constant opposing pressure of 80 kPa, then the amount of heat transferred is _____ J. [2024]

Q 6 :

An ideal gas undergoes a cyclic transformation starting from the point A and coming back to the same point by tracing the path $A \to B \to C \to A$ as shown in the diagram above. The total work done in the process is _____ J. [2024]

Q 9 :

One mole of an ideal gas expands isothermally and reversibly from 10 ${dm}^{3}$ to 20 ${dm}^{3}$ at 300 K. $ΔU$ , $q$ and work done in the process respectively are:

Given: R = 8.3 J $K^{- 1}$ ${mol}^{- 1}$

ln 10 = 2.3
log 2 = 0.30
log 3 = 0.48 [2025]

Q 10 :

A liquid when kept inside a thermally insulated closed vessel at 25°C was mechanically stirred from outside. What will be the correct option for the following thermodynamic parameters? [2025]

Q 13 :

One mole of an ideal monoatomic gas is subjected to changes as shown in the graph. The magnitude of the work done (by the system or on the system) is ______ J (nearest integer) [2023]

Given: log 2 = 0.3
ln 10 = 2.3

Q 14 :

When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is _________ J. (Nearest Integer) [2023]

Q 15 :

1 mole of ideal gas is allowed to expand reversibly and adiabatically from a temperature of 27°C. The work done is 3 kJ ${mol}^{- 1}$ . The final temperature of the gas is ______ K (Nearest integer). Given $C_{v}$ = 20 J ${mol}^{- 1}$ $K^{- 1}$ . [2023]

Q 16 :

A cup of water at 5°C (system) is placed in a microwave oven and the oven is turned on for one minute during which the water begins to boil. Which of the following option is true? [2026]

Q 17 :

20.0 $d m ³$ of an ideal gas ‘X’ at 600 K and 0.5 MPa undergoes isothermal reversible expansion until pressure of the gas is 0.2 MPa. Which of the following option is correct?

(Given: log 2 = 0.3010 and log 5 = 0.6989) [2026]

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 1 : Choose the correct option for free expansion of an ideal gas under adiabatic condition from the following: [2024]

Q 2 : If 5 moles of an ideal gas expands from 10 L to a volume of 100 L at 300 K under isothermal and reversible condition then work, w, is -x J. The value of x is _________ . (Given R = 8.314 J K-1 mol-1) [2024]

Q 3 : The heat of combustion of solid benzoic acid at constant volume is –321.30 kJ at 27°C. The heat of combustion at constant pressure is (–321.30 – xR) kJ, the value of x is _________ . [2024]

Q 4 : ΔvapHΘ for water is + 40.79 kJ mol-1 at 1 bar and 100°C. Change in internal energy for this vapourisation under same condition is ________ kJ mol-1. (Integer answer) (Given R = 8.3 JK-1 mol-1) [2024]

Q 5 : If three moles of an ideal gas at 300 K expand isothermally from 30 dm3 to 45 dm3 against a constant opposing pressure of 80 kPa, then the amount of heat transferred is _____ J. [2024]

Q 6 : An ideal gas undergoes a cyclic transformation starting from the point A and coming back to the same point by tracing the path A→B→C→A as shown in the diagram above. The total work done in the process is _____ J. [2024]

Q 9 : One mole of an ideal gas expands isothermally and reversibly from 10 dm3 to 20 dm3 at 300 K. ΔU, q and work done in the process respectively are: Given: R = 8.3 J K-1 mol-1 ln 10 = 2.3 log 2 = 0.30 log 3 = 0.48 [2025]

Q 10 : A liquid when kept inside a thermally insulated closed vessel at 25°C was mechanically stirred from outside. What will be the correct option for the following thermodynamic parameters? [2025]

Q 13 : One mole of an ideal monoatomic gas is subjected to changes as shown in the graph. The magnitude of the work done (by the system or on the system) is ______ J (nearest integer) [2023] Given: log 2 = 0.3 ln 10 = 2.3

Q 14 : When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is _________ J. (Nearest Integer) [2023]

Q 15 : 1 mole of ideal gas is allowed to expand reversibly and adiabatically from a temperature of 27°C. The work done is 3 kJ mol-1. The final temperature of the gas is ______ K (Nearest integer). Given Cv = 20 J mol-1 K-1. [2023]

Q 16 : A cup of water at 5°C (system) is placed in a microwave oven and the oven is turned on for one minute during which the water begins to boil. Which of the following option is true? [2026]

Q 17 : 20.0 dm³ of an ideal gas ‘X’ at 600 K and 0.5 MPa undergoes isothermal reversible expansion until pressure of the gas is 0.2 MPa. Which of the following option is correct? (Given: log 2 = 0.3010 and log 5 = 0.6989) [2026]

Want Us to Email you About Special Offers & Updates?

Q 1 :

Choose the correct option for free expansion of an ideal gas under adiabatic condition from the following: [2024]

Q 2 :

If 5 moles of an ideal gas expands from 10 L to a volume of 100 L at 300 K under isothermal and reversible condition then work, w, is $- x$ J. The value of $x$ is _________ .

(Given R = 8.314 J $K^{- 1}$ $m o l^{- 1}$ ) [2024]

Q 3 :

The heat of combustion of solid benzoic acid at constant volume is –321.30 kJ at 27°C. The heat of combustion at constant pressure is (–321.30 – $x$ R) kJ, the value of $x$ is _________ . [2024]

Q 4 :

$Δ_{vap} H^{Θ}$ for water is + 40.79 kJ $m o l^{- 1}$ at 1 bar and 100°C. Change in internal energy for this vapourisation under same condition is ________ kJ $m o l^{- 1}$ . (Integer answer)

(Given R = 8.3 $J K^{- 1}$ $m o l^{- 1}$ ) [2024]

Q 5 :

If three moles of an ideal gas at 300 K expand isothermally from 30 ${dm}^{3}$ to 45 ${dm}^{3}$ against a constant opposing pressure of 80 kPa, then the amount of heat transferred is _____ J. [2024]

Q 6 :

An ideal gas undergoes a cyclic transformation starting from the point A and coming back to the same point by tracing the path $A \to B \to C \to A$ as shown in the diagram above. The total work done in the process is _____ J. [2024]

Q 9 :

One mole of an ideal gas expands isothermally and reversibly from 10 ${dm}^{3}$ to 20 ${dm}^{3}$ at 300 K. $ΔU$ , $q$ and work done in the process respectively are:

Given: R = 8.3 J $K^{- 1}$ ${mol}^{- 1}$

ln 10 = 2.3
log 2 = 0.30
log 3 = 0.48 [2025]

Q 10 :

A liquid when kept inside a thermally insulated closed vessel at 25°C was mechanically stirred from outside. What will be the correct option for the following thermodynamic parameters? [2025]

Q 13 :

One mole of an ideal monoatomic gas is subjected to changes as shown in the graph. The magnitude of the work done (by the system or on the system) is ______ J (nearest integer) [2023]

Given: log 2 = 0.3
ln 10 = 2.3

Q 14 :

When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is _________ J. (Nearest Integer) [2023]

Q 15 :

1 mole of ideal gas is allowed to expand reversibly and adiabatically from a temperature of 27°C. The work done is 3 kJ ${mol}^{- 1}$ . The final temperature of the gas is ______ K (Nearest integer). Given $C_{v}$ = 20 J ${mol}^{- 1}$ $K^{- 1}$ . [2023]

Q 16 :

A cup of water at 5°C (system) is placed in a microwave oven and the oven is turned on for one minute during which the water begins to boil. Which of the following option is true? [2026]

Q 17 :

20.0 $d m ³$ of an ideal gas ‘X’ at 600 K and 0.5 MPa undergoes isothermal reversible expansion until pressure of the gas is 0.2 MPa. Which of the following option is correct?

(Given: log 2 = 0.3010 and log 5 = 0.6989) [2026]