A hollow sphere is rolling on a plane surface about its axis of symmetry. The ratio of rotational kinetic energy to its total kinetic energy is . The value of is _______ . [2024]
(2)
Hence,
A circular disc reaches from top to bottom of an inclined plane of length . When it slips down the plane, if takes s. When it rolls down the plane then it takes , where is _______ . [2024]
(3) If disc slips on inclined plane, then it's acceleration,
If disc rolls on inclined plane its acceleration,
Now
On comparing,
A solid sphere and a hollow cylinder roll up without slipping on the same inclined plane with the same initial speed v. The sphere and the cylinder reach up to maximum heights and , respectively, above the initial level. The ratio is . The value of n is _____. [2024]
(7)
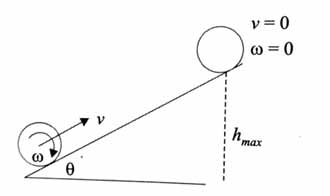
Energy conservation, Gain in P.E. = Loss in K.E.
For pure rolling;
Hence,
A solid sphere of mass 'm' and radius 'r' is allowed to roll without slipping from the highest point of an inclined plane of length 'L' and makes an angle 30° with the horizontal. The speed of the particle at the bottom of the plane is . If the angle of inclination is increased to 45° while keeping L constant. Then the new speed of the sphere at the bottom of the plane is . The ratio of is [2025]
1 : 3
1 : 2
(1)
Loss in P.E. = gain in K.E.
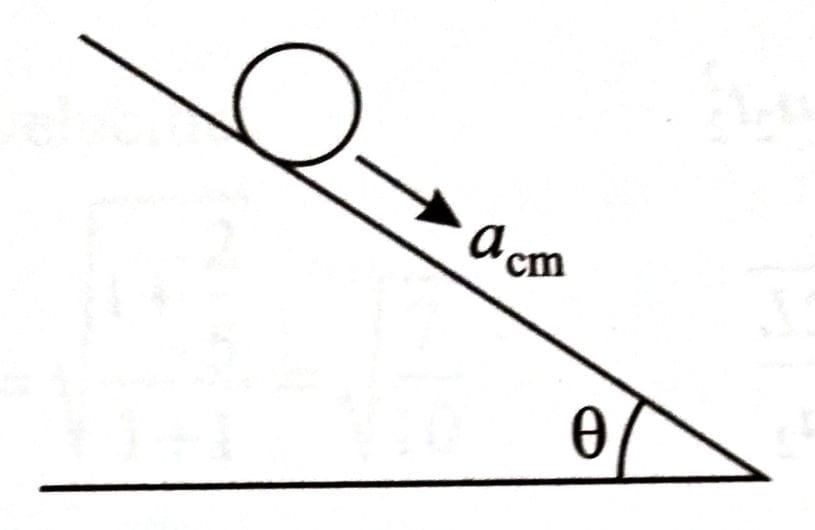
A uniform solid cylinder of mass 'm' and radius 'r' rolls along an inclined rough plane of inclination 45°. If it starts to roll from rest from the top of the plane then the linear acceleration of the cylinder axis will be: [2025]
(3)
A solid sphere and a hollow sphere of the same mass and of same radius are rolled on an inclined plane. Let the time taken to reach the bottom by the solid sphere and the hollow sphere be and , respectively, then [2025]
(1)
The time taken to reach the bottom,
Acceleration,
... Solid
... Hollow
As hence,
A force of 49 N acts tangentially at the highest point of a sphere (solid) of mass 20 kg, kept on a rough horizontal plane. If the sphere rolls without slipping, then the acceleration of the center of the sphere is [2025]

3.5
0.35
2.5
0.25
(1)
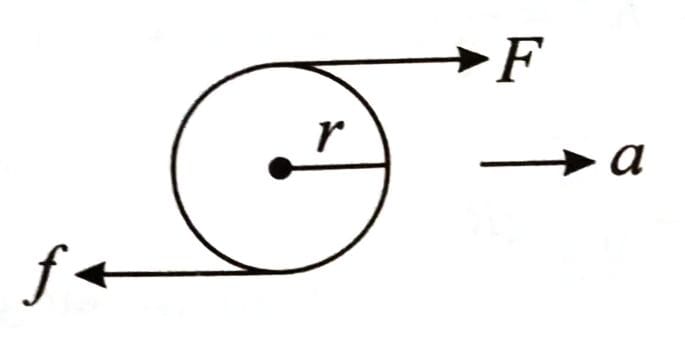
Torque about bottom point,
... (i)
As sphere rolls without slipping
... (ii)
A circular ring and a solid sphere having same radius roll down on an inclined plane from rest without slipping. The ratio of their velocities when reached at the bottom of the plane is where x = __________. [2025]
(4)
Applying Mechanical Energy conservation:
So, Ratio of velocities
x = 3.5 Rounding off x = 4.