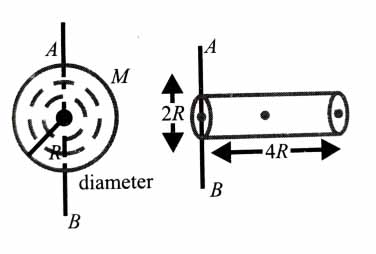
Ratio of radius of gyration of a hollow sphere to that of a solid cylinder of equal mass, for the moment of inertia about their diameter axis AB as shown in the figure, is . The value of is [2024].
17
34
67
51
(3)
Hollow Sphere,
Solid Cylinder,
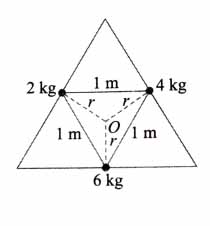
Three balls of masses 2 kg, 4 kg, and 6 kg respectively are arranged at the center of the edges of an equilateral triangle of side 2 m. The moment of inertia of the system about an axis through the centroid and perpendicular to the plane of the triangle will be ____ kg . [2024]
(4)
Four particles each of mass 1 kg are placed at four corners of a square of side 2 m. Moment of inertia of system about an axis perpendicular to its plane and passing through one of its vertex is _____ . [2024]
(16)

Two identical spheres each of mass 2 kg and radius 50 cm are fixed at the ends of a light rod so that the separation between the centers is 150 cm. Then, moment of inertia of the system about an axis perpendicular to the rod and passing through its middle point is , where the value of is _____. [2024]
(53)

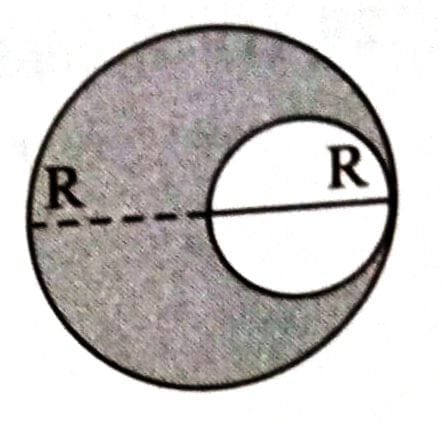
A uniform circular disc of radius 'R' and mass 'M' is rotating about an axis perpendicular to its plane and passing through its centre. A small circular part of radius R/2 is removed from the original disc as shown in the figure. Find the moment of inertia of the remaining part of the original disc about the axis as given above. [2025]

(4)
Moment of inertia of a rod of mass 'M' and length 'L' about an axis passing through its center and normal to its length is ''. Now the rod is cut into two equal parts and these parts are joined symmetrically to form a cross shape. Moment of inertia of cross about an axis passing through its center and normal to plane containing cross is: [2025]
(2)
Moment of inertia of the rod in case,

Moment of inertia of the rod in case,

The moment of inertia of a circular ring of mass M and diameter r about a tangential axis lying in the plane of the ring is: [2025]
(2)
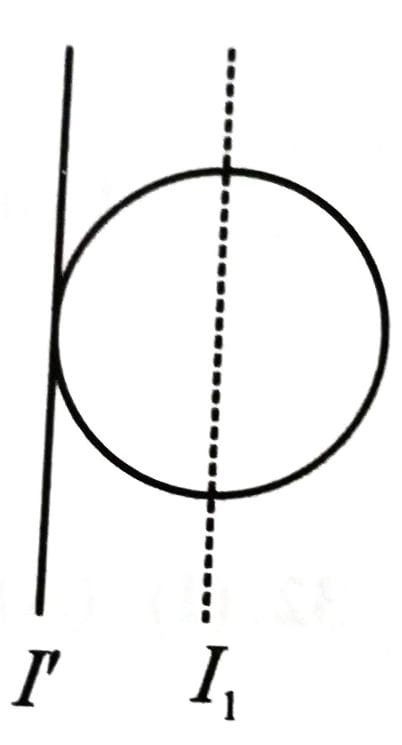
For r = 2R
A rod of linear mass density '' and length 'L' is bent to form a ring of radius 'R'. Moment of inertia of ring about any of its diameter is: [2025]
(4)
The moment of inertia of a solid disc rotating along its diameter is 2.5 times higher than the moment of inertia of a ring rotating in similar way. The moment of inertia of a solid sphere which has same radius as the disc and rotating in similar way, is n times higher than the moment of inertia of the given ring. Here, n = __________.
Consider all the bodies have equal masses. [2025]
(4)
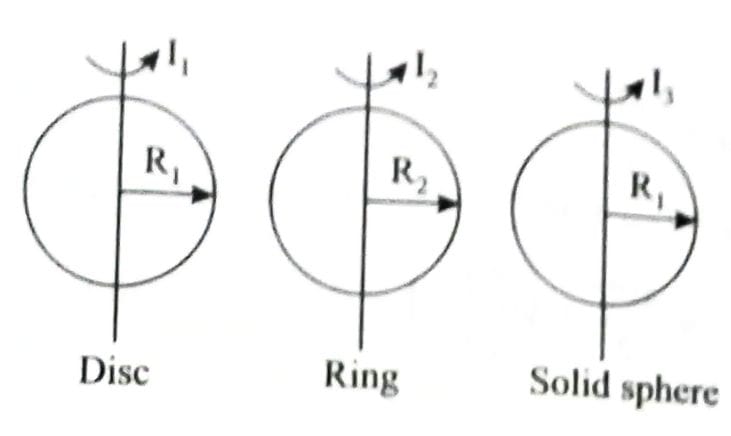
According to problem
... (i)
Now we are provided with information that
... (ii)
From Eq. (i) and (ii) n = 4
Two iron solid discs of negligible thickness have radii and and moment of inertia and , respectively. For , the ratio of and would be 1/x, where x = __________. [2025]
(16)
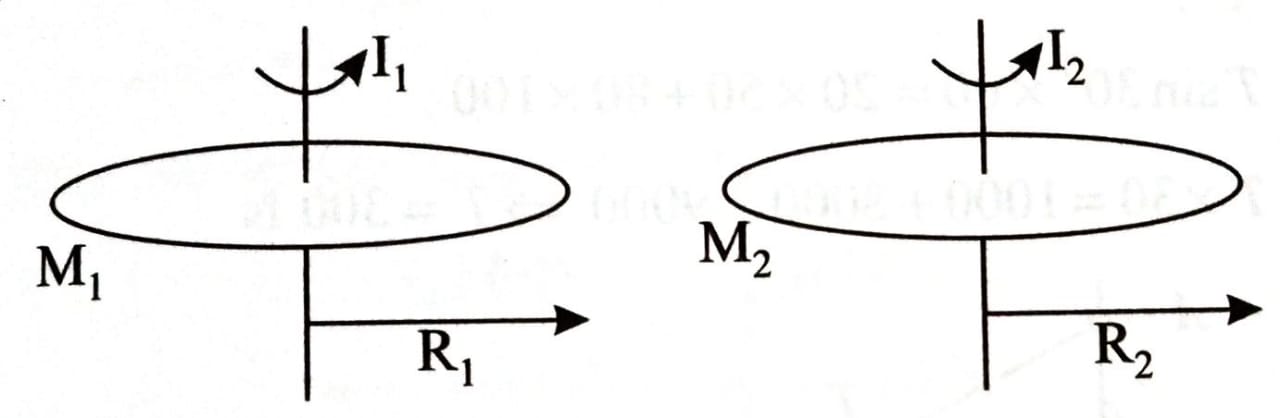
Given,