Topic Question Set
Q 1
:
Two planets A and B having masses and move around the sun in circular orbits of and radii respectively. If angular momentum of A is L and that of B is 3 L, the ratio of time period is [2024]
(1)
We know,
...(1)
Given
...(2)
Orbital velocity, ...(3)
From eq. (2) and eq. (3)
...(4)
Using eq. (4) in eq. (1)
Q 2
:
Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A): The angular speed of the moon in its orbit about the earth is more than the angular speed of the earth in its orbit about the sun.
Reason (R): The moon takes less time to move around the earth than the time taken by the earth to move around the sun.
In the light of the above statements, choose the most appropriate answer from the given below: [2024]
(A) is correct but (R) is not correct
Both (A) and (R) are correct and (R) is the correct explanation of (A)
Both (A) and (R) are correct but (R) is not the correct explanation of (A)
(A) is not correct but (R) is correct
(2)
27 days
365 days 4 hour
Q 3
:
A planet takes 200 days to complete one revolution around the Sun. If the distance of the planet from Sun is reduced to one fourth of the original distance, how many days will it take to complete one revolution [2024]
20
25
50
100
(2)
Q 4
:
A light planet is revolving around a massive star in a circular orbit of radius R with a period of revolution T. If the force of attraction between planet and star is proportional to then choose the correct option [2024]
(1)
or
Gravitational force provides centripetal force.
Q 5
:
Four identical particles of mass m are kept at the four corners of a square. If the gravitational force exerted on one of the masses by the other masses is the length of the sides of the square is [2024]
2 L
4 L
3 L
L/2
(2)

Q 6
:
A small point of mass m is placed at a distance 2R from the centre 'O' of a big uniform solid sphere of mass M and radius R. The gravitational force on 'm' due to M is . A spherical part of radius R/3 is removed from the big sphere as shown in the figure and the gravitational force on m due to remaining part of M is found to be . The value of ratio is [2025]
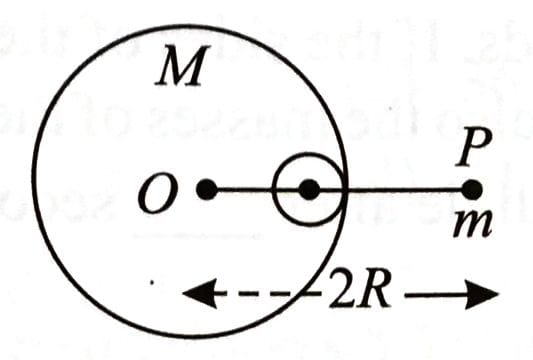

16 : 9
11 : 10
12 : 11
12 : 9
(3)
Q 7
:
If a satellite orbiting the earth is 9 times closer to the earth than the moon, what is the time period of rotation of the satellite? Given rotational time period of Moon = 27 days and gravitational attraction between the satellite and the moon is neglected. [2025]
1 day
81 days
27 days
3 days
(1)
From Kepler's law,
Q 8
:
A satellite is launched into a circular orbit of radius 'R' around the earth. A second satellite is launched into an orbit of radius 1.03 R. The time period of revolution of the second satellite is larger than the first one approximately by: [2025]
3%
4.5%
9%
2.5%
(2)
Q 9
:
Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : The radius vector from the Sun to a planet sweeps out equal areas in equal intervals of time and thus areal velocity of planet is constant.
Reason (R) : For a central force field the angular momentum is a constant.
In the light of the above statements, choose the most appropriate answer from the options given below: [2025]
Both (A) and (R) are correct and (R) is the correct explanation of (A)
Both (A) and (R) are correct but (R) is not the correct explanation of (A)
(A) is correct but (R) is not correct
(A) is not correct but (R) is correct
(1)
From Kepler's law
Due to central force torque is zero and angular momentum is constant.
Q 10
:
Two planets, A and B are orbiting a common star in circular orbits of radii and , respectively, with . The planet B is times more massive than planet A. The ratio of angular momentum () of planet B to that of planet A() is closest to integer __________. [2025]
(8)
Here M is mass of star

