Topic Question Set
Q 1
:
A 90 kg body placed at 2R distance from surface of earth experiences gravitational pull of (R = Radius of earth, ) [2024]
100 N
300 N
120 N
225 N
(1)
Acceleration due to gravity at height from earth's surface
Here gravitational acceleration at surface
Gravitational Pull
Q 2
:
Assuming the earth to be a sphere of uniform mass density, a body weighed 300 N on the surface of earth. How much it would weigh at R/4 depth under surface of earth? [2024]
300 N
75 N
225 N
375 N
(3)
At surface:
Gravitational field at depth under surface,
Weight at depth,
Q 3
:
The acceleration due to gravity on the surface of earth is g. If the diameter of earth reduces to half of its original value and mass remains constant, then acceleration due to gravity on the surface of earth would be [2024]
g/4
2g
g/2
4g
(4)
Q 4
:
If R is the radius of the earth and the acceleration due to gravity on the surface of earth is , then the length of the second's pendulum at a height from the surface of earth will be [2024]
(2)
We know,
Since the time period of second pendulum is 2 sec.
On solving,
Q 5
:
At what distance above and below the surface of the earth a body will have same weight? (take radius of earth as R). [2024]
(4)
Below the surface of the earth,
Above the surface of the earth,
If the body will have same weight,
Q 6
:
A simple pendulum is placed at a place where its distance from the earth's surface is equal to the radius of the earth. If the length of the string is 4m, then the time period of small oscillations will be ___________ s. [2024]
(8)
Q 7
:
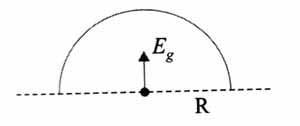
A metal wire of uniform mass density having length L and mass M is bent to form a semicircular arc, and a particle of mass m is placed at the centre of the arc. The gravitational force on the particle by the wire is _____. [2024]
0
-
-
-
(3)
Q 8
:
Acceleration due to gravity on the surface of earth is 'g'. If the diameter of earth is reduced to one third of its original value and mass remains unchanged, then the acceleration due to gravity on the surface of the earth is ________ g. [2025]
(9)
Acceleration due to gravity on surface,
Now since diameter is reduced to , radius also reduces to , keeping mass constant.
New value of acceleration due to gravity on Earth's surface,

