Topic Question Set
Q 1
:
Match List-I with List-II [2024]
List - I
List - II
A
Kinetic energy of planet
(I)
B
Gravitation Potential energy of sun planet system
(II)
C
Total mechanical energy of planet
(III)
D
Escape energy at the surface of planet for unit mass object
(IV)
(A)-(I), (B)-(IV), (C)-(II), (D)-(III)
(A)-(II), (B)-(I), (C)-(IV), (D)-(III)
(A)-(III), (B)-(IV), (C)-(I), (D)-(II)
(A)-(I), (B)-(II), (C)-(III), (D)-(IV)
(2)
Potential energy =
Total energy =
kinetic energy =
Escape energy =
Q 2
:
To project a body of mass m from earth's surface to infinity, the required kinetic energy is (assume, the radius of earth is ) g = acceleration due to gravity on the surface of earth: [2024]
(1)
Escape speed
Escape kinetic energy
Also,
Q 3
:
An astronaut takes a ball of mass m from earth to space. He throws the ball into a circular orbit about earth at an altitude of 318.5 km. From earth's surface to the orbit, the change in total mechanical energy of the ball is The value of is (take = 6370 km): [2024]
11
9
12
10
(1)
Change in total mechanical energy =
Q 4
:
The gravitational potential at a point above the surface of earth is J/kg and the acceleration due to gravity at that point is 6.4
Assume that the mean radius of earth to be 6400 km. The height of this point above the earth's surface is: [2024]
1600 km
540 km
1200 km
1000 km
(1)
6400 km + h = 8000 km
h = 1600 km
Q 5
:
Escape velocity of a body from earth is 11.2 km/s. If the radius of a planet be one-third the radius of earth and mass be one-sixth that of earth, the escape velocity from the planet is [2024]
11.2 km/s
8.4 km/s
4.2 km/s
7.9 km/s
(4)
for earth
for planet
km/sec
Q 6
:
The mass of the moon is 1/144 times the mass of a planet and its diameter 1/16 times the diameter of a planet. If the escape velocity on the planet is V, the escape velocity on the moon will be [2024]
(1)
Q 7
:
A satellite of kg mass is revolving in a circular orbit of radius 2R. If energy is supplied to the satellite, it would revolve in a new circular orbit of radius: (use g = 10 , R = radius of Earth) [2024]
2.5 R
3 R
4 R
6 R
(4)
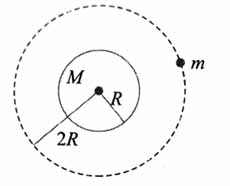
Q 8
:
Earth has mass 8 times and radius 2 times that of a planet. If the escape velocity from the earth is 11.2 km/s, the escape velocity in km/s from the planet will be : [2025]
11.2
5.6
2.8
8.4
(2)
Q 9
:
Given below are two statements, one is labelled as Assertion A and the other is labelled as Reason R
Assertion A : The kinetic energy needed to project a body of mass m from earth surface to infinity is , where R is the radius of earth.
Reason R : The maximum potential energy of a body is zero when it is projected to infinity from earth surface.
in the light of the above statements, choose the correct answer from the options given below [2025]
A is false but R is true
Both A and R are true and R is the correct explanation of A
A is true but R is false
Both A and R are true but R is NOT the correct explanation of A
(1)
At , PE is zero, U = 0
Q 10
:
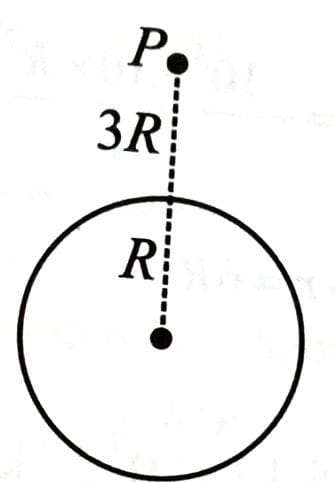
An object is kept at rest at a distance of 3R above the earth's surface where R is earth's radius. The minimum speed with which it must be projected so that it does not return to earth is: (Assume M = mass of earth, G = Universal gravitational constant) [2025]
(1)