If is the locus of a point, which moves such that it is always equidistant from the lines x + 2y + 7 = 0 and 2x – y + 8 = 0, then the value of g + c + h – f equals [2024]
29
6
8
14
(4)
Let point P(x, y) be equidistant from the given lines.
... (i)
This is the locus of the point P(x, y).
Now, compare equation (i) with given equation of locus, we get
,
,
,
and c = 5
.
Let A (a, b), B(3, 4) and C(–6, –8) respectively denote the centroid, circumcentre and orthocentre of a triangle. Then, the distance of the point P(2a + 3, 7b + 5) from the line 2x + 3y – 4 = 0 measured parallel to the line x – 2y – 1 = 0 is [2024]
(3)
Given the A (a, b), B(3, 4) and C(–6, –8) respectively the centroid, circumcentre and orthocentre of a triangle.
We know that centroid divides circumcentre and orthocentre internally in the ratio 1 : 2

a = 0, b = 0
Therefore, the coordinates of P are (3, 5).
Also, : 2x + 3y – 4 = 0 (Given) ... (i)
Equation of the line passing through P(3, 5) and parallel to the line x – 2y – 1 = 0 is
... (ii)
Solving equation (i) and (ii), we get
Distance between (3, 5) and is
Consider a triangle ABC having the vertices A(1, 2), and and angles and . If the points B and C lie on the line y = x + 4, then is equal to __________ [2024]
(14)
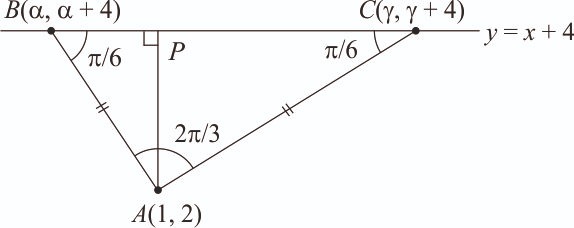
satisfy same equation
and are the roots of the equation
Sum of roots =
Product of roots =
Now,
.
Let a ray of light passing through the point (3, 10) reflects on the line 2x + y = 6 and the reflected ray passes through the point (7, 2). If the equation of the incident ray is ax + by + 1 = 0, then is equal to __________ . [2024]
(1)
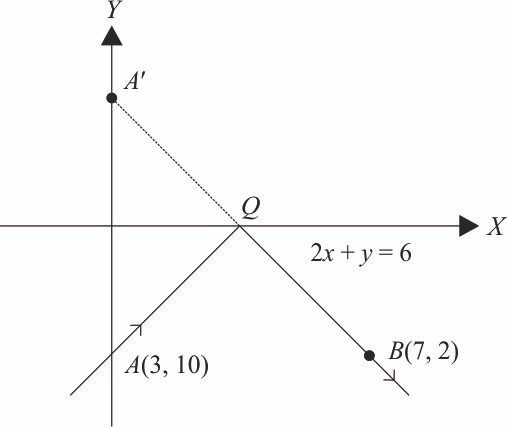
A'(–5, 6) and B(7, 2)
Equation of line A'B is
and 2x + y = 6 (Given line).
On solving, we get y = 4, x = 1
Equation of line AQ is
On comparing with given equation ax + by + 1 = 0, we get a = 3, b = –1
Hence, .
Let ABC be an isosceles triangle in which A is at (–1, 0), , AB = AC and B is on the positive x-axis. If and the line BC intersects the line y = x + 3 at , then is __________. [2024]
(36)

We have,
But B is on positive x-axis
Coordinates of B = (3, 0)
Now,
... (i)
Also,
... (ii)
Solving (i) and (ii), we get
So, coordinates of
Equation of line BC is,
... (iii)
Now, point of intersection of line (iii) and x + 3 = y is
.
If the sum of squares of all real values of , for which the lines 2x – y + 3 = 0, 6x + 3y + 1 = 0 and do not form a triangle is p, then the greatest integer less than or equal to p is __________. [2024]
(32)
We have, 2x – y + 3 = 0 ... (i)
6x + 3y + 1 = 0 ... (ii)
... (iii)
Case I. If the lines are concurrent then they do not form a triangle
Case II. If the lines are parallel then they do not form a triangle.
If the lines (i) and (iii) are parallel,
If the lines (ii) and (iii) are parallel,
Sum of squares of all real values of
.
Let for any three distinct consecutive terms a, b, c of an A.P., the lines ax + by + c = 0 be concurrent at the point P and be a point such that the system of equations x + y + z = 6, 2x + 5y + z = and x + 2y + 3z = 4, has infinitely many solutions. Then is equal to __________. [2024]
(113)
a, b, c are in A.P. 2b = a + c a – 2b + c = 0 and ax + by + c = 0 are concurrent.
Two lines and are concurrent if
So, P(1, –2) is the point of concurrency.
Now, x + y + z = 6 ... (i)
2x + 5y + z = ... (ii)
x + 2y + 3z = 4 ... (iii)
has infinitely many solution.
On solving these equation, we have
x + y = 6 – z, x + 2y = 4 – 3z
On solving these two, we get –y = 2 + 2z
y = –2(1 + z) x = 6 – z + 2 + 2z x = 8 + z
From (ii), we get 2(8 + z) + 5(–2(1 + z)) + z =
For infinitely many solution,
Let the area of the triangle formed by a straight line L : x + by + c = 0 with co-ordinate axes be 48 square units. If the perpendicular drawn from the origin to the line L makes an angle of 45° with the positive x-axis, then the value of is : [2025]
83
97
93
90
(2)
We have, area of OAB = 48 sq. units
Now, slope of line OD is m = tan 45° = 1
Slope of line ... (i)
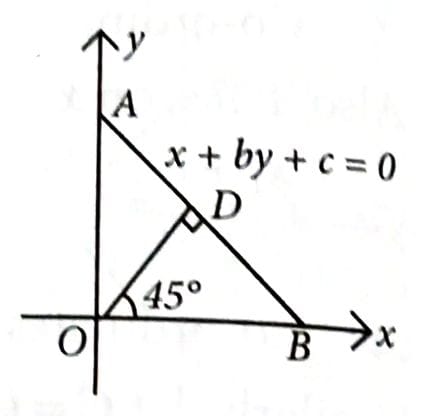
Also, equation of line AB is x + by + c = 0
So, Slope ... (ii)
From (i) and (ii), we get
Now, x and y intercept of line x + by + c = 0 are given as x = – c and respectively.
Area of OAB =
[ b = 1]
So, .
A line passes through the origin and makes equal angles with the positive coordinate axes. It intersects the lines and , p > 0, at the points A and B, respectively. If and the foot of the perpendicular from the point A on the line is M, then is equal to [2025]
4
3
5
2
(2)
We have, and
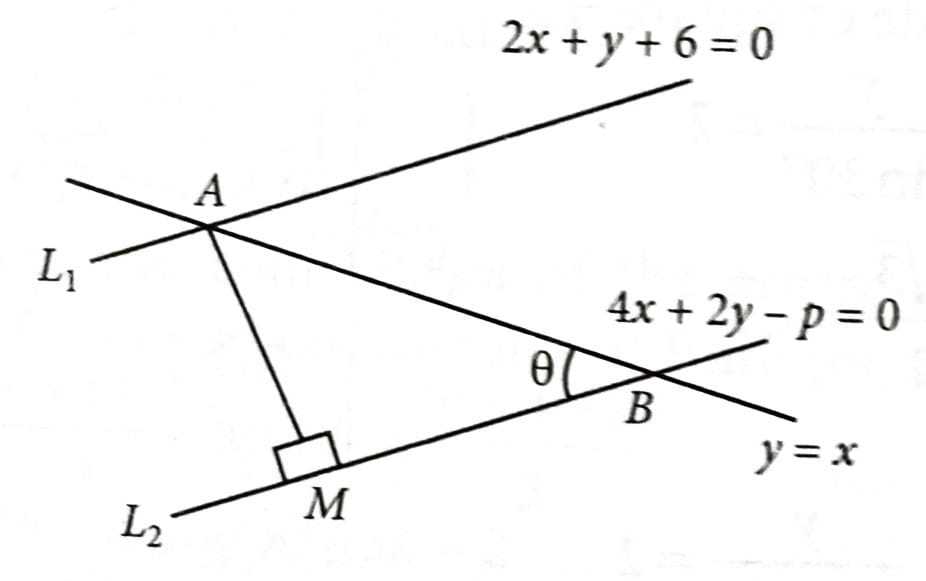
Line y = x passes through origin and makes equal angles with the positive coordinates axes.
Slope of y = x is , Slope of is
So,
Now, in ABM
.
The shortest distance between the curves and is: [2025]
(4)
Given, , which is a circle having centre, C = (0, –6)
Radius,
Since, the shortest distance between both curves will be normal to the curve from centre (0, –6), as shown in figure.
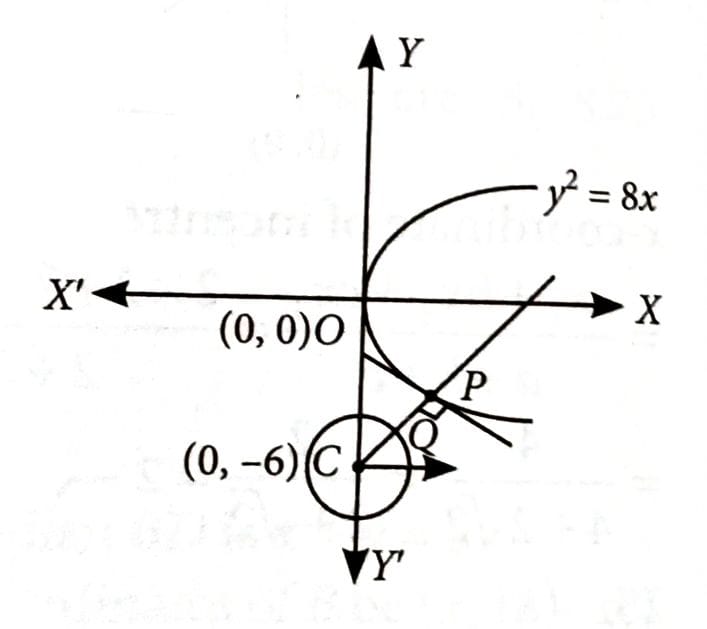
Equation of normal to the parabola
is
where m is slope, passes through (0, –6), then –6 = –4m –
Shortest distance, PQ = PC – r
.