Two Dimensional Geometry jee mains questions | JEE Main Two Dimensional Geometry Previous Year Question

Q 1 :

Let PQ be a chord of the parabola $y^{2} = 12 x$ and the midpoint of PQ be at (4, 1). Then, which of the following point lies on the line passing through the points P and Q? [2024]

(2, –9)
$(\frac{3}{2}, - 16)$
$(\frac{1}{2}, - 20)$
(3, –3)

1

2

3

4

(3)

$y^{2} = 12 x$

Chord PQ having mid-point $(x_{1}, y_{1}) \equiv (4, 1)$

Equation of chord PQ

$T = S_{1}$

$y y_{1} - 12 \frac{(x + x_{1})}{2} = y_{1}^{2} - 12 x_{1}$

$\Rightarrow y - 6 (x + 4) = 1 - 12 \times 4$

$\Rightarrow y - 6 x - 24 = - 47 \Rightarrow y - 6 x + 23 = 0$

From the options, point $(\frac{1}{2}, - 20)$ lies on the chord.

Q 2 :

Let C be the circle of minimum area touching the parabola $y = 6 - x^{2}$ and the lines $y = \sqrt{3} | x |$ . Then, which one of the following points lies on the circle C? [2024]

(1, 1)
(2, 4)
(2, 2)
(1, 2)

1

2

3

4

(*)

The given data is inadequate.

Q 3 :

If the shortest distance of the parabola $y^{2} = 4 x$ from the centre of the circle $x^{2} + y^{2} - 4 x - 16 y + 64 = 0$ is d, then $d^{2}$ is equal to : [2024]

16
24
20
36

1

2

3

4

(3)

Circle $x^{2} + y^{2} - 4 x - 16 y + 64 = 0$

Centre $\equiv$ (2, 8), Radius = 2 units

Parabola : $y^{2} = 4 x$

a = 1

Equation of normal at $(t^{2}, 2 t)$ of parabola:

$x t + y = a t^{3} + 2 a t$

$x t + y = t^{3} + 2 t$ ... (i)

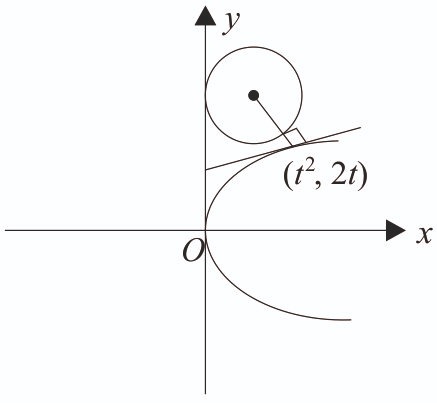
Since, normal passes through centre, then

$2 t + 8 = t^{3} + 2 t$ ... (ii)

$\Rightarrow t^{3} = 8$

$\Rightarrow t^{3} - 8 = 0$

$\Rightarrow (t - 2) (t^{2} + 4 + 2 t) = 0$

$\Rightarrow t = 2 (∵ t \geq 0)$

d = distance between (2, 8) and (4, 4) = $\sqrt{4 + 16} = \sqrt{20}$

$\Rightarrow d^{2} = 20$ .

Q 4 :

Let the length of the focal chord PQ of the parabola $y^{2} = 12 x$ be 15 units. If the distance of PQ from the origin is p, then $10 p^{2}$ is equal to __________. [2024]

(72)

$P Q = 15 \Rightarrow (3 {(t^{2} - \frac{1}{t^{2}}))}^{2} + (6 {(t + \frac{1}{t}))}^{2} = 225$

$\Rightarrow 9 {(t^{2} - \frac{1}{t^{2}})}^{2} + 36 {(t + \frac{1}{t})}^{2} = 225$

$\Rightarrow {(t + \frac{1}{t})}^{2} [{(t - \frac{1}{t})}^{2} + 4] = 25$

$\Rightarrow {(t + \frac{1}{t})}^{2} {(t + \frac{1}{t})}^{2} = 25 \Rightarrow {(t + \frac{1}{t})}^{4} = 25$

$\Rightarrow t + \frac{1}{t} = \pm \sqrt{5} \Rightarrow (t - \frac{1}{t}) = \pm 1$

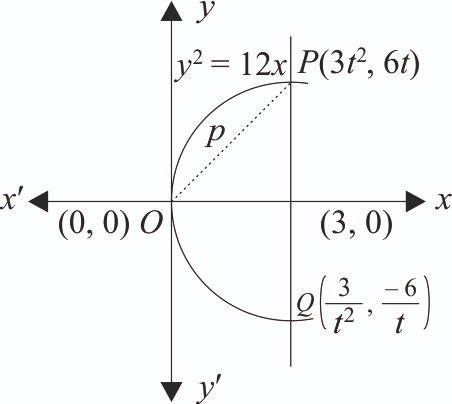
$\Rightarrow$ Equation of PQ : $(y - 6 t) = (\frac{2 t}{t^{2} - 1}) (x - 3 t^{2})$

$\Rightarrow$ Distance from y – 6t = mx – $3 m t^{2}$ , where $m = \frac{2 t}{t^{2} - 1}$

$\Rightarrow p = \frac{| 3 m t^{2} - 6 t |}{\sqrt{1 + m^{2}}} = \frac{| (\frac{6 t}{t^{2} - 1}) |}{\sqrt{5}} = \frac{6}{\sqrt{5}}$

$\Rightarrow 10 p^{2} = \frac{10 \times 36}{5} = 72$ .

Q 5 :

Suppose AB is a focal chord of the parabola $y^{2} = 12 x$ of length $l$ and slope $m < \sqrt{3}$ . If the distance of the chord AB from the origin is d, then $l d^{2}$ is equal to __________. [2024]

(108)

Equation of focal chord

$y - 0 = \tan θ \cdot (x - 3)$

$\Rightarrow \tan θ \cdot x - y - 3 \tan θ = 0$

$∴$ Distance from origin,

$d =$ $| \frac{- 3 \tan θ}{\sqrt{1 + \tan^{2} θ}} |$

$l$ $= 4 \times 3 {cosec}^{2} θ$

$l$ $\cdot d^{2} = \frac{9 \tan^{2} θ}{1 + \tan^{2} θ} \times 12 {cosec}^{2} θ$

$= \frac{108 {cosec}^{2} θ}{1 + \cot^{2} θ} = 108$

Q 6 :

Let a line perpendicular to the line 2x – y = 10 touch the parabola $y^{2} = 4 (x - 9)$ at the point P. The distance of the point P from the centre of the circle $x^{2} + y^{2} - 14 x - 8 y + 56 = 0$ is __________. [2024]

(10)

Let L : 2x – y = 10 and $C : y^{2} = 4 (x - 9)$

Equation of line perpendicular to L is given by

2y + x = k

Now, let us find the point of intersection of 2y + x = k and $y^{2} = 4 (x - 9)$

$i . e ., {(\frac{k - x}{2})}^{2} = 4 (x - 9)$

$\Rightarrow x^{2} - 2 (k + 8) x + (k^{2} + 144) = 0$

As parabola touches the line so this quadratic equation must have at most one real root

$\Rightarrow D = 0$

$\Rightarrow 4 {(k + 8)}^{2} - 4 (k^{2} + 144) = 0$

$\Rightarrow 16 k + 64 - 144 = 0$

$\Rightarrow k = 5$

So, equation becomes $x^{2} - 26 x + 169 = 0$

$\Rightarrow {(x - 13)}^{2} = 0$

$\Rightarrow x = 13$

$\Rightarrow y = \pm 4$

Now, parabola and line 2y + x = 5 meets at P(13, –4)

Now, centre of given circle is (7, 4)

$∴$ Required distance $= \sqrt{{(13 - 7)}^{2} + {(- 4 - 4)}^{2}} = 10$ .

Q 7 :

Let $L_{1}$ , $L_{2}$ be the lines passing through the point P(0, 1) and touching the parabola $9 x^{2} + 12 x + 18 y - 14 = 0$ . Let Q and R be the points on the lines $L_{1}$ and $L_{2}$ such that the $△ P Q R$ is an isosceles triangle with base QR. If the slopes of the lines QR are $m_{1}$ and $m_{2}$ , then $16 (m_{1}^{2} + m_{2}^{2})$ is equal to __________. [2024]

(68)

We have $9 x^{2} + 12 x + 18 y - 14 = 0$

$\Rightarrow 9 x^{2} + 12 x + 4 + 18 y - 18 = 0$

$\Rightarrow {(3 x + 2)}^{2} = - 18 y + 18$

$\Rightarrow {(x + \frac{2}{3})}^{2} = - 2 (y - 1)$

Vertex of parabola is $(\frac{- 2}{3}, 1)$

Now, equation of line passing through (0, 1) is given by y = mx + 1.

Since the line is touching the parabola, so we have

${(x + \frac{2}{3})}^{2} = - 2 m x$

$\Rightarrow {(3 x + 2)}^{2} = - 18 m x$

$\Rightarrow 9 x^{2} + (12 + 18 m) x + 4 = 0$

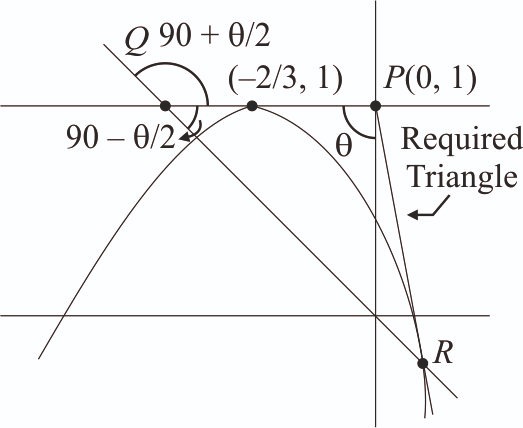
Discriminant of this quadratic equation must be zero.

$\Rightarrow 4 {(6 + 9 m)}^{2} = 4 (36)$

$\Rightarrow 6 + 9 m = 6 or - 6$

$\Rightarrow m = 0, \frac{- 4}{3}$

$∴ \tan θ = \frac{- 4}{3}$

$\Rightarrow \frac{2 \tan θ / 2}{1 - \tan^{2} θ / 2} = \frac{- 4}{3}$

$\Rightarrow - 4 + 4 \tan^{2} θ / 2 - 6 \tan θ / 2 = 0$

$\Rightarrow 2 \tan^{2} θ / 2 - 3 \tan θ / 2 - 2 = 0$

$\Rightarrow (\tan \frac{θ}{2} - 2) (2 \tan \frac{θ}{2} + 1) = 0$

$\Rightarrow \tan \frac{θ}{2} = 2, \frac{- 1}{2}$ ... (i)

Now, in $△ P Q R, ∠ P = θ s o ∠ Q = ∠ R = 90 - \frac{θ}{2}$

$∴ m_{Q R} = \tan (90 + \frac{θ}{2}) = - c o t \frac{θ}{2} = \frac{- 1}{2}, 2$ [Using (i)]

$\Rightarrow m_{1} = \frac{- 1}{2}, m_{2} = 2$

$∴ 16 (m_{1}^{2} + m_{2}^{2}) = 16 (\frac{1}{4} + 4) = 68$ .

Q 8 :

Let a conic C pass through the point (4, –2) and P(x, y), $x \geq 3$ , be any point on C. Let the slope of the line touching the conic C only at a single point P be half the slope of the line joining the points P and (3, –5). If the focal distance of the point (7, 1) on C is d, then 12d equals __________. [2024]

(75)

Slope of C at P $= \frac{1}{2} (\frac{y + 5}{x - 3})$

$\Rightarrow \frac{d y}{d x} = \frac{1}{2} (\frac{y + 5}{x - 3}) \Rightarrow \frac{2 d y}{y + 5} = \frac{1}{x - 3} d x$

On intergrating, we get

2 ln (y + 5) = ln (x – 3) + C

Since, C passes through (4, –2)

$\Rightarrow 2 \ln 3 = C$

$\Rightarrow 2 \ln (y + 5) = \ln (x - 3) + 2 \ln 3$

$\Rightarrow 2 \ln (\frac{y + 5}{3}) = \ln (x - 3) \Rightarrow {(\frac{y + 5}{3})}^{2} = x - 3$

$\Rightarrow {(y + 5)}^{2} = 9 (x - 3)$ , which represent a parabola so, 4a = 9

$\Rightarrow a = \frac{9}{4}$

Focus $= (3 + \frac{9}{4}, - 5) = (\frac{21}{4}, - 5)$

$∴ d = \sqrt{{(\frac{21}{4} - 7)}^{2} + {(- 5 - 1)}^{2}}$

$= \sqrt{{(- \frac{7}{4})}^{2} + {(- 6)}^{2}} = \frac{25}{4}$

$\Rightarrow 12 d = \frac{25}{4} \times 12 = 75$ .

Q 9 :

Let A, B and C be three points on the parabola $y^{2} = 6 x$ and let the line segment AB meet the line L through C parallel to the x-axis at the point D. Let M and N respectively be the feet of the perpendiculars from A and B on L. then ${(\frac{A M \cdot B N}{C D})}^{2}$ is equal to __________. [2024]

(36)

Equation of parabola, $y^{2} = 6 x$

$i . e ., y^{2} = 4 \times \frac{3}{2} x$

Let $A (\frac{3}{2} t_{1}^{2}, 3 t_{1}), B (\frac{3}{2} t_{2}^{2}, 3 t_{2}) and C (\frac{3}{2} t_{3}^{2}, 3 t_{3})$

be points on parabola $y^{2} = 6 x$ .

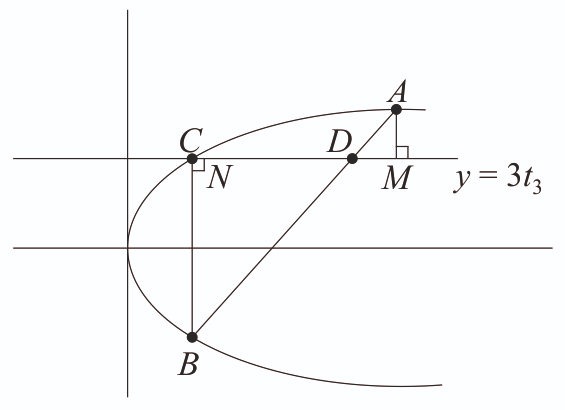
Equation of AB is given by

$\Rightarrow (y - 3 t_{1}) = \frac{3 t_{2} - 3 t_{1}}{\frac{3}{2} (t_{2}^{2} - t_{1}^{2})} (x - \frac{3}{2} t_{1}^{2})$

$\Rightarrow y - 3 t_{1} = \frac{2}{t_{1} + t_{2}} \frac{(2 x - 3 t_{1}^{2})}{2}$

$\Rightarrow (y - 3 t_{1}) (t_{1} + t_{2}) = 2 x - 3 t_{1}^{2}$

$\Rightarrow y (t_{1} + t_{2}) - 3 t_{1}^{2} - 3 t_{1} t_{2} = 2 x - 3 t_{1}^{2}$

$\Rightarrow y (t_{1} + t_{2}) = 2 x + 3 t_{1} t_{2}$

For point D, $y = 3 t_{3}$

$\Rightarrow 2 x = 3 t_{1} t_{3} + 3 t_{2} t_{3} - 3 t_{1} t_{2}$

$\Rightarrow x = \frac{3}{2} (t_{1} t_{3} + t_{2} t_{3} - t_{1} t_{2})$

$| C D | = \frac{3}{2} (t_{1} t_{3} + t_{2} t_{3} - t_{1} t_{2}) - \frac{3}{2} t_{3}^{2}$

$| A M | = | 3 t_{1} - 3 t_{3} |, | B N | = | 3 t_{2} - 3 t_{3} |$

So, ${(\frac{A M \cdot B N}{C D})}^{2} = {(\frac{9 (t_{1} - t_{3}) (t_{2} - t_{3})}{\frac{3}{2} (t_{1} - t_{3}) (t_{3} - t_{2})})}^{2} = {(- 6)}^{2} = 36$ .

Q 10 :

Consider the circle $C : x^{2} + y^{2} = 4$ and the parabola $P : y^{2} = 8 x$ . If the set of all values of $α$ , for which three chords of the circle C on three distinct lines passing through the point $(α, 0)$ are bisected by the parabola P is the interval (p, q), then ${(2 q - p)}^{2}$ is equal to __________. [2024]

(80)

Equation of chord of parabola whose mid-point is $(α, 0)$ is given by $T = S_{1}$

$\Rightarrow y y_{1} - 4 (x + x_{1}) = y_{1}^{2} - 8 x_{1}$

$i . e ., - 4 (x + α) = 0 - 8 α$

$\Rightarrow x = α$

Equation of chord of circle with $A (2 t^{2}, 4 t)$ as mid-point is

$x x_{1} + y y_{1} - 4 = x_{1}^{2} + y_{1}^{2} - 4$

$\Rightarrow 2 t^{2} x + 4 t y = 4 t^{4} + 16 t^{2}$

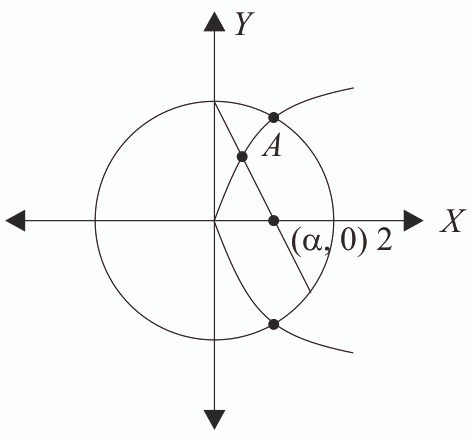
Also, it passes through $(α, 0)$

$\Rightarrow 2 t^{2} α = 4 t^{4} + 16 t^{2}$

$\Rightarrow 2 α = 4 t^{2} + 16$

$\Rightarrow α = 2 t^{2} + 8 = x_{0} + 8$

Also, we have $x^{2} + y^{2} = 4$ and $y^{2} = 8 x$

$\Rightarrow x^{2} + 8 x - 4 = 0$

$\Rightarrow x_{0} = \frac{- 8 + \sqrt{80}}{2}$

$\Rightarrow p = 8 and q = 4 + 2 \sqrt{5}$

$∴ {(2 q - p)}^{2} = 80$ .

Q 11 :

Let the line $L : \sqrt{2} x + y = α$ pass through the point of the intersection P (in the first quadrant) of the circle $x^{2} + y^{2} = 3$ and the parabola $x^{2} = 2 y$ . Let the line L touch two circles $C_{1}$ and $C_{2}$ of equal radius $2 \sqrt{3}$ . If the centres $Q_{1}$ and $Q_{2}$ of the circle $C_{1}$ and $C_{2}$ lie on the y-axis, then the square of the areas of the triangle $P Q_{1} Q_{2}$ is equal to __________. [2024]

(72)

We have, $L : \sqrt{2} x + y = α$

$x^{2} + y^{2} = 3$ ... (i)

$x^{2} = 2 y$ ... (ii)

Equation (i) and (ii) intersect at $(\sqrt{2}, 1)$ in first quadrant.

$∴ P = (\sqrt{2}, 1) and α = \sqrt{2} \times \sqrt{2} + 1 = 3$

$\Rightarrow α = 3$

Radius of Circle $C_{1}$ and $C_{2} = 2 \sqrt{3}$

We centre $C_{1}$ & $C_{2}$ of two circle are $(0, y_{1})$ and $(0, y_{2})$ respectively.

We know that length of perpendicular from centre to the tangent = Radius of circle

$∴$ $| \frac{\sqrt{2} \times 0 + y - 3}{\sqrt{3}} |$ $= 2 \sqrt{3}$

$\Rightarrow | y - 3 | = 6 \Rightarrow y = 9, - 3$

$∴ y_{1} = - 3, y_{2} = 9$

$∴$ Centre of $C_{1}$ and $C_{2}$ are $Q_{1} (0, - 3)$ and $Q_{2} (0, 9)$ respectively.

$Q_{1} Q_{2} = 12$ units, Height of triangle $= \sqrt{2}$ units

A = Area of triangle $P Q_{1} Q_{2} = \frac{1}{2} \times 12 \times \sqrt{2}$

$[∵ Area of △ P Q_{1} Q_{2} = \frac{1}{2} \times Q_{1} Q_{2} \times height]$

$= 6 \sqrt{2} sq . units$

$\Rightarrow A^{2} = 72$ .

Q 12 :

Let $P (α, β)$ be a point on the parabola $y^{2} = 4 x$ . If P also lies on the chord of the parabola $x^{2} = 8 y$ whose mid point is $(1, \frac{5}{4})$ , then $(α - 28) (β - 8)$ is equal to __________. [2024]

(192)

We $α = t^{2}, β = 2 t$

Now, equation of chord of the parabola $x^{2} = 8 y$ , bisected at $(1, \frac{5}{4})$ is $x \cdot 1 - 2 \times 2 (y + \frac{5}{4}) = 1 - 10$

$\Rightarrow x - 4 y - 5 = - 9 \Rightarrow x - 4 y + 4 = 0$

Now, $(α, β)$ satisfies the above equation

$∴ t^{2} - 8 t + 4 = 0$ ... (i)

Now, $(α - 28) (β - 8) = (t^{2} - 28) (2 t - 8)$

$= (8 t - 4 - 28) (2 t - 8)$ (Using (i))

$= 16 t^{2} - 64 t - 64 t + 256 = 16 (t^{2} - 8 t + 16)$

$= 16 (t^{2} - 8 t + 4 + 12) = 192$ .

Q 13 :

Let the focal chord PQ of the parabola $y^{2} = 4 x$ make an angle of 60° with the positive x-axis, where P lies in the first quadrant. If the circle, whose one diameter is PS, S being the focus of the parabola, touches the y-axis at the point (0, $α$ ), then $5 α^{2}$ is equal to : [2025]

15
25
20
30

1

2

3

4

(1)

Let the coordinate of a point P be $(t^{2}, 2 t)$

Coordinate of focus S of the parabola is (1, 0).

Now, $\tan 60 ° = \frac{2 t - 0}{t^{2} - 1} = \sqrt{3} \Rightarrow \sqrt{3} t^{2} - 2 t - \sqrt{3} = 0$

$\Rightarrow (\sqrt{3} t + 1) (t - \sqrt{3}) = 0 \Rightarrow t = \sqrt{3}$ { $∵$ P lies in first quadreant}

$∴ P (3, 2 \sqrt{3})$

Equation of circle is $(x - 1) (x - 3) + (y - 0) (y - 2 \sqrt{3}) = 0$

At x = 0, $3 + y^{2} - 2 \sqrt{3} y = 0$

$\Rightarrow {(y - \sqrt{3})}^{2} = 0 \Rightarrow y = \sqrt{3}$

$\Rightarrow y = \sqrt{3} = α$

$∴ 5 α^{2} = 15$ .

Q 14 :

Let the point P of the focal chord PQ of the parabola $y^{2} = 16 x$ be (1, –4). If the focus of the parabola divides the chord PQ in the ratio m : n, gcd (m, n) = 1, then $m^{2} + n^{2}$ is equal to : [2025]

10
17
26
37

1

2

3

4

(2)

End point of the focal chord PQ of the parabola $y^{2} = 4 a x$ is $P (a t^{2}, 2 a t)$

Now, we have parabola $y^{2} = 16 x$

$∴ P (4 t^{2}, 8 t) = (1, - 4)$

$\Rightarrow t = \frac{- 1}{2}$

$∴$ Point Q is given by $(\frac{a}{t^{2}}, - \frac{2 a}{t}) = (16, 16)$

Now, focus of parabola $y^{2} = 16 x$ is (4, 0)

Focus (4, 0) divides P(1, –4) and Q(16, 16) in ratio m : n

$\Rightarrow (4, 0) = (\frac{16 m + n}{m + n}, \frac{16 m - 4 n}{m + n})$

$\Rightarrow 16 m - 4 n = 0 \Rightarrow 4 m = n \Rightarrow \frac{m}{n} = \frac{1}{4}$

$\Rightarrow m = 1, n = 4$ [ $∵$ gcd(m, n) = 1]

$∴ m^{2} + n^{2} = 1 + 16 = 17$ .

Q 15 :

The radius of the smallest circle which touches the parabolas $y = x^{2} + 2$ and $x = y^{2} + 2$ is [2025]

$\frac{7 \sqrt{2}}{2}$
$\frac{7 \sqrt{2}}{16}$
$\frac{7 \sqrt{2}}{4}$
$\frac{7 \sqrt{2}}{8}$

1

2

3

4

(4)

The given parabolas are symmetric about the line y = x.

Tangents at A and B must be parallel to line y = x, so slope of the tangents = 1, which is minimum.

${(\frac{d y}{d x})}_{\min A} = 1 = {(\frac{d y}{d x})}_{\min B}$

For point B, $y = x^{2} + 2$

$\Rightarrow \frac{d y}{d x} = 2 x = 1$

When $x = \frac{1}{2} \Rightarrow y = \frac{9}{4}$

$∴ Point B = (\frac{1}{2}, \frac{9}{4})$

Similarly, point $A = (\frac{9}{4}, \frac{1}{2})$

$A B = \sqrt{{(\frac{1}{2} - \frac{9}{4})}^{2} + {(\frac{9}{4} - \frac{1}{2})}^{2}} = \sqrt{\frac{98}{16}} = \frac{7 \sqrt{2}}{4}$

Radius = $\frac{\frac{7 \sqrt{2}}{4}}{2} = \frac{7 \sqrt{2}}{8}$ .

Q 16 :

The axis of a parabola is the line y = x and its vertex and focus are in the first quadrant at distances $\sqrt{2}$ and $2 \sqrt{2}$ units from the origin, respectively. If the point (1, k) lies on the parabola, then a possible value of k is : [2025]

8
4
3
9

1

2

3

4

(4)

For the parabola, axis is y = x

$\Rightarrow$ Directrix is x + y = 0

Also, vertex and focus are of $\sqrt{2}$ and $2 \sqrt{2}$ from origin

$\Rightarrow$ Vertex is (1, 1) and focus = (2, 2)

The point P(1, k) lies on parabola.

Using definition of parabola

PS = PM

$\Rightarrow \sqrt{{(1 - 2)}^{2} + {(k - 2)}^{2}} = \frac{1 + k}{\sqrt{2}}$

$\Rightarrow k^{2} - 10 k + 9 = 0$

$\Rightarrow (k - 9) (k - 1) = 0$

$∴$ k = 9 and k = 1.

Q 17 :

Let P be the parabola, whose focus is (–2, 1) and directrix is 2x + y + 2 = 0. Then the sum of the ordinates of the points on P, whose abscissa is –2, is [2025]

$\frac{5}{2}$
$\frac{3}{2}$
$\frac{3}{4}$
$\frac{1}{4}$

1

2

3

4

(2)

Equation of parabola.

${(x + 2)}^{2} + {(y - 1)}^{2} = {(\frac{2 x + y + 2}{\sqrt{5}})}^{2}$

$\Rightarrow 5 [{(x + 2)}^{2} + {(y - 1)}^{2}] = {(2 x + y + 2)}^{2}$ ... (i)

Put x = –2, in equation (i),

$5 {(y - 1)}^{2} = {(y - 2)}^{2}$

$\Rightarrow 5 (y^{2} - 2 y + 1) = y^{2} - 4 y + 4$

$\Rightarrow 4 y^{2} - 6 y + 1 = 0$

$∴$ The sum of ordinates, $y_{1} + y_{2} = \frac{3}{2}$ .

Q 18 :

Let the parabola $y = x^{2} + p x - 3$ , meet the coordinate axes at the points P, Q and R. If the circle C with centre at (–1, –1) passes through the points P, Q and R, then the area of $△$ PQR is : [2025]

7
4
6
5

1

2

3

4

(3)

Given, equation of parabola is $y = x^{2} + p x - 3$ and equation of circle with centre at (–1, –1) is

${(x + 1)}^{2} + {(y + 1)}^{2} = r^{2}$ ... (i)

Let P( $α$ , 0), Q( $β$ , 0) and R(0, –3) are the three points.

Now, circle passes through point R(0, –3)

$\Rightarrow 1 + {(- 2)}^{2} = r^{2} \Rightarrow 5 = r^{2}$

Put y = 0 in equation (i), we get

${(x + 1)}^{2} + 1 = 5$

$\Rightarrow {(x + 1)}^{2} = 4 \Rightarrow x = 1 o r x = - 3$

$\Rightarrow$ Point are P(1, 0) and (–3, 0)

$∴ Area of △ P Q R = \frac{1}{2}$ $| \begin{matrix} 1 & 0 & 1 \\ - 3 & 0 & 1 \\ 0 & - 3 & 1 \end{matrix} |$ $= 6 sq . units$

Q 19 :

Let $P (4, 4 \sqrt{3})$ be a point on the parabola $y^{2} = 4 a x$ and PQ be a focal chord of the parabola. If M and N are the foot of perpendiculars drawn from P and Q respectively on the directrix of the parabola, then the area of the quadrilateral PQMN is equal to : [2025]

$\frac{34 \sqrt{3}}{3}$
$\frac{343 \sqrt{3}}{8}$
$\frac{263 \sqrt{3}}{8}$
$17 \sqrt{3}$

1

2

3

4

(2)

Since, $P (4, 4 \sqrt{3})$ lies on $y^{2} = 4 a x$

$\Rightarrow 48 = 4 a (4) \Rightarrow 4 a = 12$

$\Rightarrow y^{2} = 12 x$ is equation of parabola

Let $(4, 4 \sqrt{3})$ be $(a t^{2}, 2 a t) \Rightarrow t = \frac{2}{\sqrt{3}}$

As PQ is focal chord

$∴ Q (\frac{a}{t^{2}}, \frac{- 2 a}{t}) = (\frac{9}{4}, - 3 \sqrt{3})$

Area of trapezium PQNM

$= \frac{1}{2} M N (P M + Q N)$

$= \frac{1}{2} M N (P S + Q S)$ [by definition of parabola]

$= \frac{1}{2} \times M N \times P Q$

$= \frac{1}{2} \times 7 \sqrt{3} (\frac{49}{4}) = (343) \frac{\sqrt{3}}{8}$ .

Q 20 :

If the line 3x – 2y + 12 = 0 intersects the parabola $4 y = 3 x^{2}$ at the points A and B, then at the vertex of the parabola, the line segment AB subtends an angle equal to [2025]

$\tan^{- 1} (\frac{11}{9})$
$\tan^{- 1} (\frac{9}{7})$
$\tan^{- 1} (\frac{4}{5})$
$\frac{π}{2} - \tan^{- 1} (\frac{3}{2})$

1

2

3

4

(2)

Given, 3x – 2y + 12 = 0

$\Rightarrow y = \frac{3 x + 12}{2}$

Put value of 'y' in $4 y = 3 x^{2}$ , we get

$\Rightarrow 2 (3 x + 12) = 3 x^{2}$

$\Rightarrow x^{2} - 2 x - 8 = 0 \Rightarrow x = - 2, 4$

$∴$ y = 3, 12

Let A(–2, 3) and B(4, 12)

Since, vertex of parabola is O(0, 0).

$∴ m_{O A} = \frac{- 3}{2}, m_{O B} = \frac{- 12}{- 4} = 3$

$∴ \tan θ =$ $| \frac{m_{O A} - m_{O B}}{1 + m_{O A} \times m_{O B}} |$ $=$ $| \frac{\frac{- 3}{2} - 3}{1 + (\frac{- 3}{2}) \times 3} |$

$\Rightarrow \tan θ = \frac{9}{7} \Rightarrow θ = \tan^{- 1} (\frac{9}{7})$ .

Q 21 :

Let the shortest distance from (a, 0), a > 0, to the parabola $y^{2} = 4 x$ be 4. Then the equation of the circle passing through the point (a, 0) and the focus of the parabola, and having its centre on the axis of the parabola is: [2025]

$x^{2} + y^{2} - 4 x + 3 = 0$
$x^{2} + y^{2} - 8 x + 7 = 0$
$x^{2} + y^{2} - 10 x + 9 = 0$
$x^{2} + y^{2} - 6 x + 5 = 0$

1

2

3

4

(4)

Equation of normal at $P (t^{2}, 2 t)$ is given by

$y + t x = 2 t + t^{3}$ ... (i)

Put x = a, y = 0 in equation (i), we get

$a t = 2 t + t^{3}$

$\Rightarrow a = 2 + t^{2}$

$∴$ The point Q is $(2 + t^{2}, 0)$

$\Rightarrow P Q = 4 \Rightarrow 4 + 4 t^{2} = 16$

$\Rightarrow 4 t^{2} = 12 \Rightarrow t^{2} = 3$

$∴ a = 5 and Q \equiv (5, 0)$

$∵$ Focus of parabola is (1, 0) and centre of circle lie on axis of parabola.
$\Rightarrow$ (1, 0) and (5, 0) will be the end points of diameter of the circle.

$∴$ Equation of circle is $(x - 1) (x - 5) + y^{2} = 0$

$\Rightarrow x^{2} + y^{2} - 6 x + 5 = 0$ .

Q 22 :

If the equation of the parabola with vertex $V (\frac{3}{2}, 3)$ and the directrix x + 2y = 0 is $α x^{2} + β y^{2} - γ x y - 30 x - 60 y + 225 = 0$ , then $α + β + γ$ is equal to : [2025]

9
6
8
7

1

2

3

4

(1)

Given : Vertex of parabola $(\frac{3}{2}, 3)$ and directrix is x + 2y = 0

Since, axis is $⊥$ to directrix and passes through vertex, then equation of axis

$y - 3 = 2 (x - \frac{3}{2}) \Rightarrow y = 2 x - 3 + 3 \Rightarrow y = 2 x$

$∴$ Foot of directrix is intersecting point of

y = 2x & 2y + x = 0 i.e., (0, 0)

$∴$ Focus $\equiv$ (3, 6)

Using definition of parabola,

$P S^{2} = P M^{2}$

$\Rightarrow {(x - 3)}^{2} + {(y - 6)}^{2} = {(\frac{x + 2 y}{\sqrt{5}})}^{2}$

$\Rightarrow x^{2} + 9 - 6 x + y^{2} + 36 - 12 y = \frac{x^{2} + 4 y^{2} + 4 x y}{5}$

$\Rightarrow 4 x^{2} + y^{2} - 4 x y - 30 x - 60 y + 225 = 0$

On comparing we get $α = 4, β = 1 and γ = 4$

Hence, $α + β + γ$ = 4 + 1 + 4 = 9.

Q 23 :

Let ABCD be a trapezium whose vertices lie on the parabola $y^{2} = 4 x$ . Let the sides AD and BC of the trapezium be parallel to y-axis. If the diagonal AC is of length $\frac{25}{4}$ and it passes through the point (1, 0), then the area of ABCD is [2025]

$\frac{25}{2}$
$\frac{125}{8}$
$\frac{75}{4}$
$\frac{75}{8}$

1

2

3

4

(3)

Let $A (a t_{1}^{2}, 2 a t_{1})$ and $C (\frac{a}{t_{1}^{2}}, \frac{- 2 a}{t_{1}})$ be the points lies on parabola $y^{2} = 4 x$ .

$∴$ Length of $A C = a {(t_{1} + \frac{1}{t_{1}})}^{2} = \frac{25}{4}$ (Given)

$\Rightarrow t_{1} + \frac{1}{t_{1}} = \pm \frac{5}{2}$

So, $A (\frac{1}{4}, 1), B (4, 4), C (4, - 4)$ and $D (\frac{1}{4}, - 1)$

$∴$ The area of trapezium ABCD = $\frac{1}{2} (8 + 2) (4 - \frac{1}{4}) = \frac{75}{4}$ .

Q 24 :

Two parabolas have the same focus (4, 3) and their directrices are the x-axis and the y-axis, respectively. If these parabolas intersects at the points A and B, then ${(A B)}^{2}$ is equal to : [2025]

392
96
384
192

1

2

3

4

(4)

Let the two parabolas intersect at $A (x_{1}, y_{1})$ and $B (x_{2}, y_{2})$ .

$∴$ Equation of parabolas are

${(x - 4)}^{2} + {(y - 3)}^{2} = x^{2}$ ... (i)

and ${(x - 4)}^{2} + {(y - 3)}^{2} = y^{2}$ ... (ii)

From (i) and (ii), we get x = y

$\Rightarrow x^{2} - 14 x + 25 = 0$ and

$\Rightarrow x_{1} + x_{2} = 14 and x_{1} x_{2} = 25$

$∴ A B^{2} = {(x_{1} - x_{2})}^{2} + {(y_{1} - y_{2})}^{2} = 2 {(x_{1} - x_{2})}^{2}$

$= 2 {[(x_{1} + x_{2})}^{2} - 4 x_{1} x_{2}] = 2 [14^{2} - 100] = 192$ .

Q 25 :

The focus of the parabola $y^{2} = 4 x + 16$ is the centre of the circle C of radius 5. If the values of $λ$ , for which C passes through the point of intersection of the lines 3x – y = 0 and x + $λ$ y = 4, are $λ_{1}$ and $λ_{2}, λ_{1} < λ_{2}$ , then $12 λ_{1} + 29 λ_{2}$ is equal to __________. [2025]

(15)

We have, $y^{2} = 4 (x + 4)$

Focus of parabola = (–3, 0) $\Rightarrow$ Center $\equiv$ (–3, 0)

$∴$ Equation of circle is given by ${(x + 3)}^{2} + y^{2} = 25$

Intersection point of 3x – y = 0 and x + $λ$ y = 4 is

$(\frac{4}{3 λ + 1}, \frac{12}{3 λ + 1})$

Circle passes through the point of intersection of two lines 3x – y = 0 and x + $λ$ y = 4.

$\Rightarrow 144 λ^{2} + 24 λ - 168 = 0 \Rightarrow 18 λ^{2} + 3 λ - 21 = 0$

$\Rightarrow λ = - \frac{7}{6}, 1$

Now, $12 λ_{1} + 29 λ_{2}$ = –14 + 29 = 15.

Q 26 :

Let A and B be the two points of intersection of the line y + 5 = 0 and the mirror image of the parabola $y^{2} = 4 x$ with respect to the line x + y + 4 = 0. If d denotes the distance between A and B, and $a$ denotes the area of $△$ SAB, where S is the focus of the parabola $y^{2} = 4 x$ , then the value of (a + d) is __________. [2025]

(14)

Image of point (0, 0) w.r.t. to line x + y + 4 = 0

$\frac{x - x_{1}}{a} = \frac{y - y_{1}}{b} = - 2 \frac{(a x_{1} + b y_{1} + c)}{a^{2} + b^{2}}$

$\frac{x - 0}{1} = \frac{y - 0}{1} = \frac{- 2 (4)}{2} \Rightarrow x = y = - 4$

Image of focus (1, 0) w.r.t. to line x + y + 4 = 0

$\frac{x - 1}{1} = \frac{y - 0}{1} = - 2 \frac{(1 + 4)}{2} x = - 4; y = - 5$

Equation of mirror image of parabola

${(x - h)}^{2} = - 4 a (y - k) {(x + 4)}^{2} = - 4 (1) (y + 4)$

Put y = –5; we get x = –6 and –2

$∴$ A = (–6, –5); B = (–2, –5)

Distance between the points, d = AB = 4

Area of $△$ SAB, $a = \frac{1}{2} \times 4 \times 5 = 10$

So, a + d = 14.

Q 27 :

Let $y^{2} = 12 x$ be the parabola and S be its focus. Let PQ be a focal chord of the parabola such that (SP)(SQ) = $\frac{147}{4}$ . Let C be the circle described taking PQ as a diameter. If the equation of a circle C is $64 x^{2} + 64 y^{2} - α x - 64 \sqrt{3} y = β$ , then $β - α$ is equal to __________. [2025]

(1328)

Given, equation of parabola is $y^{2} = 12 x$

Focus = S = (3, 0)

Let $P (3 t_{1}^{2}, 6 t_{1})$ and $Q (3 t_{2}^{2}, 6 t_{2})$ are points on parabola

Also, $t_{1} t_{2} = - 1$

$∴ P \equiv (3 t^{2}, 6 t) and Q \equiv (\frac{3}{t^{2}}, \frac{- 6}{t})$

Now, $(S P) (S Q) = \frac{147}{4} \Rightarrow (3 + 3 t^{2}) (3 + \frac{3}{t^{2}}) = \frac{147}{4}$

( $∵$ (SP) = PM and (SQ) = QN, where PM and QN are perpendicular distance from directrix)

$\Rightarrow \frac{{(1 + t^{2})}^{2}}{t^{2}} = \frac{49}{12} \Rightarrow 12 t^{4} - 25 t^{2} + 12 = 0$

$\Rightarrow t^{2} = \frac{3}{4}, \frac{4}{3} \Rightarrow t = \pm \frac{\sqrt{3}}{2} or t = \pm \frac{2}{\sqrt{3}}$

Case I : When $t = \frac{\sqrt{3}}{2} or t = - \frac{2}{\sqrt{3}}$

Points are $P (\frac{9}{4}, 3 \sqrt{3}) and Q (4, - 4 \sqrt{3})$

$∴$ Equation of circle is

$(x - 4) (x - \frac{9}{4}) + (y - 3 \sqrt{3}) (y + 4 \sqrt{3}) = 0$

$\Rightarrow x^{2} + y^{2} - \frac{25 x}{4} + \sqrt{3} y - 27 = 0$

On comparing with given equation of circle $64 x^{2} + 64 y^{2} - α x$

$- 64 \sqrt{3} y = β$ , we get $α$ = 400, $β$ = 1728

Case II : When $t = \frac{- \sqrt{3}}{2} or t = \frac{2}{\sqrt{3}}$

Point are $P (\frac{9}{4}, - 3 \sqrt{3}) and Q (4, 4 \sqrt{3})$

Similarly, we get $α$ = 400, $β$ = 1728

$∴ β - α$ = 1728 – 400 = 1328.

Q 28 :

Let R be the focus of the parabola $y^{2} = 20 x$ and the line $y = m x + c$ intersect the parabola at two points P and Q. Let the point G(10,10) be the centroid of the triangle PQR. If $c - m$ = 6, then ${(P Q)}^{2}$ is [2023]

296
325
346
317

1

2

3

4

(2)

$We have y^{2} = 20 x$ $\dots (i)$

$∴$ $Focus, R \equiv (5, 0)$

$Now, put x = \frac{y - c}{m} in y^{2} = 20 x$

$\Rightarrow y^{2} = 20 (\frac{y - c}{m})$

$\Rightarrow m y^{2} - 20 y + 20 c = 0$

$\Rightarrow m y^{2} - 20 y + 20 (6 + m) = 0$ $(∵ c - m = 6 given)$

$Let y_{1}$ and $y_{2} be the roots, then$

$y_{1} + y_{2} = \frac{20}{m}$ $\dots (i i)$

Now centroid of $∆ P Q R$ is at (10,10)

$∴$ $\frac{y_{1} + y_{2} + 0}{3} = 10$

$\Rightarrow y_{1} + y_{2} = 30$ $\dots (i i i)$

$From (ii) and (iii), we get$

$\frac{20}{m} = 30 \Rightarrow m = \frac{20}{30} = \frac{2}{3}$

$Now, c = 6 + m = 6 + \frac{2}{3} = \frac{20}{3}$

$∴$ $Equation of line P Q is y = \frac{2}{3} x + \frac{20}{3}$

$\Rightarrow 2 x - 3 y + 20 = 0$ $\dots (i v)$

$Now, solving equation (i) and (iv), we get$

$P \equiv (5, 10), Q \equiv (20, 20)$

$∴$ ${(P Q)}^{2} = 15^{2} + 10^{2} = 325$

Q 29 :

Let A(0, 1), B(1, 1) and C(1, 0) be the mid-points of the sides of a triangle with incentre at the point D. If the focus of the parabola $y^{2} = 4 a x$ passing through D is $(α + β \sqrt{2}, 0)$ , where $α$ and $β$ are rational numbers, then $\frac{α}{β^{2}}$ is equal to [2023]

12
6
$\frac{9}{2}$
8

1

2

3

4

(4)

$a = O P = 2, b = O Q = 2, c = P Q = 2 \sqrt{2}$

$Incentre is given by$

$D \equiv (\frac{a x_{1} + b x_{2} + c x_{3}}{a + b + c}, \frac{a y_{1} + b y_{2} + c y_{3}}{a + b + c})$

$D \equiv (\frac{4}{2 + 2 + 2 \sqrt{2}}, \frac{4}{2 + 2 + 2 \sqrt{2}}) \equiv (2 - \sqrt{2}, 2 - \sqrt{2})$

Now, $y^{2} = 4 a x$ passes through $D$ .

So, ${(2 - \sqrt{2})}^{2} = 4 \times a (2 - \sqrt{2}) \Rightarrow a = \frac{2 - \sqrt{2}}{4}$

The focus of parabola $y^{2} = 4 a x$ is $(a, 0)$

So, $(\frac{2 - \sqrt{2}}{4}, 0) \equiv (α + β \sqrt{2}, 0)$

$\Rightarrow α = \frac{2}{4} = \frac{1}{2}, β = - \frac{1}{4}$

$∴$ $\frac{α}{β^{2}} = 8$

Q 30 :

Let PQ be a focal chord of the parabola $y^{2} = 36 x$ of length 100, making an acute angle with the positive $x$ -axis. Let the ordinate of P be positive and M be the point on the line segment PQ such that PM : MQ = 3 : 1. Then which of the following points does NOT lie on the line passing through M and perpendicular to the line PQ? [2023]

(3, 33)
(- 6, 45)
(6, 29)
(- 3, 43)

1

2

3

4

(4)

$We have y^{2} = 36 x \dots (i)$

$Length of P Q = 100 units$

$P M : M Q = 3 : 1$

$y^{2} = 4 a x \dots (i i)$

$On comparing (i) and (ii), we get a = 9$

$∴$ $Now, the coordinates of P \equiv (9 t^{2}, 18 t)$ $and coordinate of Q \equiv (\frac{9}{t^{2}}, \frac{18}{t})$

$P Q = a {(t + \frac{1}{t})}^{2} \Rightarrow 100 = {(t + \frac{1}{t})}^{2}$

$\Rightarrow t + \frac{1}{t} = \pm \frac{10}{3}$

$\Rightarrow 3 t^{2} + 3 = 10 t \Rightarrow 3 t^{2} - 10 t + 3 = 0 \Rightarrow (3 t - 1) (t - 3) = 0$

$\Rightarrow t = \frac{1}{3}, 3$

or $t + \frac{1}{t} = \frac{- 10}{3} \Rightarrow 3 t^{2} + 3 = - 10 t \Rightarrow 3 t^{2} + 10 t + 3 = 0$

$\Rightarrow (3 t + 1) (t + 3) = 0 \Rightarrow t = \frac{- 1}{3}, - 3$

$∴$ $Coordinates of P are (81, 54) and coordinates of Q are (1, - 6)$

$Now, the coordinates of M are$ $(\frac{3 (1) + 81}{4}, \frac{3 (- 6) + 54}{4}) \equiv (21, 9)$

$Slope of P Q = \frac{- 6 - 54}{1 - 81} = \frac{3}{4}$

$∴$ $Slope of the line perpendicular to P Q = \frac{- 4}{3}$

$Therefore, the equation of line passing through M and perpendicular to P Q is given by$

$y - 9 = \frac{- 4}{3} (x - 21) \Rightarrow 4 x + 3 y = 111$

$Only point (- 3, 43) does not satisfy the equation 4 x + 3 y = 111 .$

Topic Question Set

Q 1 :

Let PQ be a chord of the parabola $y^{2} = 12 x$ and the midpoint of PQ be at (4, 1). Then, which of the following point lies on the line passing through the points P and Q? [2024]

Q 2 :

Let C be the circle of minimum area touching the parabola $y = 6 - x^{2}$ and the lines $y = \sqrt{3} | x |$ . Then, which one of the following points lies on the circle C? [2024]

Q 3 :

If the shortest distance of the parabola $y^{2} = 4 x$ from the centre of the circle $x^{2} + y^{2} - 4 x - 16 y + 64 = 0$ is d, then $d^{2}$ is equal to : [2024]

Q 4 :

Let the length of the focal chord PQ of the parabola $y^{2} = 12 x$ be 15 units. If the distance of PQ from the origin is p, then $10 p^{2}$ is equal to __________. [2024]

Q 5 :

Suppose AB is a focal chord of the parabola $y^{2} = 12 x$ of length $l$ and slope $m < \sqrt{3}$ . If the distance of the chord AB from the origin is d, then $l d^{2}$ is equal to __________. [2024]

Q 6 :

Let a line perpendicular to the line 2x – y = 10 touch the parabola $y^{2} = 4 (x - 9)$ at the point P. The distance of the point P from the centre of the circle $x^{2} + y^{2} - 14 x - 8 y + 56 = 0$ is __________. [2024]

Q 12 :

Let $P (α, β)$ be a point on the parabola $y^{2} = 4 x$ . If P also lies on the chord of the parabola $x^{2} = 8 y$ whose mid point is $(1, \frac{5}{4})$ , then $(α - 28) (β - 8)$ is equal to __________. [2024]

Q 14 :

Let the point P of the focal chord PQ of the parabola $y^{2} = 16 x$ be (1, –4). If the focus of the parabola divides the chord PQ in the ratio m : n, gcd (m, n) = 1, then $m^{2} + n^{2}$ is equal to : [2025]

Q 15 :

The radius of the smallest circle which touches the parabolas $y = x^{2} + 2$ and $x = y^{2} + 2$ is [2025]

Q 16 :

The axis of a parabola is the line y = x and its vertex and focus are in the first quadrant at distances $\sqrt{2}$ and $2 \sqrt{2}$ units from the origin, respectively. If the point (1, k) lies on the parabola, then a possible value of k is : [2025]

Q 17 :

Let P be the parabola, whose focus is (–2, 1) and directrix is 2x + y + 2 = 0. Then the sum of the ordinates of the points on P, whose abscissa is –2, is [2025]

Q 18 :

Let the parabola $y = x^{2} + p x - 3$ , meet the coordinate axes at the points P, Q and R. If the circle C with centre at (–1, –1) passes through the points P, Q and R, then the area of $△$ PQR is : [2025]

Q 19 :

Let $P (4, 4 \sqrt{3})$ be a point on the parabola $y^{2} = 4 a x$ and PQ be a focal chord of the parabola. If M and N are the foot of perpendiculars drawn from P and Q respectively on the directrix of the parabola, then the area of the quadrilateral PQMN is equal to : [2025]

Q 20 :

If the line 3x – 2y + 12 = 0 intersects the parabola $4 y = 3 x^{2}$ at the points A and B, then at the vertex of the parabola, the line segment AB subtends an angle equal to [2025]

Q 21 :

Let the shortest distance from (a, 0), a > 0, to the parabola $y^{2} = 4 x$ be 4. Then the equation of the circle passing through the point (a, 0) and the focus of the parabola, and having its centre on the axis of the parabola is: [2025]

Q 22 :

If the equation of the parabola with vertex $V (\frac{3}{2}, 3)$ and the directrix x + 2y = 0 is $α x^{2} + β y^{2} - γ x y - 30 x - 60 y + 225 = 0$ , then $α + β + γ$ is equal to : [2025]

Q 23 :

Let ABCD be a trapezium whose vertices lie on the parabola $y^{2} = 4 x$ . Let the sides AD and BC of the trapezium be parallel to y-axis. If the diagonal AC is of length $\frac{25}{4}$ and it passes through the point (1, 0), then the area of ABCD is [2025]

Q 24 :

Two parabolas have the same focus (4, 3) and their directrices are the x-axis and the y-axis, respectively. If these parabolas intersects at the points A and B, then ${(A B)}^{2}$ is equal to : [2025]

Q 28 :

Let R be the focus of the parabola $y^{2} = 20 x$ and the line $y = m x + c$ intersect the parabola at two points P and Q. Let the point G(10,10) be the centroid of the triangle PQR. If $c - m$ = 6, then ${(P Q)}^{2}$ is [2023]

Q 29 :

Let A(0, 1), B(1, 1) and C(1, 0) be the mid-points of the sides of a triangle with incentre at the point D. If the focus of the parabola $y^{2} = 4 a x$ passing through D is $(α + β \sqrt{2}, 0)$ , where $α$ and $β$ are rational numbers, then $\frac{α}{β^{2}}$ is equal to [2023]

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 1 : Let PQ be a chord of the parabola y2=12x and the midpoint of PQ be at (4, 1). Then, which of the following point lies on the line passing through the points P and Q? [2024]

Q 2 : Let C be the circle of minimum area touching the parabola y=6–x2 and the lines y=3|x|. Then, which one of the following points lies on the circle C? [2024]

Q 3 : If the shortest distance of the parabola y2=4x from the centre of the circle x2+y2–4x–16y+64=0 is d, then d2 is equal to : [2024]

Q 4 : Let the length of the focal chord PQ of the parabola y2=12x be 15 units. If the distance of PQ from the origin is p, then 10p2 is equal to __________. [2024]

Q 5 : Suppose AB is a focal chord of the parabola y2=12x of length l and slope m<3. If the distance of the chord AB from the origin is d, then ld2 is equal to __________. [2024]

Q 6 : Let a line perpendicular to the line 2x – y = 10 touch the parabola y2=4(x–9) at the point P. The distance of the point P from the centre of the circle x2+y2–14x–8y+56=0 is __________. [2024]

Q 9 : Let A, B and C be three points on the parabola y2=6x and let the line segment AB meet the line L through C parallel to the x-axis at the point D. Let M and N respectively be the feet of the perpendiculars from A and B on L. then (AM·BNCD)2 is equal to __________. [2024]

Q 10 : Consider the circle C:x2+y2=4 and the parabola P:y2=8x. If the set of all values of α, for which three chords of the circle C on three distinct lines passing through the point (α,0) are bisected by the parabola P is the interval (p, q), then (2q–p)2 is equal to __________. [2024]

Q 12 : Let P(α, β) be a point on the parabola y2=4x. If P also lies on the chord of the parabola x2=8y whose mid point is (1, 54), then (α–28)(β–8) is equal to __________. [2024]

Q 13 : Let the focal chord PQ of the parabola y2=4x make an angle of 60° with the positive x-axis, where P lies in the first quadrant. If the circle, whose one diameter is PS, S being the focus of the parabola, touches the y-axis at the point (0, α), then 5α2 is equal to : [2025]

Q 14 : Let the point P of the focal chord PQ of the parabola y2=16x be (1, –4). If the focus of the parabola divides the chord PQ in the ratio m : n, gcd (m, n) = 1, then m2+n2 is equal to : [2025]

Q 15 : The radius of the smallest circle which touches the parabolas y=x2+2 and x=y2+2 is [2025]

Q 16 : The axis of a parabola is the line y = x and its vertex and focus are in the first quadrant at distances 2 and 22 units from the origin, respectively. If the point (1, k) lies on the parabola, then a possible value of k is : [2025]

Q 17 : Let P be the parabola, whose focus is (–2, 1) and directrix is 2x + y + 2 = 0. Then the sum of the ordinates of the points on P, whose abscissa is –2, is [2025]

Q 18 : Let the parabola y=x2+px–3, meet the coordinate axes at the points P, Q and R. If the circle C with centre at (–1, –1) passes through the points P, Q and R, then the area of △PQR is : [2025]

Q 19 : Let P(4,43) be a point on the parabola y2=4ax and PQ be a focal chord of the parabola. If M and N are the foot of perpendiculars drawn from P and Q respectively on the directrix of the parabola, then the area of the quadrilateral PQMN is equal to : [2025]

Q 20 : If the line 3x – 2y + 12 = 0 intersects the parabola 4y=3x2 at the points A and B, then at the vertex of the parabola, the line segment AB subtends an angle equal to [2025]

Q 21 : Let the shortest distance from (a, 0), a > 0, to the parabola y2=4x be 4. Then the equation of the circle passing through the point (a, 0) and the focus of the parabola, and having its centre on the axis of the parabola is: [2025]

Q 22 : If the equation of the parabola with vertex V(32,3) and the directrix x + 2y = 0 is αx2+βy2–γxy–30x–60y+225=0, then α+β+γ is equal to : [2025]

Q 23 : Let ABCD be a trapezium whose vertices lie on the parabola y2=4x. Let the sides AD and BC of the trapezium be parallel to y-axis. If the diagonal AC is of length 254 and it passes through the point (1, 0), then the area of ABCD is [2025]

Q 24 : Two parabolas have the same focus (4, 3) and their directrices are the x-axis and the y-axis, respectively. If these parabolas intersects at the points A and B, then (AB)2 is equal to : [2025]

Q 25 : The focus of the parabola y2=4x+16 is the centre of the circle C of radius 5. If the values of λ, for which C passes through the point of intersection of the lines 3x – y = 0 and x + λy = 4, are λ1 and λ2,λ1<λ2, then 12λ1+29λ2 is equal to __________. [2025]

Q 27 : Let y2=12x be the parabola and S be its focus. Let PQ be a focal chord of the parabola such that (SP)(SQ) = 1474. Let C be the circle described taking PQ as a diameter. If the equation of a circle C is 64x2+64y2–αx–643y=β, then β–α is equal to __________. [2025]

Q 28 : Let R be the focus of the parabola y2=20x and the line y=mx+c intersect the parabola at two points P and Q. Let the point G(10,10) be the centroid of the triangle PQR. If c-m = 6, then (PQ)2 is [2023]

Q 29 : Let A(0, 1), B(1, 1) and C(1, 0) be the mid-points of the sides of a triangle with incentre at the point D. If the focus of the parabola y2=4ax passing through D is (α+β2,0), where α and β are rational numbers, then αβ2 is equal to [2023]

Want Us to Email you About Special Offers & Updates?

Q 1 :

Let PQ be a chord of the parabola $y^{2} = 12 x$ and the midpoint of PQ be at (4, 1). Then, which of the following point lies on the line passing through the points P and Q? [2024]

Q 2 :

Let C be the circle of minimum area touching the parabola $y = 6 - x^{2}$ and the lines $y = \sqrt{3} | x |$ . Then, which one of the following points lies on the circle C? [2024]

Q 3 :

If the shortest distance of the parabola $y^{2} = 4 x$ from the centre of the circle $x^{2} + y^{2} - 4 x - 16 y + 64 = 0$ is d, then $d^{2}$ is equal to : [2024]

Q 4 :

Let the length of the focal chord PQ of the parabola $y^{2} = 12 x$ be 15 units. If the distance of PQ from the origin is p, then $10 p^{2}$ is equal to __________. [2024]

Q 5 :

Suppose AB is a focal chord of the parabola $y^{2} = 12 x$ of length $l$ and slope $m < \sqrt{3}$ . If the distance of the chord AB from the origin is d, then $l d^{2}$ is equal to __________. [2024]

Q 6 :

Let a line perpendicular to the line 2x – y = 10 touch the parabola $y^{2} = 4 (x - 9)$ at the point P. The distance of the point P from the centre of the circle $x^{2} + y^{2} - 14 x - 8 y + 56 = 0$ is __________. [2024]

Q 12 :

Let $P (α, β)$ be a point on the parabola $y^{2} = 4 x$ . If P also lies on the chord of the parabola $x^{2} = 8 y$ whose mid point is $(1, \frac{5}{4})$ , then $(α - 28) (β - 8)$ is equal to __________. [2024]

Q 14 :

Let the point P of the focal chord PQ of the parabola $y^{2} = 16 x$ be (1, –4). If the focus of the parabola divides the chord PQ in the ratio m : n, gcd (m, n) = 1, then $m^{2} + n^{2}$ is equal to : [2025]

Q 15 :

The radius of the smallest circle which touches the parabolas $y = x^{2} + 2$ and $x = y^{2} + 2$ is [2025]

Q 16 :

The axis of a parabola is the line y = x and its vertex and focus are in the first quadrant at distances $\sqrt{2}$ and $2 \sqrt{2}$ units from the origin, respectively. If the point (1, k) lies on the parabola, then a possible value of k is : [2025]

Q 17 :

Let P be the parabola, whose focus is (–2, 1) and directrix is 2x + y + 2 = 0. Then the sum of the ordinates of the points on P, whose abscissa is –2, is [2025]

Q 18 :

Let the parabola $y = x^{2} + p x - 3$ , meet the coordinate axes at the points P, Q and R. If the circle C with centre at (–1, –1) passes through the points P, Q and R, then the area of $△$ PQR is : [2025]

Q 19 :

Let $P (4, 4 \sqrt{3})$ be a point on the parabola $y^{2} = 4 a x$ and PQ be a focal chord of the parabola. If M and N are the foot of perpendiculars drawn from P and Q respectively on the directrix of the parabola, then the area of the quadrilateral PQMN is equal to : [2025]

Q 20 :

If the line 3x – 2y + 12 = 0 intersects the parabola $4 y = 3 x^{2}$ at the points A and B, then at the vertex of the parabola, the line segment AB subtends an angle equal to [2025]

Q 21 :

Let the shortest distance from (a, 0), a > 0, to the parabola $y^{2} = 4 x$ be 4. Then the equation of the circle passing through the point (a, 0) and the focus of the parabola, and having its centre on the axis of the parabola is: [2025]

Q 22 :

If the equation of the parabola with vertex $V (\frac{3}{2}, 3)$ and the directrix x + 2y = 0 is $α x^{2} + β y^{2} - γ x y - 30 x - 60 y + 225 = 0$ , then $α + β + γ$ is equal to : [2025]

Q 23 :

Let ABCD be a trapezium whose vertices lie on the parabola $y^{2} = 4 x$ . Let the sides AD and BC of the trapezium be parallel to y-axis. If the diagonal AC is of length $\frac{25}{4}$ and it passes through the point (1, 0), then the area of ABCD is [2025]

Q 24 :

Two parabolas have the same focus (4, 3) and their directrices are the x-axis and the y-axis, respectively. If these parabolas intersects at the points A and B, then ${(A B)}^{2}$ is equal to : [2025]

Q 28 :

Let R be the focus of the parabola $y^{2} = 20 x$ and the line $y = m x + c$ intersect the parabola at two points P and Q. Let the point G(10,10) be the centroid of the triangle PQR. If $c - m$ = 6, then ${(P Q)}^{2}$ is [2023]

Q 29 :

Let A(0, 1), B(1, 1) and C(1, 0) be the mid-points of the sides of a triangle with incentre at the point D. If the focus of the parabola $y^{2} = 4 a x$ passing through D is $(α + β \sqrt{2}, 0)$ , where $α$ and $β$ are rational numbers, then $\frac{α}{β^{2}}$ is equal to [2023]