Topic Question Set
Q 1
:
Let the line 2x + 3y – k = 0, k > 0, intersect the x-axis and y-axis at the points A and B, respectively. If the equation of the circle having the line segment AB as a diameter is and the length of the latus rectum of the ellipse is , where m and n are coprime, then 2m + n is equal to [2024]
10
13
12
11
(4)
Equation of circle with AB as diameter is
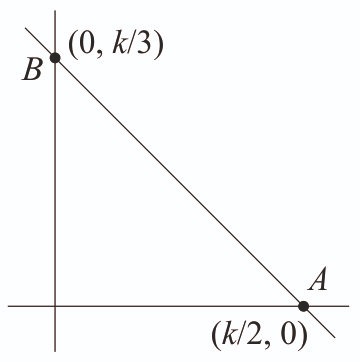
On comparing it with given equation
we get
Now, equation of ellipse is given by
So latus rectum
.
Q 2
:
Let , and a = fog (10), b = gof (3). If e and denote the eccentricity and the length of the latus rectum of the ellipse , then + is equal to [2024]
6
12
16
8
(4)
We have,
Also
Also,
Now,
So ellipse becomes
and
Hence, + .
Q 3
:
Let , a > b be an ellipse, whose eccentricity is and the length of the latusrectum is . Then the square of the eccentricity of is: [2024]
3
3/2
7/2
5/2
(2)
Q 4
:
Let P be a point on the ellipse , Let the line passing through P and parallel to y-axis meet the circle at point Q such that P and Q are on the same side of the x-axis. then, the eccentricity of the locus of the point R and PQ such that PR : RQ = 4 : 3 as P moves on the ellipse, is [2024]
(3)
As, P be a point on the ellipse.
Coordinates of P = (3 cos , 2 sin )
Also, Q is a point on the circle
Coordinates of Q = (3 cos , 3 sin )
Now, R(h, k) divides the line PQ in the ratio 4 : 3.
and
Now,
So, locus of R, is ellipse.
Eccentricity = .
Q 5
:
The length of the chord of the ellipse , whose mid point is , is equal to : [2024]
(2)
Ellipse : ... (i)
Equation of chord having mid point is
...(ii)
From equation (i) & (ii), we get
Length of Chord
Q 6
:
If the length of the minor axis of an ellipse is equal to half of the distance between the foci, then the eccentricity of the ellipse is : [2024]
(2)
Let be the given ellipse
We have, minor axis , where e is eccentricity
[]
Now,
Q 7
:
Let A(, 0) and B(0, ) be the points on the line 5x + 7y = 50. Let the point P divide the line segment AB internally in the ratio 7 : 3. Let 3x – 25 = 0 be a directrix of the ellipse , and the corresponding focus be S. If from S, the perpendicular on the x-axis passes through P, then the length of the latus rectum of E is equal to, [2024]
(1)

A(, 0) and B(0, ) be the points on the line 5x + 7y = 50
Using section formula, we have P(3, 5).
Directrix :
The equation of the line passing through P(3, 5) and perpendicular to x-axis is x = 3.
The perpendicular is also passes through S.
ae = 3
Length of latus rectum .
Q 8
:
Let P be a parabola with vertex (2, 3) and directrix 2x + y = 6. Let an ellipse , a > b of eccentricity pass through the focus of the parabola P. Then the square of the length of the latus rectum of E is [2024]
(2)
Given that vertex of parabola P is (2, 3) and equation of directrix is 2x + y = 6 ... (i)
Let S(, ) be the focus of parabola.
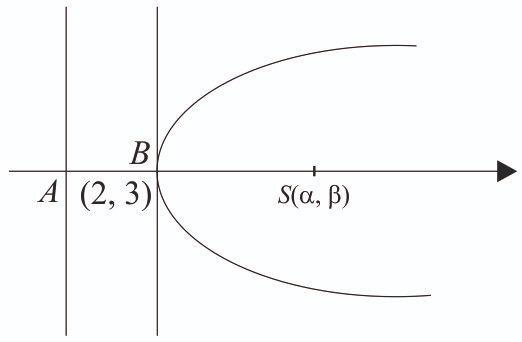
Equation of axis of AS is
... (ii)
Solving equations (i) and (ii), we get
Now, using mid point formula, we have
The point will satisfy the ellipse.
... (iii)
Now,
From (iii),
Length of latus rectum
Square of the latus rectum .
Q 9
:
If the points of intersection of two distinct conics and lie on the curve , then times the area of the rectangle formed by the intersection points is _________. [2024]
(432)
We have, ... (i)
and ... (ii)
From (i) and (ii), and
These points lie on curve .
So,
So, the points are
Area of rectangle = sq. units
Thus, times of the area of rectangle .
Q 10
:
If S and are the foci of the ellipse and P be a point on the ellipse, then is equal to : [2025]
27
-
9
-
(1)
We have,
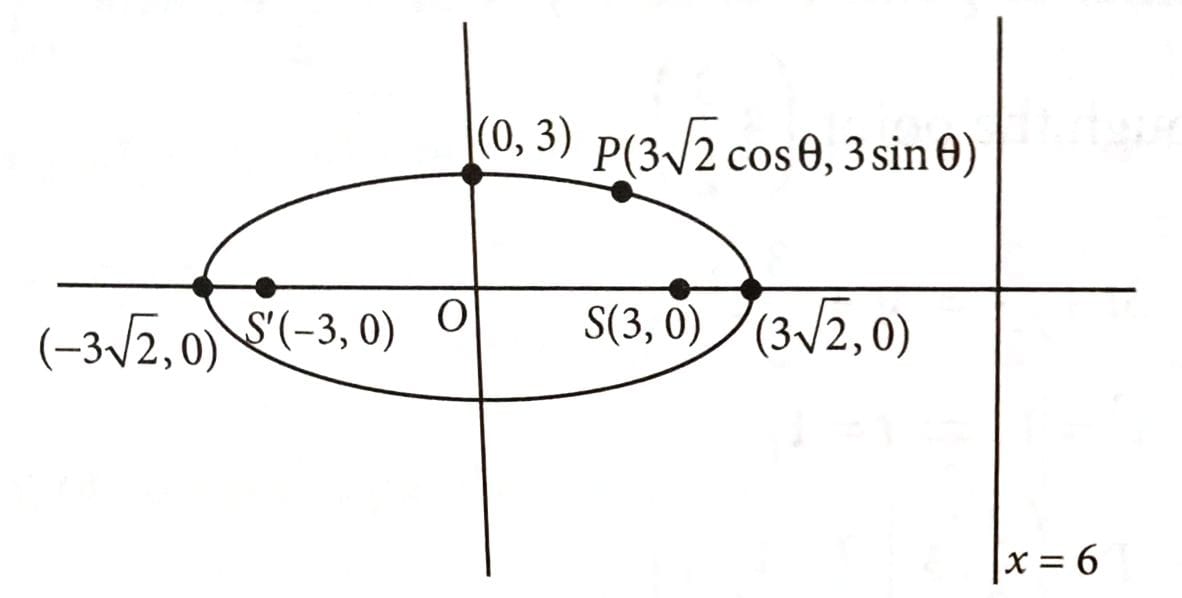
Since,
Also,
Equation of Directrix is
[]
Required sum = 27.

