Topic Question Set
Q 1
:
Let a variable line of slope m > 0 passing through the point (4, –9) intersect the coordinate axes at the points A and B. The minimum value of the sum of the distances of A and B from the origin is [2024]
10
25
30
15
(2)
Equation of line passing through (4, –9) and having slope m is given by
(y + 9) = m(x – 4)
Since it intersect the coordinate axes at points A and B so at A, y = 0
Similarly at B, x = 0
Now, let O(0, 0) be origin, then
Now,
Q 2
:
If the locus of the point, whose distance from the point (2, 1) and (1, 3) are in the ratio 5 : 4, is , then the value of is equal to : [2024]
5
– 27
37
437
(3)
Let given point be (h, k) whose distance from (2, 1) and (1, 3) is in ratio 5 : 4.
On replacing h by x and k by y, we get
On comparing, we get
Q 3
:
If the line segment joining the points (5, 2) and (2, a) subtends an angle at the origin, then the absolute value of the product of all possible values of a is: [2024]
8
2
4
6
(3)
Angle between the lines is
Since,
Required product .
Q 4
:
A ray of light coming from the point P(1, 2) gets reflected from the point Q on the x-axis and then passes through the point R(4, 3). If the point S(h, k) is such that PQRS is a parallelogram, then is equal to: [2024]
70
90
60
80
(1)
Slope of
Equation of
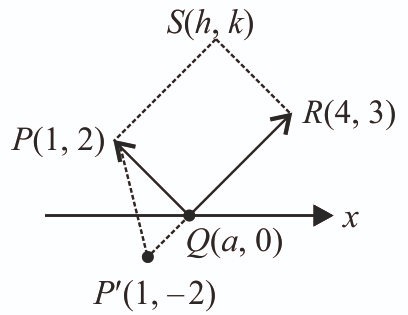
Since, point Q is on x-axis.
Let
Also, point Q lies P'R
Now, PQRS is a parallelogram
and
Q 5
:
Two vertices of a triangle ABC are A(3, –1) and B(–2, 3) and its orthocentre is P(1, 1). If the coordinates of the point C are and the centre of the circle circumscribing the triangle PAB is (h, k), then the value of equals [2024]
15
5
51
81
(2)
Slope of
Slope of
Equation of PC is given
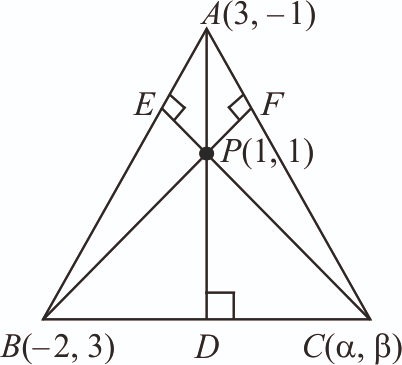
4y – 4 = 5x – 5
5x – 4y – 1 = 0 ... (i)
Now slope of
Slope of BC = 1
Equation of BC is given by
y – 3 = 1(x + 2)
x – y + 5 = 0 ... (ii)
Solving (i) and (ii), we get
and
Centre of circle circumseribing is point of intersection of perpendicular bisector of AP, AB and AC.
Equation of perpendicular bisector of AP
y – 0 = x – 2
y = x – 2 ... (iii)
Equation of perpendicular bisector of AB
... (iv)
On solving (iii) and (iv), we get
So, .
Q 6
:
Let and let and D(1, 2) be the vertices of a parallelogram ABCD. If and the points A and C lie on the line 3y = 2x + 1, then is equal to [2024]
12
10
8
5
(3)
Givem, and D(1, 2).
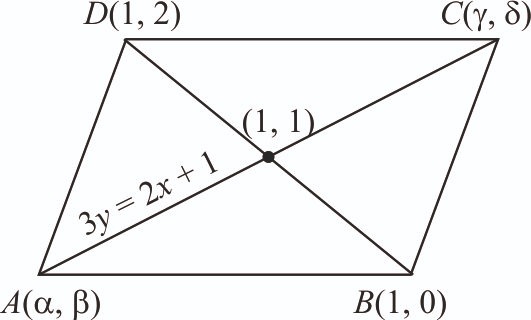
Using mid point Formula.
So,
.
Q 7
:
Let and be the vertices of a parallelogram ABCD. If the point C lies on 2x – y = 5 and the point D lies on 3x – 2y = 6, then the value of is equal to __________. [2024]
(32)
We have, A(–2, –1), B(1, 0), and be the vertices of a parallelogram ABCD.
Since, lies on 2x – y = 5
... (i)
Also, lies on the line 3x – 2y = 6
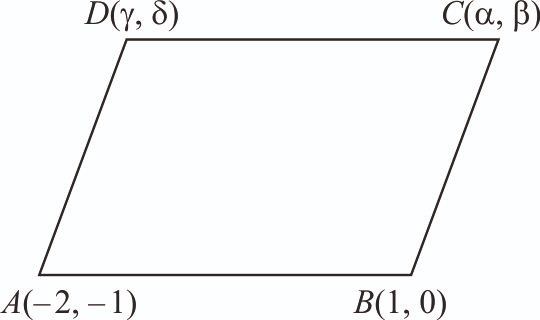
So, ... (ii)
Now,
... (iii)
From (ii),
Solving (i) and (iii), we get
.
Q 8
:
Let the three sides of a triangle are on the lines 4x – 7y + 10 = 0, x + y = 5 and 7x + 4y = 15. Then the distance of its orthocentre from the orthocentre of the triangle formed by the lines x = 0, y = 0 and x + y = 1 is [2025]
-
20
5
-
(1)
Since the lines 4x –7y + 10 = 0 and 7x + 4y = 15 are perpendicular to each other.
So, the triangle formed by lines 4x –7y + 10 = 0, 7x + 4y = 15 and x + y = 5 is right angled triangle.
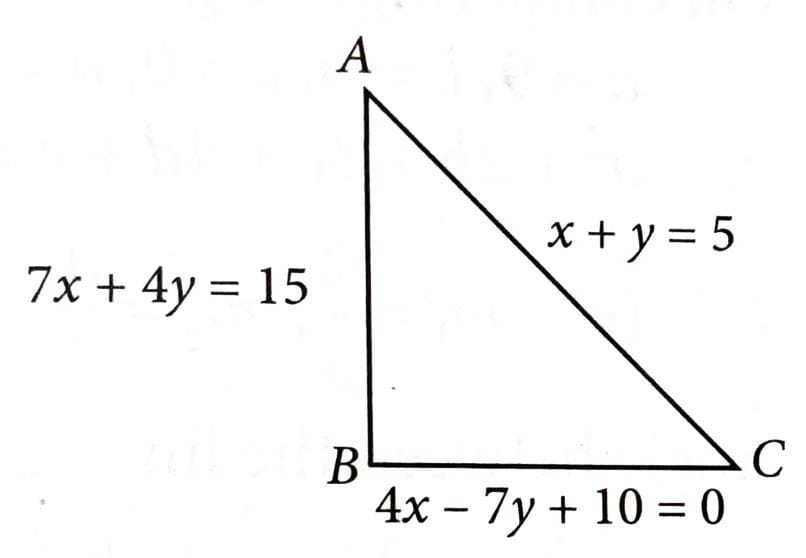
So, orthrocentre = Point of intersection of 4x – 7y + 10 = 0 and 7x + 4y = 15 which is, B = (1, 2)
Also, triangle formed by sides x = 0, y = 0, x + y = 1 is right angled triangle.
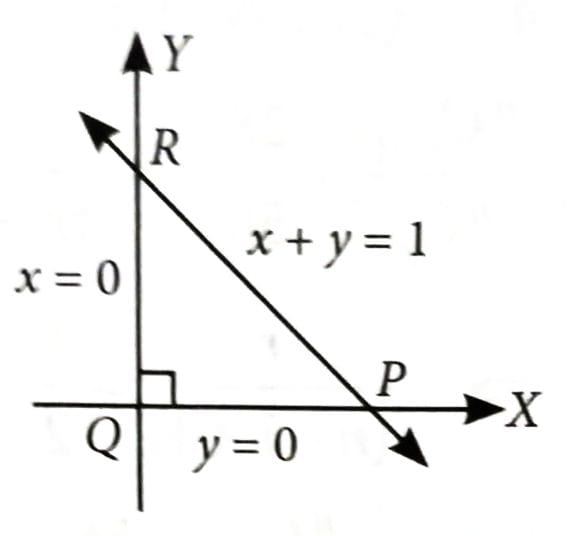
So, orthrocentre, Q = (0, 0)
Distance between Q and .
Q 9
:
Let the area of a PQR with vertices P(5, 4), Q(–2, 4) and R(a, b) be 35 square units. If its orthocenter and centroid are and C(c, d) respectively, then c + 2d is equal to [2025]
2
3
-
-
(2)
Since,
... (i)
Also,
... (ii)
Solving equations (i) and (ii), we get
a = 2, b = - 6

Now, centroid of PQR = (c, d)
.
Q 10
:
Let the points lie on or inside the triangle with sides x + y = 11, x + 2y = 16 and 2x + 3y = 29. Then the product of the smallest and the largest values of is equal to : [2025]
22
55
33
44
(3)
Given that a triangle bounded by lines : x + y = 11, : x + 2y =16 and : 2x + 3y = 29.
The region is given by
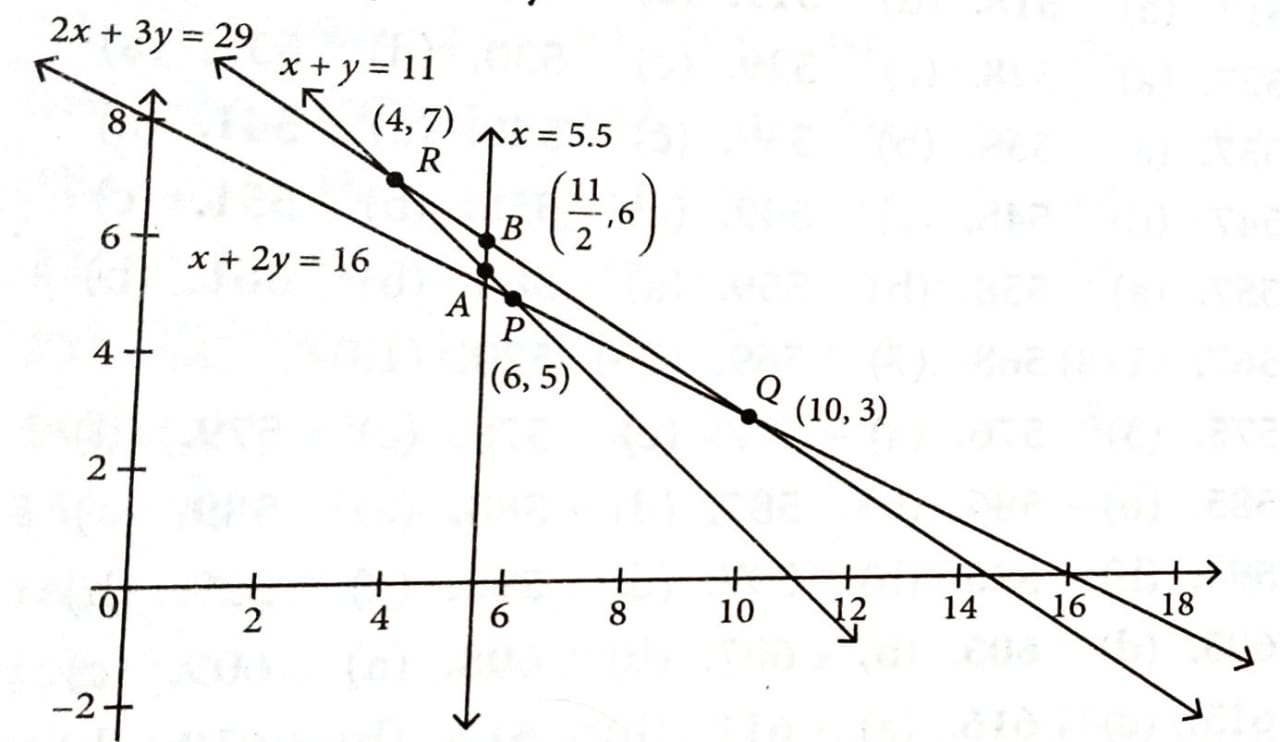
Using : When , y = 11 – x
(minimum)
Using : when x = 11/2, 3y = 29 – 2x
(maximum)
Product of the smallest and the largest value of .

