Topic Question Set
Q 1
:
A spherical ball of radius m and density falls freely under gravity through a distance before entering a tank of water. If after entering in water the velocity of the ball does not change, then the value of is approximately
(The coefficient of viscosity of water is ) [2024]
2296 m
2249 m
2518 m
2396 m
(3)
Terminal velocity,
When ball fall from height
Now
Q 2
:
A small spherical ball of radius , falling through a viscous medium of negligible density has terminal velocity . Another ball of the same mass but of radius , falling through the same viscous medium will have terminal velocity [2024]
(1)
Since density is negligible hence Buoyancy force will be negligible
At terminal velocity,
(as mass is constant)
Now,
So,
Q 3
:
Small water droplets of radius 0.01 mm are formed in the upper atmosphere and falling with a terminal velocity of 10 cm/s. Due to condensation, if 8 such droplets are coalesced and formed a larger drop, the new terminal velocity will be _______ cm/s. [2024]
(40)
Q 4
:
A small ball of mass m and density is dropped in a viscous liquid of density . After some time, the ball falls with constant velocity. The viscous force on the ball is: [2024]
(4)
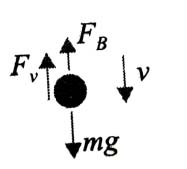
At constant velocity,
Q 5
:
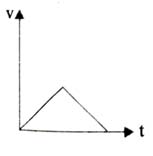
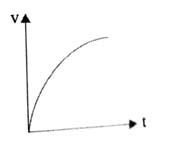
A small steel ball is dropped into a long cylinder containing glycerine. Which one of the following is the correct representation of the velocity-time graph for the transit of the ball? [2024]
(4)
Let
Q 6
:
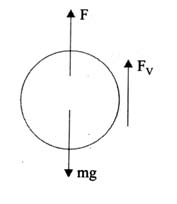
A small rigid spherical ball of mass M is dropped in a long vertical tube containing glycerine. The velocity of the ball becomes constant after some time. If the density of glycerine is half of the density of the ball, then the viscous force acting on the ball will be (consider g as acceleration due to gravity) [2025]
-
-
Mg
2 Mg
(2)
At terminal velocity a = 0
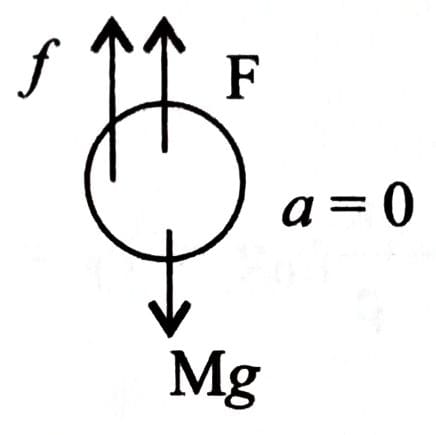
Q 7
:
Given below are two statements:
Statement-I : The hot water flows faster than cold water.
Statement-II : Soap water has higher surface tension as compared to fresh water.
In the light above statements, choose the correct answer from the options given below: [2025]
Statement-I is false but Statement-II is true
Statement-I is true but Statement-II is false
Both Statement-I and Statement-II are true
Both Statement-I and Statement-II are false
(2)
Hot water flows faster because of less viscosity and soap water has less surface tension because bubbles are easily formed.
Q 8
:
A solid steel ball of diameter 3.6 mm acquired terminal velocity while falling under gravity through an oil of density 925 . Take density of steel as 7825 and g as 9.8 . The viscosity of the oil in SI unit is [2025]
2.18
2.38
1.68
1.99
(4)