A sphere of relative density and diameter D has concentric cavity of diameter d. The ratio of , if it just floats on water in a tank is: [2024]
(1)
Weight of sphere,
Buoyant force
For Just Float,
A cube of ice floats partly in water and partly in kerosene oil. The ratio of volume of ice immersed in water to that in kerosene oil (specific gravity of kerosene oil = 0.8, specific gravity of ice = 0.9) [2024]
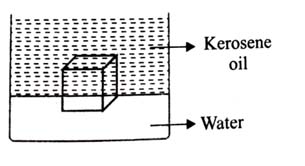
5 : 4
9 : 10
1 : 1
8 : 9
(3)
Buoyancy force balance weight at equilibrium
A 400 g solid cube having an edge of length 10 cm floats in water. How much volume of the cube is outside the water? (Given: density of water = 1000 kg ) [2025]
1400
4000
400
600
(4)
Volume of cube inside water
Volume of cube outside water
= Volume of cube – Volume of cube inside water
= 1000 – 400 = 600
A cube having a side of 10 cm with unknown mass and 200 gm mass were hung at two ends of an uniform rigid rod of 27 cm long. The rod along with masses was placed on a wedge keeping the distance between wedge point and 200 gm weight as 25 cm. Initially the masses were not at balance. A beaker is placed beneath the unknown mass and water is added slowly to it. At given point the masses were in balance and half volume of the unknown mass was inside the water.
(Take the density of unknown mass more than that of the water, the mass did not absorb water and water density is 1 gm/.)The unknown mass is ______ kg. [2025]
(3)
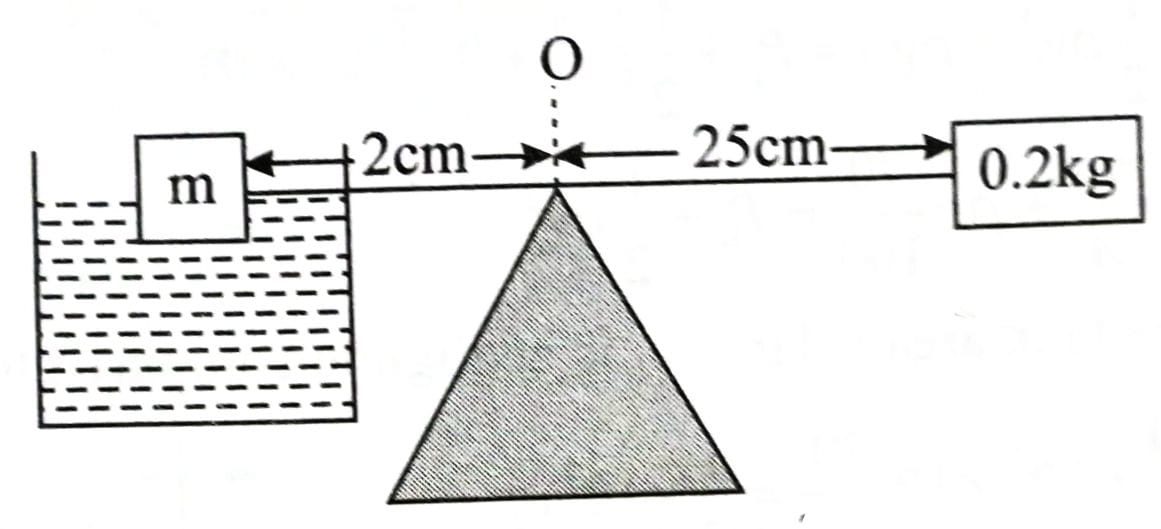
Given, volume of block
Let density of block
mass of block
Buoyant Force
F.B.D. of blocks
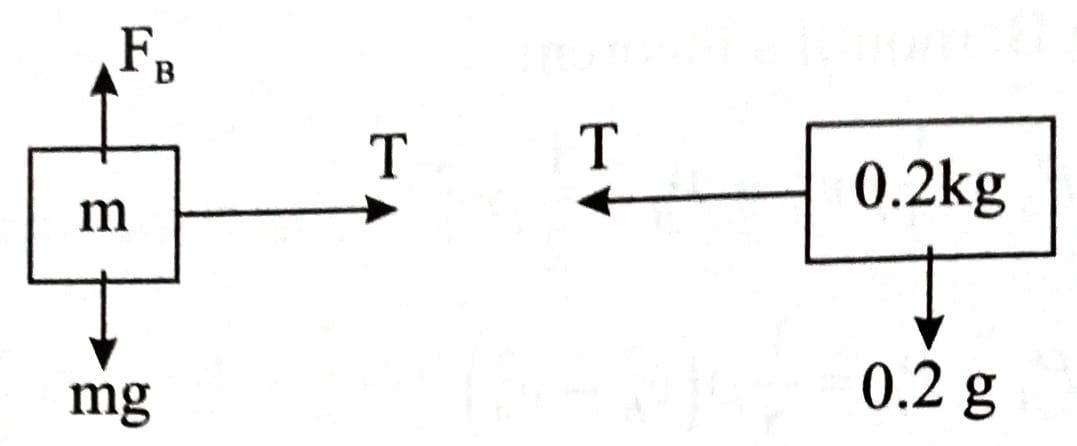
Balancing torque about point O, we get
Hence, mass of block,