Topic Question Set
Q 1
:
The resistances of the platinum wire of a platinum resistance thermometer at the ice point and steam point are and respectively. After inserting in a hot bath of temperature 400°C, the resistance of platinum wire is : [2024]
(3)
Given, and
Also,
Q 2
:
An electric toaster has resistance of at room temperature (27°C). The toaster is connected to a 220 V supply. If the current flowing through it reaches 2.75 A, the temperature attained by toaster is around
(if ) [2024]
694°C
1235°C
1694°C
1667°C
(3)
Q 3
:
Two conductors have the same resistances at but their temperature coefficients of resistance are and . The respective temperature coefficients for their series and parallel combinations are [2024]
(2)
Series:
Parallel:
Neglecting small terms
Q 4
:
Two wires A and B are made up of the same material and have the same mass. Wire A has radius of 2.0 mm and wire B has radius of 4.0 mm. The resistance of wire B is 2 . The resistance of wire A is _______ . [2024]
(32) Given:
We know
...(i)
The volume of wire remains constant,
...(ii)
from equation (i) and equation (ii)
Q 5
:
A wire of resistance R and radius r is stretched till its radius became r/2. If new resistance of the stretched wire is x R. then value of x is _______ . [2024]
(16) We know
As we stretch the wire, its length will increase but its radius will decrease keeping the volume constant.
Q 6
:
Resistance of a wire at 0°C, 100°C and t °C is found to be 10 , 10.2 and 10.95 respectively. The temperature t in Kelvin scale is _______ . [2024]
(748)
Case-I:
...(i)
Case-II:
...(ii)
Q 7
:
At room temperature (27°C) the resistance of a heating element is 50 . The temperature coefficient of the material is . The temperature of the element, when its resistance is 62 , is ______ . [2024]
(1027)
Final temperature,
Q 8
:
In the given circuit, the current in resistance is [2024]
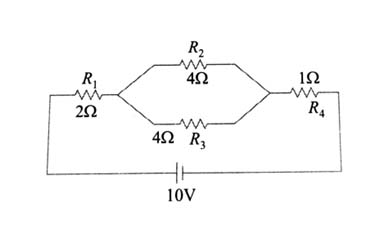

2.5 A
1 A
1.5 A
2 A
(2)

Current in Resistance

