The de-Broglie's wavelength of an electron in the 4th orbit is _________ . ( = Bohr's radius) [2024]
(8) De-Broglie's wavelength () is related to mass (m) and velocity v of electron by:
where is Planck's constant.
By quantization of angular momentum:
Where is orbit number and is radius of orbit.
Rearranging equation II
From I and III
Radius () of orbit is related to radius of first orbit () as:
From IV and V:
Taking atom to be H, Z = 1 and for fourth orbit, n = 4
Frequency of the de-Broglie wave of electron in Bohr's first orbit of hydrogen atoms is ________ Hz (nearest integer). (Given: (Rydberg constant) = J, (Plank's constant) J.s.) [2024]
(656) Velocity () of electron in orbit of single electron specie with atomic number Z is given by:
...(i)
Radius () of orbit of single electron specie with atomic number Z is given by:
...(ii)
Circumference of orbit is integral multiple of wavelength () of electron i.e.
...(iii)
Frequency (v) is related to velocity (v) and wavelength () by:
...(iv)
Put from (iii) in (iv)
...(v)
Put and from (i) and (ii) in (iv)
For H, Z = 1 and for first orbit n = 1
Based on Heisenberg's uncertainty principle, the uncertainty in the velocity of the electron to be found within an atomic nucleus of diameter m is ______ (nearest integer) [Given: mass of electron = kg, Plank's constant Js] (Value of ) [2024]
(58)
Substituting other values:
According to the wave-particle duality of matter by de-Broglie, which of the following graph plot presents most appropriate relationship between wavelength of electron () and momentum of electron (p)? [2024]
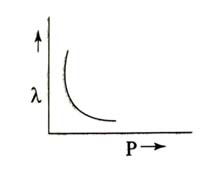

(1)
According to de-Broglie hypothesis, wavelength () of a particle is related to its momentum (p) as:
or
Where is a constant called planck's constant.
Graph of with = constant is a rectangular hyperbola.