Two Dimensional Geometry jee mains questions | JEE Main Two Dimensional Geometry Previous Year Question

Q 11 :
Four distinct points (2k, 3k), (1, 0), (0, 1) and (0, 0) lie on a circle for k equal to : [2024]

$\frac{2}{13}$
$\frac{5}{13}$
$\frac{3}{13}$
$\frac{1}{13}$

1

2

3

4

(2)

Let the equation of circle be

${(x - x_{1})}^{2} + {(y - y_{1})}^{2} = r^{2}$ ...(i)

Put x = 0, y = 0 in (i), we get

$\Rightarrow x_{1}^{2} + y_{1}^{2} = r^{2}$ ... (ii)

Put x = 0, y = 1 in (i), we get

$\Rightarrow x_{1}^{2} + {(1 - y_{1})}^{2} = r^{2}$ ... (iii)

From (ii) and (iii), we get

$y_{1}^{2} = {(1 - y_{1})}^{2} \Rightarrow y_{1}^{2} = 1 + y_{1}^{2} - 2 y_{1} \Rightarrow y_{1} = \frac{1}{2}$

Put x = 1, y = 0 in (i), we get

${(1 - x_{1})}^{2} + y_{1}^{2} = r^{2}$ ... (iv)

From (ii) and (iv), we get

${(1 - x_{1})}^{2} = x_{1}^{2} \Rightarrow 1 + x_{1}^{2} - 2 x_{1} = x_{1}^{2} \Rightarrow x_{1} = \frac{1}{2}$

$∴$ From (ii), we get $r = \frac{1}{\sqrt{2}}$

Putting $x_{1} = y_{1} = \frac{1}{2}$ and $r = \frac{1}{\sqrt{2}}$ in (i), we get

${(x - \frac{1}{2})}^{2} + {(y - \frac{1}{2})}^{2} = \frac{1}{2}$

Point (2k, 3k) also satisfies the equation of circle.

${(2 k - \frac{1}{2})}^{2} + {(3 k - \frac{1}{2})}^{2} = \frac{1}{2}$

$\Rightarrow 4 k^{2} + \frac{1}{4} - 2 k + 9 k^{2} + \frac{1}{4} - 3 k = \frac{1}{2}$

$\Rightarrow 13 k^{2} = 5 k \Rightarrow k = \frac{5}{13}$

Q 12 :
If the circles ${(x + 1)}^{2} + {(y + 2)}^{2} = r^{2}$ and $x^{2} + y^{2} - 4 x - 4 y + 4 = 0$ intersect at exactly two distinct points, then [2024]

5 < r < 9
3 < r < 7
0 < r < 7
$\frac{1}{2} < r < 7$

1

2

3

4

(2)

Let $P : {(x + 1)}^{2} + {(y + 2)}^{2} = r^{2}$ and $Q : x^{2} + y^{2} - 4 x - 4 y + 4 = 0$ be two given circles.

Q can be written as ${(x - 2)}^{2} + {(y - 2)}^{2} = 4$

Centre of circle P and Q are (–1, –2) and (2, 2) respectively

Distance between centre of circle is given by

$D = \sqrt{{(2 + 1)}^{2} + {(2 + 2)}^{2}} = \sqrt{9 + 16} = \sqrt{25} = 5 units$

For the intersection of circles, $D > | r_{P} - r_{Q} |$ and $D < (r_{P} + r_{Q})$ , where $r_{P}$ and $r_{Q}$ are radius of circle P and Q respectively

$\Rightarrow 5 > | r - 2 |$ and 5 < r + 2

$\Rightarrow - 5 < r - 2 < 5 \Rightarrow - 3 < r < 7$ ... (i)

and r > 3 ... (ii)

From (i) and (ii), 3 < r < 7.

Q 13 :
If one of the diameters of the circle $x^{2} + y^{2} - 10 x + 4 y + 13 = 0$ is a chord of another circle C, whose centre is the point of intersection of the lines 2x + 3y = 12 and 3x –2y = 5, then the radius of the circle C is : [2024]

6
4
$\sqrt{20}$
$3 \sqrt{2}$

1

2

3

4

(1)

Given, 2x + 3y = 12

3x – 2y = 5

[Figure]

Point of intersection = (3, 2)

$∴$ Centre is (3, 2)

$l = \sqrt{4 + 16} = 2 \sqrt{5}$

$l^{2} + R^{2} = r^{2}$

$\Rightarrow 20 + (25 + 4 - 13) = r^{2}$

$\Rightarrow 20 + 16 = r^{2} \Rightarrow r = 6$

Q 14 :
Let a variable line passing through the centre of the circle $x^{2} + y^{2} - 16 x - 4 y = 0$ , meet the positive co-ordinate axes at the points A and B. Then the minimum value of OA + OB, where O is the origin is equal to [2024]

18
20
12
24

1

2

3

4

(1)

Given circle is $x^{2} + y^{2} - 16 x - 4 y = 0$

Centre is (– g, – f)

Now, 2g = 16 $\Rightarrow$ g = 8 and 2f = 4 $\Rightarrow$ f = 2

$∴$ Centre is (8, 2)

[Figure]

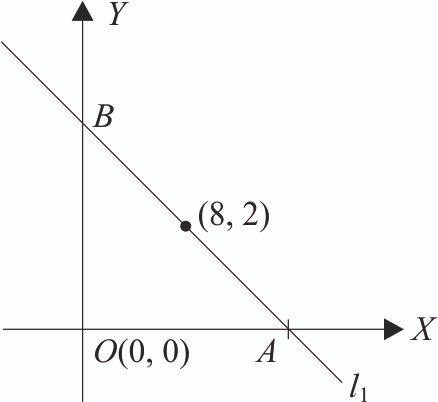
Equation of line $l_{1}$ is ... (i)

y – 2 = m(x – 8)

Equation (i) cuts the x-axis then y = 0

$\frac{- 2}{m} = x - 8 \Rightarrow x = \frac{8 m - 2}{m} = O A$

Equation (i) cuts the y-axis, then x = 0

y – 2 = – 8m $\Rightarrow$ y = 2 – 8m = OB

Let $l = O A + O B = \frac{8 m - 2}{m} + 2 - 8 m = 10 - 8 m - \frac{2}{m}$ ... (ii)

Differentiate (ii) w.r.t. m we get

$\frac{d l}{d m} = - 8 + \frac{2}{m^{2}}$ ... (iii)

$\frac{d l}{d m} = 0 \Rightarrow - 8 + \frac{2}{m^{2}} = 0 \Rightarrow m = \pm \frac{1}{2}$

Differentiate (iii), w.r.t. m, we get

$\frac{d^{2} l}{d m^{2}} = \frac{- 4}{m^{3}}$

Now, $\frac{d^{2} l}{d m^{2}}$ $|_{m = \frac{- 1}{2}} = \frac{- 4}{{(\frac{- 1}{2})}^{3}} = 32 a n d \frac{d^{2} l}{d m^{2}} |_{m = \frac{1}{2}}$ $= \frac{- 4}{{(\frac{1}{2})}^{3}} = - 32$

$∴ \frac{d^{2} l}{d m^{2}} > 0 at m = \frac{- 1}{2}$

Hence, minima occurs at $m = \frac{- 1}{2}$

So, the minimum value of $l$ is

$= 10 - 8 \times (- \frac{1}{2}) - 2 (- 2) = 10 + 4 + 4 = 18$ .

Q 15 :
Let the maximum and minimum values of ${(\sqrt{8 x - x^{2} - 12} - 4)}^{2} + {(x - 7)}^{2}, x \in R$ be M and m, respectively. Then $M^{2} - m^{2}$ is equal to __________. [2024]

0%

(1600)

Let $y = \sqrt{8 x - x^{2} - 12}$

$\Rightarrow y^{2} = 8 x - x^{2} - 12$

$= - (x^{2} - 8 x + 16) + 4 = - {(x - 4)}^{2} + 4$

$\Rightarrow {(x - 4)}^{2} + y^{2} = 4$ ... (i)

which is a circle with centre (4, 0) and radius 2.

[Figure]

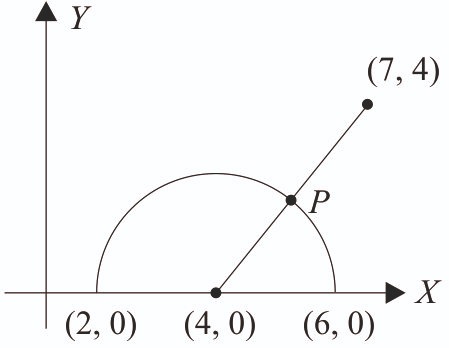
Now, ${(y - 4)}^{2} + {(x - 7)}^{2}$ represent distance of (x, y) from (7, 4)

M = Maxium distance = ${(2 - 7)}^{2} + {(0 - 4)}^{2} = 25 + 16 = 41$

m = Minimum distance = Distance between P and (7, 4)

where P is the intersection of circle with line joining (4, 0) and (7, 4).

Now, equation of line joining (4, 0) and (7, 4) is given by

$(y - 0) = \frac{4 - 0}{7 - 4} (x - 4)$

i.e., $y = \frac{4}{3} (x - 4)$

On substituting the value of y is in (i), we get

${(x - 4)}^{2} + \frac{16}{9} {(x - 4)}^{2} = 4$

$\Rightarrow {(x - 4)}^{2} [\frac{25}{9}] = 4 \Rightarrow {(x - 4)}^{2} = \frac{36}{25} \Rightarrow x - 4 = \pm \frac{6}{5}$

$\Rightarrow x = \frac{6}{5} + 4 = \frac{26}{5}, y = \frac{8}{5}$ (Neglect negative sign)

So, $m = {(\frac{26}{5} - 7)}^{2} + {(\frac{8}{5} - 4)}^{2} = \frac{81}{25} + \frac{144}{25} = \frac{225}{25} = 9$

$∴ M^{2} - m^{2} = 41^{2} - 9^{2} = 1600$ .

Q 16 :
Let the centre of a circle, passing through the points (0, 0), (1, 0) and touching the circle $x^{2} + y^{2} = 9$ , be (h, k). Then for all possible values of the coordinates of the centre (h, k), $4 (h^{2} + k^{2})$ is equal to _________. [2024]

0%

(9)

Circle will touch internally

$∴ C_{1} C_{2} = | r_{1} - r_{2} |$

$\Rightarrow \sqrt{h^{2} + k^{2}} = 3 - \sqrt{h^{2} + k^{2}} \Rightarrow 2 \sqrt{h^{2} + k^{2}} = 3$

$\Rightarrow h^{2} + k^{2} = \frac{9}{4} ∴ 4 (h^{2} + k^{2}) = 9$ .

Q 17 :
Consider a circle ${(x - α)}^{2} + {(y - β)}^{2} = 50$ , where $α, β > 0$ . If the circle touches the line y + x = 0 at the point P, whose distance from the origin is $4 \sqrt{2}$ , then ${(α + β)}^{2}$ is equal to __________. [2024]

0%

(100)

We have circle,

${(x - α)}^{2} + {(y - β)}^{2} = 50$

[Figure]

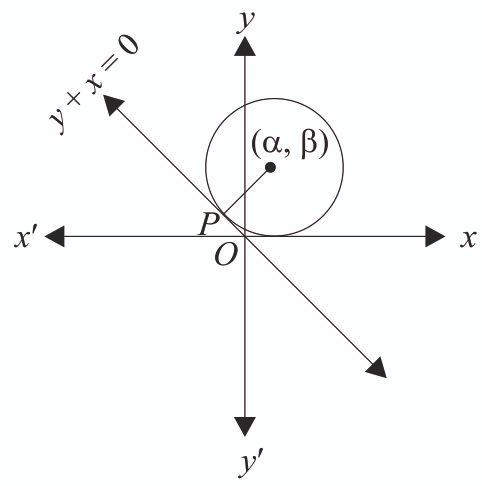
Centre of (i), is $C (α, β)$ and radius, $r = 5 \sqrt{2}$

$∴ C P = r$

$\Rightarrow$ $| \frac{α + β}{\sqrt{2}} |$ $= 5 \sqrt{2}$

$\Rightarrow | α + β | = 10 \Rightarrow {(α + β)}^{2} = 100$ .

Q 18 :
Equations of two diameters of a circle are 2x – 3y = 5 and 3x – 4y = 7. The line joining the points $(- \frac{22}{7}, - 4)$ and $(- \frac{1}{7}, 3)$ intersects the circle at only one point $P (α, β)$ . Then $17 β - α$ is equal to __________. [2024]

0%

(2)

Solving 2x – 3y = 5 and 3x – 4y = 7, we get x = 1 and y = –1

[Figure]

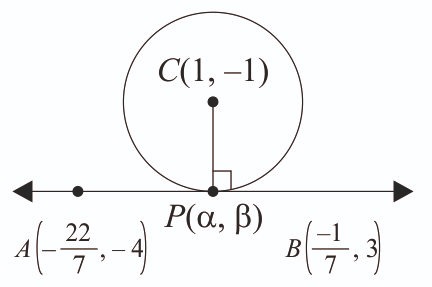
Now, equation of tangent joining the points $(- \frac{22}{7}, - 4)$ and $(- \frac{1}{7}, 3)$ is

$y + 4 = \frac{3 + 4}{\frac{- 1}{7} + \frac{22}{7}} (x + \frac{22}{7}) \Rightarrow 3 (y + 4) = 7 (x + \frac{22}{7})$

$\Rightarrow 7 x - 3 y + 10 = 0$

$α, β$ be the foot of perpendicular from point (1, –1) to the line 7x – 3y + 10 = 0.

$∴ \frac{α - 1}{7} = \frac{β + 1}{- 3} = \frac{- (7 (1) - 3 (- 1) + 10)}{{(- 3)}^{2} + 7^{2}} = - \frac{20}{58} = \frac{- 10}{29}$

$∴ α = \frac{- 70}{29} + 1 = \frac{- 41}{29}, β = \frac{30}{29} - 1 = \frac{1}{29}$

$∴ 17 β - α = \frac{17}{29} + \frac{41}{29} = \frac{58}{29} = 2$ .

Q 19 :
Consider two circles $C_{1} : x^{2} + y^{2} = 25$ and $C_{2} : {(x - α)}^{2} + y^{2} = 16$ , where $α \in (5, 9)$ . Let the angle between the two radii (one to each circle) drawn from one of the intersection points of $C_{1}$ and $C_{2}$ be $\sin^{- 1} (\frac{\sqrt{63}}{8})$ . If the length of common chord of $C_{1}$ and $C_{2}$ is $β$ , then the value of ${(α β)}^{2}$ equals __________. [2024]

0%

(1575)

We have,

$C_{1} : x^{2} + y^{2} = 25$ or $C_{2} : {(x - α)}^{2} + y^{2} = 16$

[figure]

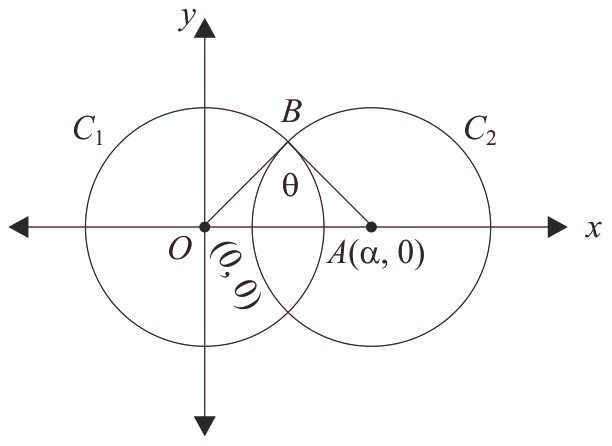
Let $θ$ be the angle between two radii

$∴ θ = \sin^{- 1} \frac{\sqrt{63}}{8}$

$\Rightarrow \sin θ = \frac{\sqrt{63}}{8}$

Area of $△ O A B = \frac{1}{2} \times 4 \times 5 \sin θ$

$\Rightarrow \frac{1}{2} \times α \times \frac{β}{2} = 10 \frac{\sqrt{63}}{8} \Rightarrow α β = 5 \sqrt{63} \Rightarrow {(α β)}^{2} = 1575$ .

Topic Question Set

Q 11 :
Four distinct points (2k, 3k), (1, 0), (0, 1) and (0, 0) lie on a circle for k equal to : [2024]

Q 12 :
If the circles ${(x + 1)}^{2} + {(y + 2)}^{2} = r^{2}$ and $x^{2} + y^{2} - 4 x - 4 y + 4 = 0$ intersect at exactly two distinct points, then [2024]

Q 13 :
If one of the diameters of the circle $x^{2} + y^{2} - 10 x + 4 y + 13 = 0$ is a chord of another circle C, whose centre is the point of intersection of the lines 2x + 3y = 12 and 3x –2y = 5, then the radius of the circle C is : [2024]

Q 14 :
Let a variable line passing through the centre of the circle $x^{2} + y^{2} - 16 x - 4 y = 0$ , meet the positive co-ordinate axes at the points A and B. Then the minimum value of OA + OB, where O is the origin is equal to [2024]

Q 15 :
Let the maximum and minimum values of ${(\sqrt{8 x - x^{2} - 12} - 4)}^{2} + {(x - 7)}^{2}, x \in R$ be M and m, respectively. Then $M^{2} - m^{2}$ is equal to __________. [2024]

0%

Q 16 :
Let the centre of a circle, passing through the points (0, 0), (1, 0) and touching the circle $x^{2} + y^{2} = 9$ , be (h, k). Then for all possible values of the coordinates of the centre (h, k), $4 (h^{2} + k^{2})$ is equal to _________. [2024]

0%

Q 17 :
Consider a circle ${(x - α)}^{2} + {(y - β)}^{2} = 50$ , where $α, β > 0$ . If the circle touches the line y + x = 0 at the point P, whose distance from the origin is $4 \sqrt{2}$ , then ${(α + β)}^{2}$ is equal to __________. [2024]

0%

Q 18 :
Equations of two diameters of a circle are 2x – 3y = 5 and 3x – 4y = 7. The line joining the points $(- \frac{22}{7}, - 4)$ and $(- \frac{1}{7}, 3)$ intersects the circle at only one point $P (α, β)$ . Then $17 β - α$ is equal to __________. [2024]

0%

0%

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 11 : Four distinct points (2k, 3k), (1, 0), (0, 1) and (0, 0) lie on a circle for k equal to : [2024]

Q 12 : If the circles (x+1)2+(y+2)2=r2 and x2+y2–4x–4y+4=0 intersect at exactly two distinct points, then [2024]

Q 13 : If one of the diameters of the circle x2+y2–10x+4y+13=0 is a chord of another circle C, whose centre is the point of intersection of the lines 2x + 3y = 12 and 3x –2y = 5, then the radius of the circle C is : [2024]

Q 14 : Let a variable line passing through the centre of the circle x2+y2–16x–4y=0, meet the positive co-ordinate axes at the points A and B. Then the minimum value of OA + OB, where O is the origin is equal to [2024]

Q 15 : Let the maximum and minimum values of (8x –x2–12 –4)2+(x-7)2, x∈R be M and m, respectively. Then M2–m2 is equal to __________. [2024]

0%

Q 16 : Let the centre of a circle, passing through the points (0, 0), (1, 0) and touching the circle x2+y2=9, be (h, k). Then for all possible values of the coordinates of the centre (h, k), 4(h2+k2) is equal to _________. [2024]

0%

Q 17 : Consider a circle (x–α)2+(y–β)2=50, where α,β>0. If the circle touches the line y + x = 0 at the point P, whose distance from the origin is 42, then (α+β)2 is equal to __________. [2024]

0%

Q 18 : Equations of two diameters of a circle are 2x – 3y = 5 and 3x – 4y = 7. The line joining the points (–227,–4) and (–17,3) intersects the circle at only one point P(α,β). Then 17β–α is equal to __________. [2024]

0%

0%

Want Us to Email you About Special Offers & Updates?

Q 11 :
Four distinct points (2k, 3k), (1, 0), (0, 1) and (0, 0) lie on a circle for k equal to : [2024]

Q 12 :
If the circles ${(x + 1)}^{2} + {(y + 2)}^{2} = r^{2}$ and $x^{2} + y^{2} - 4 x - 4 y + 4 = 0$ intersect at exactly two distinct points, then [2024]

Q 13 :
If one of the diameters of the circle $x^{2} + y^{2} - 10 x + 4 y + 13 = 0$ is a chord of another circle C, whose centre is the point of intersection of the lines 2x + 3y = 12 and 3x –2y = 5, then the radius of the circle C is : [2024]

Q 14 :
Let a variable line passing through the centre of the circle $x^{2} + y^{2} - 16 x - 4 y = 0$ , meet the positive co-ordinate axes at the points A and B. Then the minimum value of OA + OB, where O is the origin is equal to [2024]

Q 15 :
Let the maximum and minimum values of ${(\sqrt{8 x - x^{2} - 12} - 4)}^{2} + {(x - 7)}^{2}, x \in R$ be M and m, respectively. Then $M^{2} - m^{2}$ is equal to __________. [2024]

Q 16 :
Let the centre of a circle, passing through the points (0, 0), (1, 0) and touching the circle $x^{2} + y^{2} = 9$ , be (h, k). Then for all possible values of the coordinates of the centre (h, k), $4 (h^{2} + k^{2})$ is equal to _________. [2024]

Q 17 :
Consider a circle ${(x - α)}^{2} + {(y - β)}^{2} = 50$ , where $α, β > 0$ . If the circle touches the line y + x = 0 at the point P, whose distance from the origin is $4 \sqrt{2}$ , then ${(α + β)}^{2}$ is equal to __________. [2024]

Q 18 :
Equations of two diameters of a circle are 2x – 3y = 5 and 3x – 4y = 7. The line joining the points $(- \frac{22}{7}, - 4)$ and $(- \frac{1}{7}, 3)$ intersects the circle at only one point $P (α, β)$ . Then $17 β - α$ is equal to __________. [2024]