Two Dimensional Geometry jee mains questions | JEE Main Two Dimensional Geometry Previous Year Question

Q 1 :
Consider a hyperbola H having centre at the origin and foci on the x-axis. Let $C_{1}$ be the circle touching the hyperbola H and having the centre at the origin. Let $C_{2}$ be the circle touching the hyperbola H at its vertex and having the centre at one of its foci. If areas (in sq. units) of $C_{1}$ and $C_{2}$ are 36 $π$ and 4 $π$ , respectively, then the length (in units) of latus rectum of H is [2024]

$\frac{28}{3}$
$\frac{14}{3}$
$\frac{10}{3}$
$\frac{11}{3}$

1

2

3

4

(1)

$C_{1} : x^{2} + y^{2} = a^{2}$

$\Rightarrow Area = {πa}^{2} = 36 π \Rightarrow a = 6$

$C_{2} : {(x - a e)}^{2} + y^{2} = {(a e - a)}^{2}$

$\Rightarrow Area = π {(a e - a)}^{2} = 4 π \Rightarrow 36 {(e - 1)}^{2} = 4$

$\Rightarrow e - 1 = \frac{1}{3} \Rightarrow e = \frac{4}{3} \Rightarrow b^{2} = 28$

$∴$ Length of Latus Rectum = $\frac{2 b^{2}}{a} = \frac{2 \times 28}{6} = \frac{28}{3}$ units.

Q 2 :
Let $H : \frac{- x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ be the hyperbola, whose eccentricity is $\sqrt{3}$ and the length of the latus rectum is $4 \sqrt{3}$ . Suppose the point ( $α$ , 6), $α$ > 0 lies on H. If $β$ is the product of the focal distances of the point ( $α$ , 6), then $α^{2} + β$ is equal to [2024]

169
171
172
170

1

2

3

4

(2)

$H : \frac{y^{2}}{b^{2}} - \frac{x^{2}}{a^{2}} = 1$ and $e = \sqrt{3}$

Now, $e = \sqrt{1 + \frac{a^{2}}{b^{2}}} = \sqrt{3}$

$\Rightarrow \frac{a^{2}}{b^{2}} = 2 \Rightarrow a^{2} = 2 b^{2}$

Length of latus rectum $= \frac{2 a^{2}}{b} = \frac{4 b^{2}}{b} = 4 b = 4 \sqrt{3}$ [Given]

$\Rightarrow b = \sqrt{3} \Rightarrow a = \sqrt{6}$ [ $∵$ a > 0]

Now, P( $α$ , 6) lies on $H : \frac{y^{2}}{3} - \frac{x^{2}}{6} = 1$

$\Rightarrow 12 - \frac{α^{2}}{6} = 1 \Rightarrow α^{2} = 66$

Now, foci of hyperbola = (0, $\pm$ be) = (0, $\pm$ 3)

Let $d_{1}$ and $d_{2}$ be focal distancee of ( $α$ , 6)

$\Rightarrow d_{1} = \sqrt{α^{2} + {(6 - 3)}^{2}}, d_{2} = \sqrt{α^{2} + {(6 + 3)}^{2}}$

$\Rightarrow d_{1} = \sqrt{66 + 9}, d_{2} = \sqrt{66 + 81}$

$d_{1} = \sqrt{75}, d_{2} = \sqrt{147}$

Now, $β = d_{1} d_{2} = \sqrt{11025} = 105$

Now, $α^{2} + β = 66 + 105 = 171$ .

Q 3 :
Let the foci of a hypebola H coincide with the foci of the ellipse $E : \frac{{(x - 1)}^{2}}{100} + \frac{{(y - 1)}^{2}}{75} = 1$ and the eccentriticy of the hyperbola H be the reciprocal of the eccentricity of the ellipse E. If the length of the transverse axis of H is $α$ and the length of its conjugate axis is $β$ , then $3 α^{2} + 2 β^{2}$ is equal to [2024]

237
225
205
242

1

2

3

4

(2)

Eccentricity of ellipse E i.e., $e_{E} = \sqrt{1 - \frac{b^{2}}{a^{2}}}$

$= \sqrt{1 - \frac{75}{100}} = \sqrt{\frac{25}{100}} = \frac{1}{2}$

So, eccentricity of hyperbola = 2

Now, foci of ellipse = (1 $\pm$ ae, 1) = (1 $\pm$ 5, 1)

= (6, 1) and (–4, 1) = Foci of hyperbola

Now, distance between foci = $2 a e_{H}$

$\Rightarrow \sqrt{10^{2} + 0} = 4 a \Rightarrow a = \frac{5}{2}$

Also, $e_{H}^{2} = 1 + \frac{b^{2}}{a^{2}}$

$\Rightarrow {(2)}^{2} = 1 + \frac{4 b^{2}}{25} \Rightarrow \frac{75}{4} = b^{2} \Rightarrow b = \frac{\sqrt{75}}{2}$

Length of transverse axis of H = 2a = $2 \times \frac{5}{2}$

$\Rightarrow α = 5$

Length of conjugate axis of H = 2b = $\sqrt{75}$

$\Rightarrow β = \sqrt{75}$

Now, $3 α^{2} + 2 β^{2} = 75 + 150 = 225$ .

Q 4 :
For 0 < $θ$ < $π$ /2, if the eccentricity of the hyperbola $x^{2} - y^{2} {cosec}^{2} θ = 5$ is $\sqrt{7}$ times eccentricity of the ellipse $x^{2} {cosec}^{2} θ + y^{2} = 5$ , then the value of $θ$ is [2024]

$π / 6$
$π / 3$
$5 π / 12$
$π / 4$

1

2

3

4

(2)

Given, $\frac{x^{2}}{5} - \frac{y^{2}}{5 \sin^{2} θ} = 1$

$a = \sqrt{5} > b = \sqrt{5} \sin θ$

$e = \sqrt{1 + \frac{b^{2}}{a^{2}}} = \sqrt{1 + \sin^{2} θ}$ ... (i)

Also, $\frac{x^{2}}{5 \sin^{2} θ} + \frac{y^{2}}{5} = 1$ (Given)

$a = \sqrt{5} \sin θ < b = \sqrt{5}$

$e = \sqrt{1 - \frac{a^{2}}{b^{2}}} = \sqrt{1 - \sin^{2} θ}$ ... (ii)

Given, eqn. (i) = $\sqrt{7}$ eqn. (ii)

$\sqrt{1 + \sin^{2} θ} = \sqrt{7} \sqrt{1 - \sin^{2} θ}$

Squaring on both sides.

$1 + \sin^{2} θ = 7 (1 - \sin^{2} θ) \Rightarrow 1 + \sin^{2} θ = 7 - 7 \sin^{2} θ$

$\Rightarrow 8 \sin^{2} θ = 6$

$\sin^{2} θ = \frac{6}{8} = \frac{3}{4} \Rightarrow \sin θ = \frac{\sqrt{3}}{2}$

So, $θ = \frac{π}{3}$ .

Q 5 :
Let $e_{1}$ be the eccentricity of the hyperbola $\frac{x^{2}}{16} - \frac{y^{2}}{9} = 1$ and $e_{2}$ be the eccentricity of the ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ , a > b, which passes through the foci of the hyperbola. If $e_{1} e_{2} = 1$ , then the length of the chord of the ellipse parallel to the x-axis and passing through (0, 2) is [2024]

$4 \sqrt{5}$
$\frac{10 \sqrt{5}}{3}$
$\frac{8 \sqrt{5}}{3}$
$3 \sqrt{5}$

1

2

3

4

(2)

Given hyperbola is $\frac{x^{2}}{16} - \frac{y^{2}}{9} = 1$

$e_{1}$ = eccentricity of hyperbola = $\frac{\sqrt{16 + 9}}{4} = \frac{5}{4}$

Foci of hyperbola = ( $\pm$ 5, 0)

Given $e_{1} e_{2} = 1$

$\Rightarrow e_{2} = \frac{4}{5}$ = eccentricity of ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$

$\Rightarrow$ $\frac{\sqrt{a^{2} - b^{2}}}{a} = \frac{4}{5}$ ... (i)

$∴$ The ellipse also passes through the foci of the hyperbola.

$\Rightarrow a^{2} = 25$

From (i), $b^{2} = 9$

Thus, the equation of ellipse is $\frac{x^{2}}{25} + \frac{y^{2}}{9} = 1$ ... (ii)

Now, equation of chord of ellipse which passes through (0, 2) and parallel to x-axis is y = 2.

From (ii),

$\frac{x^{2}}{25} = \frac{5}{9} \Rightarrow x^{2} = \frac{25 \times 5}{9} \Rightarrow x = \pm \frac{5 \sqrt{5}}{3}$

Thus, the end point of chord are $(\frac{- 5 \sqrt{5}}{3}, 2) and (\frac{5 \sqrt{5}}{3}, 2)$ .

$∴$ Length of chord = $\sqrt{{(\frac{5 \sqrt{5}}{3} + \frac{5 \sqrt{5}}{3})}^{2} + {(2 - 2)}^{2}} = \frac{10 \sqrt{5}}{3}$ .

Q 6 :
Let P be a point on the hyperbola $H : \frac{x^{2}}{9} - \frac{y^{2}}{4} = 1$ in the first quadrant such that the area of triangle formed by P and the two foci of H is $2 \sqrt{13}$ . Then, the square of the distance of P from the origin is [2024]

20
18
26
22

1

2

3

4

(4)

We have, $\frac{x^{2}}{9} - \frac{y^{2}}{4} = 1$

Here, $a^{2} = 9, b^{2} = 4$

$b^{2} = a^{2} (e^{2} - 1) \Rightarrow e^{2} = 1 + \frac{b^{2}}{a^{2}} \Rightarrow e^{2} = 1 + \frac{4}{9} = \frac{13}{9}$

$\Rightarrow e = \frac{\sqrt{13}}{3} \Rightarrow s_{1} s_{2} = 2 a e = 2 \times 3 \times \frac{\sqrt{13}}{3} = 2 \sqrt{13}$

Area of $△ P S_{1} S_{2} = \frac{1}{2} \times β \times s_{1} s_{2} = 2 \sqrt{13}$

$\Rightarrow \frac{1}{2} \times β \times (2 \sqrt{13}) = 2 \sqrt{13} \Rightarrow β = 2$

$\frac{α^{2}}{9} - \frac{β^{2}}{4} = 1 \Rightarrow \frac{α^{2}}{9} = 2 \Rightarrow α^{2} = 18 \Rightarrow α = 3 \sqrt{2}$

Distance of P from origin = $\sqrt{α^{2} + β^{2}} = \sqrt{18 + 4} = \sqrt{22}$ .

Q 7 :
If the foci of a hyperbola are same as that of the ellipse $\frac{x^{2}}{9} + \frac{y^{2}}{25} = 1$ and the eccentricity of the hyperbola is $\frac{15}{8}$ times the eccentricity of the ellipse, then the smaller focal distance of the point $(\sqrt{2}, \frac{14}{3} \sqrt{\frac{2}{5}})$ on the hyperbola, is equal to [2024]

$7 \sqrt{\frac{2}{5}} - \frac{8}{3}$
$14 \sqrt{\frac{2}{5}} - \frac{16}{5}$
$14 \sqrt{\frac{2}{5}} - \frac{4}{3}$
$7 \sqrt{\frac{2}{5}} + \frac{8}{3}$

1

2

3

4

(1)

We have, $E : \frac{x^{2}}{9} + \frac{y^{2}}{25} = 1$

$a = 5, b = 3$

foci : (0, $\pm$ 5e)

Since, for an ellipes, $b^{2} = a^{2} (1 - e^{2}) \Rightarrow 9 = 25 (1 - e^{2})$

$e^{2} = 1 - \frac{9}{25} = \frac{16}{25} \Rightarrow e = \frac{4}{5}$

Eccentricity of hyperbola = $e' = \frac{15}{8} \times \frac{4}{5} = \frac{3}{2}$

Focus of hyperbola = (0, $\pm$ 5e) = (0, $\pm$ 4)

$\Rightarrow a \cdot e' = 4 \Rightarrow a = 4 \times \frac{2}{3} = \frac{8}{3}$

Since, $e'^{2} = 1 + \frac{b^{2}}{a^{2}} \Rightarrow \frac{9}{4} = 1 + \frac{b^{2}}{1} \times \frac{9}{64} \Rightarrow b^{2} = \frac{80}{9}$

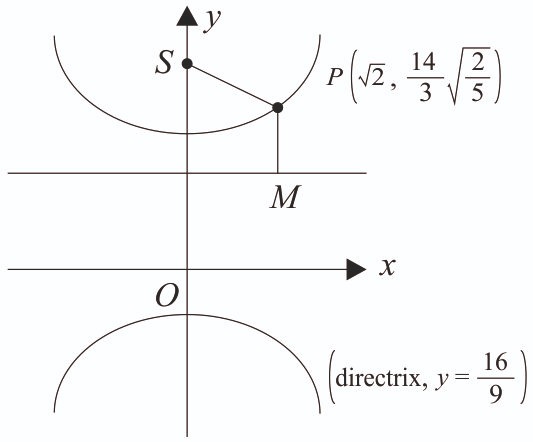
$∴$ The equation of hyperbola $\frac{9 y^{2}}{64} - \frac{9 x^{2}}{80} = 1$

PS = $e' p M$

$= \frac{3}{2} \times (\frac{14}{3} \sqrt{\frac{2}{5}} - \frac{16}{9})$

$= 7 \sqrt{\frac{2}{5}} - \frac{8}{3}$ .

Q 8 :
Let A be a square matrix of order 2 such that |A| = 2 and the sum of its diagonal elements is –3. If the points (x, y) satisfying $A^{2}$ + xA + y $I$ = O lie on a hyperbola, whose transverse axis is parallel to the x-axis, eccentricity is e and the length of the latus rectum is $l$ , then $e^{4} + l^{4}$ is equal to ___________. [2024]

0%

(*)

The given data is inadequate.

Q 9 :
The length of the latus rectum and directrices of a hyperbola with eccentricity e are 9 and $x = \pm \frac{4}{\sqrt{3}}$ , respectively. Let the line $y - \sqrt{3} x + \sqrt{3} = 0$ touch this hyperbola at $(x_{0}, y_{0})$ . If m is the product of the focal distances of the point $(x_{0}, y_{0})$ , then $4 e^{2} + m$ is equal to __________. [2024]

0%

(*)

Equation of tangent to hyperbola is given by $y = m x \pm \sqrt{a^{2} m^{2} - b^{2}}$

$\Rightarrow m = \sqrt{3}$

and $a^{2} m^{2} - b^{2} = 3$

$\Rightarrow 3 a^{2} - b^{2} = 3$ ... (i)

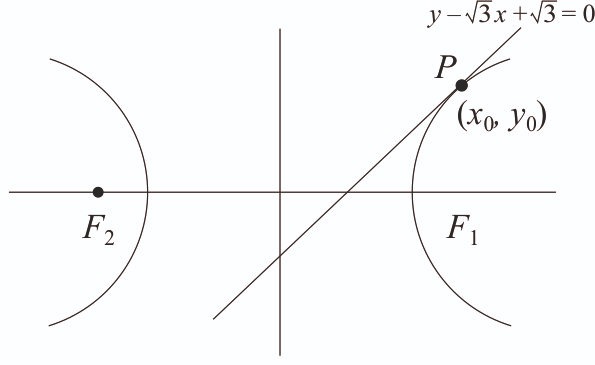
Now, length of latus rectum of hyperbola = 9

$\Rightarrow \frac{2 b^{2}}{a} = 9$

$\Rightarrow b^{2} = \frac{9 a}{2}$ ... (ii)

From equation (i) and (ii), we get

$3 a^{2} - \frac{9 a}{2} = 3$

$\Rightarrow 6 a^{2} - 9 a - 6 = 0 \Rightarrow 2 a^{2} - 3 a - 2 = 0$

$\Rightarrow 2 a^{2} - 4 a + a - 2 = 0 \Rightarrow (2 a + 1) (a - 2) = 0$

$\Rightarrow a = 2, \frac{- 1}{2} (ignore) \Rightarrow a = 2 and b = 3$

Equation of hyperbola is $\frac{x^{2}}{4} - \frac{y^{2}}{9} = 1$

Solving for tangent $y = \sqrt{3} x - \sqrt{3}$ , we get

$\frac{x^{2}}{4} - \frac{3 x^{2} + 3 - 6 x}{9} = 1$

$\Rightarrow 9 x^{2} - 12 x^{2} - 12 + 24 x = 36$

$\Rightarrow 3 x^{2} - 24 x + 48 = 0$

$\Rightarrow x^{2} - 8 x + 16 = 0 \Rightarrow {(x - 4)}^{2} = 0$

$\Rightarrow x = 4 and y = 3 \sqrt{3}, e = \sqrt{1 + \frac{9}{4}} = \frac{\sqrt{13}}{2}$

So, $(x_{0}, y_{0}) \equiv (4, 3 \sqrt{3})$ is the point of contact.

Focus of hyperbola is $(\pm \sqrt{13}, 0)$

$∴ P F_{1} \cdot P F_{2}$

$= (\sqrt{{(4 - \sqrt{13})}^{2} + {(3 \sqrt{3})}^{2}}) (\sqrt{{(4 + \sqrt{13})}^{2} + {(3 \sqrt{3})}^{2}})$

$= \sqrt{2304} = 48 = m$

$∴ 4 e^{2} + m = 4 \times 13 / 4 + 48 = 61$

Note: Here equation of directrix should be $x = \pm \frac{4}{\sqrt{13}}$ , but in given question it is given $x = \pm \frac{4}{\sqrt{3}}$ which is wrong because eccentricity should be greater than 1, So, this question is bonus.

Q 10 :
Let S be the focus of the hyperbola $\frac{x^{2}}{3} - \frac{y^{2}}{5} = 1$ , on the positive x-axis. Let C be the circle with its centre at $A (\sqrt{6}, \sqrt{5})$ and passing through the point S. If O is the origin and SAB is a diameter of C, then the square of the area of the triangle OSB is equal to __________. [2024]

0%

(40)

$\frac{x^{3}}{3} - \frac{y^{2}}{5} = 1 \Rightarrow a = \sqrt{3}, b = \sqrt{5}$

$∴ e = \sqrt{1 + \frac{5}{3}} \Rightarrow e = \sqrt{\frac{8}{3}}$

$∴ a e = \sqrt{8} = 2 \sqrt{2} ∴ S = (2 \sqrt{2}, 0)$

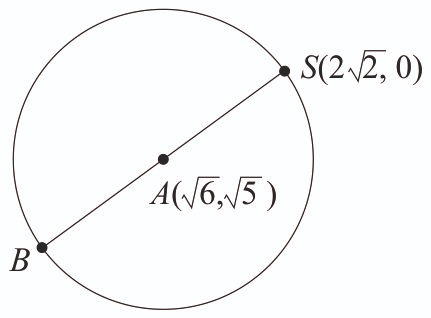
A is mid-point of BS $\Rightarrow B (2 \sqrt{6} - 2 \sqrt{2}, 2 \sqrt{5)}$

Area of $△ (O S B)$ , $A =$ $|$ $\frac{1}{2}$ $| \begin{matrix} 0 & 0 & 1 \\ 2 \sqrt{2} & 0 & 1 \\ 2 \sqrt{6} - 2 \sqrt{2} & 2 \sqrt{5} & 1 \end{matrix} |$ $|$ $= 2 \sqrt{10}$

$\Rightarrow A^{2} = 40$ .

Topic Question Set

Q 4 :
For 0 < $θ$ < $π$ /2, if the eccentricity of the hyperbola $x^{2} - y^{2} {cosec}^{2} θ = 5$ is $\sqrt{7}$ times eccentricity of the ellipse $x^{2} {cosec}^{2} θ + y^{2} = 5$ , then the value of $θ$ is [2024]

Q 6 :
Let P be a point on the hyperbola $H : \frac{x^{2}}{9} - \frac{y^{2}}{4} = 1$ in the first quadrant such that the area of triangle formed by P and the two foci of H is $2 \sqrt{13}$ . Then, the square of the distance of P from the origin is [2024]

0%

0%

0%

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 2 : Let H : –x2a2 + y2b2 = 1 be the hyperbola, whose eccentricity is 3 and the length of the latus rectum is 43. Suppose the point (α, 6), α > 0 lies on H. If β is the product of the focal distances of the point (α, 6), then α2 + β is equal to [2024]

Q 4 : For 0 < θ < π/2, if the eccentricity of the hyperbola x2–y2 cosec2 θ=5 is 7 times eccentricity of the ellipse x2 cosec2 θ + y2=5, then the value of θ is [2024]

Q 5 : Let e1 be the eccentricity of the hyperbola x216–y29=1 and e2 be the eccentricity of the ellipse x2a2+y2b2=1, a > b, which passes through the foci of the hyperbola. If e1e2=1, then the length of the chord of the ellipse parallel to the x-axis and passing through (0, 2) is [2024]

Q 6 : Let P be a point on the hyperbola H : x29–y24=1 in the first quadrant such that the area of triangle formed by P and the two foci of H is 213. Then, the square of the distance of P from the origin is [2024]

Q 7 : If the foci of a hyperbola are same as that of the ellipse x29+y225=1 and the eccentricity of the hyperbola is 158 times the eccentricity of the ellipse, then the smaller focal distance of the point (2,14325) on the hyperbola, is equal to [2024]

0%

Q 9 : The length of the latus rectum and directrices of a hyperbola with eccentricity e are 9 and x=±43, respectively. Let the line y–3x+3=0 touch this hyperbola at (x0, y0). If m is the product of the focal distances of the point (x0, y0), then 4e2+m is equal to __________. [2024]

0%

Q 10 : Let S be the focus of the hyperbola x23–y25=1, on the positive x-axis. Let C be the circle with its centre at A(6,5) and passing through the point S. If O is the origin and SAB is a diameter of C, then the square of the area of the triangle OSB is equal to __________. [2024]

0%

Want Us to Email you About Special Offers & Updates?

Q 4 :
For 0 < $θ$ < $π$ /2, if the eccentricity of the hyperbola $x^{2} - y^{2} {cosec}^{2} θ = 5$ is $\sqrt{7}$ times eccentricity of the ellipse $x^{2} {cosec}^{2} θ + y^{2} = 5$ , then the value of $θ$ is [2024]

Q 6 :
Let P be a point on the hyperbola $H : \frac{x^{2}}{9} - \frac{y^{2}}{4} = 1$ in the first quadrant such that the area of triangle formed by P and the two foci of H is $2 \sqrt{13}$ . Then, the square of the distance of P from the origin is [2024]