Q 1 :    

In a coil, the current changes from $-2A$ to $+2A$ in 0.2 s and induces an emf 0.1 V. The self-inductance of the coil is:               [2024]

• 4 mH

   

• 5 mH

   

• 2.5 mH

   

• 1 MH

   

Q 2 :    

The current in an inductor is given by $I=(3t+8)$ where $t$ is in second. The magnitude of induced emf produced in the inductor is 12 mV. The self-inductance of the inductor ________ mH.                 [2024]

Q 3 :    

Two coils have mutual inductance 0.002H. The current changes in the first coil according to the relation $i=i_0\sin \omega t$, where $i_0=5A$ and $\omega =50\pi$ rad/s. The maximum value of emf in the second coil is $\frac{\pi }{\alpha }$ V. The value of $\alpha$ is _____.                              [2024]

Q 4 :    

Two conducting circular loops A and B are placed in the same plane with their centres coinciding as shown in figure. The mutual inductance between them is           [2024]

• $\frac{{\mu }_0\pi b^2}{2a}$

   

• $\frac{{\mu }_0\pi a^2}{2b}$

   

• $\frac{{\mu }_0}{2\pi }·\frac{b^2}{a}$

   

• $\frac{{\mu }_0}{2\pi }·\frac{a^2}{b}$

   

Q 5 :    

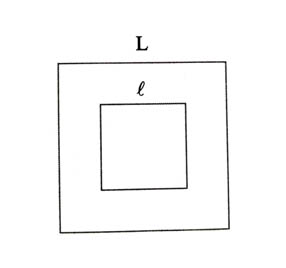
A small square loop of wire of side L is placed inside a large square loop of wire of side $L(L=l^2)$. The loops are coplanar and their centers coincide. The value of the mutual inductance of the system is $\sqrt{x}\times {10}^{-7}$H, where $x=$ ____.                  [2024]

Q 6 :    

Regarding self-inductance:

A. The self-inductance of the coil depends on its geometry.

B. Self-inductance does not depend on the permeability of the medium.

C. Self-induced e.m.f. opposes any change in the current in a circuit.

D. Self-inductance is electromagnetic analogue of mass in mechanics.

E. Work needs to be done against self-induced e.m.f. in establishing the current.

Choose the correct answer from the options given below:          [2025]

• A, B, C, D only

   

• A, C, D, E only

   

• A, B, C, E only

   

• B, C, D, E only

   

Q 7 :    

Consider $I_1$ and $I_2$ are he currents flowing simultaneously in two nearby coils 1 and 2, respectively. If $L_1$ = self-inductance of coil 1, $M_{12}$ = mutual inductance of coil 1 with respect to coil 2, then the value of induced emf in coil 1 will be          [2025]

• ${\epsilon }_1=–L_1\frac{d{I}_1}{dt}+M_{12}\frac{d{I}_2}{dt}$

   

• ${\epsilon }_1=–L_1\frac{d{I}_1}{dt}–M_{12}\frac{d{I}_1}{dt}$

   

• ${\epsilon }_1=–L_1\frac{d{I}_1}{dt}–M_{12}\frac{d{I}_2}{dt}$

   

• ${\epsilon }_1=–L_1\frac{d{I}_2}{dt}+M_{12}\frac{d{I}_1}{dt}$