Topic Question Set
Q 1
:
A sample of gas at temperature T is adiabatically expanded to double its volume. Adiabatic constant for the gas is The work done by the gas in the process is (n = 1 mole) [2024]
(4)
Also, constant
constant constant
Q 2
:
During an adiabatic process, if the pressure of a gas is found to be proportional to the cube of its absolute temperature, then the ratio of for the gas is [2024]
(1)
constant
constant from ideal gas equation
constant constant
constant ... (1)
Process equation for adiabatic process is
constant ... (2)
Comparing equation (1) and (2)
Q 3
:
A total of 48 J heat is given to one mole of helium kept in a cylinder. The temperature of helium increases by 2°C. The work done by the gas is (Given: ) [2024]
48 J
23.1 J
24.9 J
72.9 J
(2)
Given :
law of thermodynamics,
Q 4
:
A diatomic gas does 100 J of work in an isobaric expansion. The heat given to the gas is [2024]
150 J
250 J
490 J
350 J
(4)
work done (W)
Q 5
:
The volume of an ideal gas is changed adiabatically from 5 litres to 4 litres. The ratio of initial pressure to final pressure is: [2024]
(3)
For adiabatic process,
Q 6
:
A sample of 1 mole gas at temperature T is adiabatically expanded to double its volume. If adiabatic constant for the gas is , then the work done by the gas in the process is: [2024]
(1)
WD by gas in adiabatic process
Q 7
:
During an adiabatic process, the pressure of a gas is found to be proportional to the cube of its absolute temperature. The ratio of for the gas is [2024]
(2)
constant
const
const
const const
Q 8
:
The pressure and volume of an ideal gas are related as (Constant). The work done when the gas is taken from state to state is [2024]
(1)
For constant
Here
Q 9
:
A diatomic gas does 200 J of work when it is expanded isobarically. The heat given to the gas in the process is [2024]
850 J
800 J
600 J
700 J
(4)
Q 10
:
The heat absorbed by a system in going through the given cyclic process is: [2024]
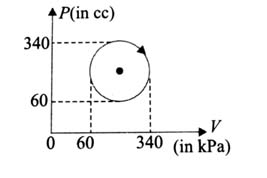

431.2 J
616 J
61.6 J
19.6 J
(3)

