Electric Potential and Capacitance | Physics JEE previous year questions with Solutions

Q 1 :

A parallel plate capacitor of capacitance 12.5 pF is charged by a battery connected between its plates to potential difference of 12.0 V. The battery is now disconnected and a dielectric slab $(ε_{r} = 6)$ is inserted between the plates. The change in its potential energy after inserting the dielectric slab is _______ $\times 10^{- 12} J .$ [2024]

(750) Before inserting dielectric, capacitance is given as $C_{0} = 12.5$ pF and charge on the capacitor is $Q = C_{0} V$ . After inserting dielectric capacitance will become $C_{f} = ε_{r} C_{0} .$

Change in potential energy of the capacitor

$∆ U = U_{0} - U_{f} = \frac{Q^{2}}{2 C_{0}} - \frac{Q^{2}}{2 C_{f}} = \frac{Q^{2}}{2 C_{0}} [1 - \frac{1}{ε_{r}}]$

$∆ U = \frac{{(C_{0} V)}^{2}}{2 C_{0}} [1 - \frac{1}{ε_{r}}] = \frac{1}{2} C_{0} V^{2} [1 - \frac{1}{ε_{r}}]$

Using $C_{0} = 12.5 p F,$ $V = 12$ $V,$ $ε_{r} = 6$

$∆ U = \frac{1}{2} (12.5) \times 12^{2} [1 - \frac{1}{6}] = \frac{1}{2} (12.5) \times 12^{2} \times \frac{5}{6}$

$= 750 p J = 750 \times 10^{- 12} J$

Q 2 :

A parallel plate capacitor with plate separation 5mm is charged up by a battery. It is found that on introducing a dielectric sheet of thickness 2 mm, while keeping the battery connections intact, the capacitor draws 25% more charge from the battery than before. The dielectric constant of the sheet is __________. [2024]

(2) Without dielectric $Q = \frac{A \in_{0}}{d} V$

with dielectric

$Q = \frac{A \in_{0} V}{d - t + \frac{t}{K}}$

Given $\frac{A \in_{0} V}{d - t + \frac{t}{K}} = (1.25) \frac{A \in_{0} V}{d}$

$\Rightarrow 1.25 (3 + \frac{2}{K}) = 5 \Rightarrow K = 2$

Q 3 :

A capacitor has air as dielectric medium and two conducting plates of area 12 ${cm}^{2}$ and they are 0.6 cm apart. When a slab of dielectric having area 12 ${cm}^{2}$ and 0.6 cm thickness is inserted between the plates, one of the conducting plates has to be moved by 0.2 cm to keep the capacitance same as in previous case. The dielectric constant of the slab is

(Given $ε_{0} = 8.834 \times 10^{- 12}$ F/m) [2024]

1
0.66
1.33
1.50

1

2

3

4

(4)

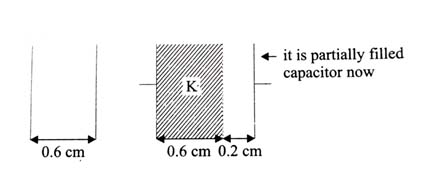
Parallel plate capacitance, $C = \frac{ϵ_{o} A}{d_{1}}$

For partially filled capacity, $C = \frac{ϵ_{o} A}{(d_{2} - t + \frac{t}{k})}$

For case (i) $C_{1} = \frac{ϵ_{o} A}{0.6}$ , For case (ii) $C_{2} = \frac{ϵ_{o} A}{0.8 - 0.6 + \frac{0.6}{K}}$

Now $C_{1} = C_{2}, \frac{ϵ_{o} A}{0.6} = \frac{ϵ_{o} A}{0.2 + \frac{0.6}{K}}$ ,

$0.6 = 0.2 + \frac{0.6}{K} \Rightarrow K = \frac{3}{2}$

Q 4 :

Consider a parallel plate capacitor of area A (of each plate) and separation $' d'$ between the plates. If E is the electric field and $ε_{0}$ is the permittivity of free space between the plates, then potential energy stored in the capacitor is [2025]

$\frac{1}{2} ε_{0} E^{2} A d$
$\frac{3}{4} ε_{0} E^{2} A d$
$\frac{1}{4} ε_{0} E^{2} A d$
$ε_{0} E^{2} A d$

1

2

3

4

(1)

We know energy density

$ρ_{av} = \frac{1}{2} ε_{0} E^{2}$

So potential energy $= ρ_{av} \times$ Volume

$\Rightarrow P . E . = \frac{1}{2} ε_{0} E^{2} A d$

Q 5 :

A parallel plate capacitor was made with two rectangular plates, each with a length of $l$ = 3 cm and breath of b = 1 cm. The distance between the plates is 3 $μ$ m. Out of the following, which are the ways to increase the capacitance by a factor of 10?

A. $l$ = 30 cm, b = 1 cm, d = 1 $μ$ m

B. $l$ = 3 cm, b = 1 cm, d = 30 $μ$ m

C. $l$ = 6 cm, b = 5 cm, d = 3 $μ$ m

D. $l$ = 1 cm, b = 1 cm, d = 10 $μ$ m

E. $l$ = 5 cm, b = 2 cm, d = 1 $μ$ m

Choose the correct answer from the options given below: [2025]

C and E only
B and D only
A only
C only

1

2

3

4

(1)

We know $C = \frac{A ε_{0}}{d} = \frac{b l ε_{0}}{d}$

So, to increase the capacitance by factor $10 (\frac{A}{d})$ has to increase by factor 10.

For option (A) $C' = 30 C$

For option (B) $C' = \frac{C}{10}$

For option (C) $C' = 10 C$

For option (D) $C' = \frac{C}{10}$

For option (E) $C' = 10 C$

Q 6 :

A parallel plate capacitor of capacitance 1 $μ$ F is charged to a potential difference of 20 V. The distance between plates is 1 $μ$ F. The energy density between plates of capacitor is : [2025]

$1.8 \times 10^{3} J / m^{3}$
$2 \times 10^{4} J / m^{3}$
$2 \times 10^{2} J / m^{3}$
$1.8 \times 10^{5} J / m^{3}$

1

2

3

4

(1)

Energy density $u = \frac{1}{2} ε_{0} E^{2} = \frac{1}{2} ε_{0} {(\frac{V}{d})}^{2}$

$\Rightarrow u = \frac{1}{2} (8.85 \times 10^{- 12}) {(\frac{20}{10^{- 6}})}^{2} J / m^{3} = 1.8 \times 10^{3} J / m^{3}$

Q 7 :

A parallel-plate capacitor of capacitance 40 $μ$ F is connected to a 100 V power supply. Now the intermediate space between the plates is filled with a dielectric material of dielectric constant K = 2. Due to the introduction of dielectric material, the extra charge and the change in the electrostatic energy in the capacitor, respectively, are [2025]

2 mC and 0.2 J
8 mC and 2.0 J
4 mC and 0.2 J
2 mC and 0.4 J

1

2

3

4

(3)

$C = 40 μ F, C' = k C = 80 μ F$

V = 100 V

Q = CV = 4 mC

$Q' = C' V = 8 mC$

$∴$ Extra charge, $△ Q$ = 4 mC

$U_{1} = \frac{1}{2} {CV}^{2}, U_{2} = \frac{1}{2} C' V^{2}$

$△ U = \frac{1}{2} (C' - C) V^{2} = \frac{1}{2} \times 40 \times 10^{4} \times 10^{- 6} = 0.2 J$

Q 8 :

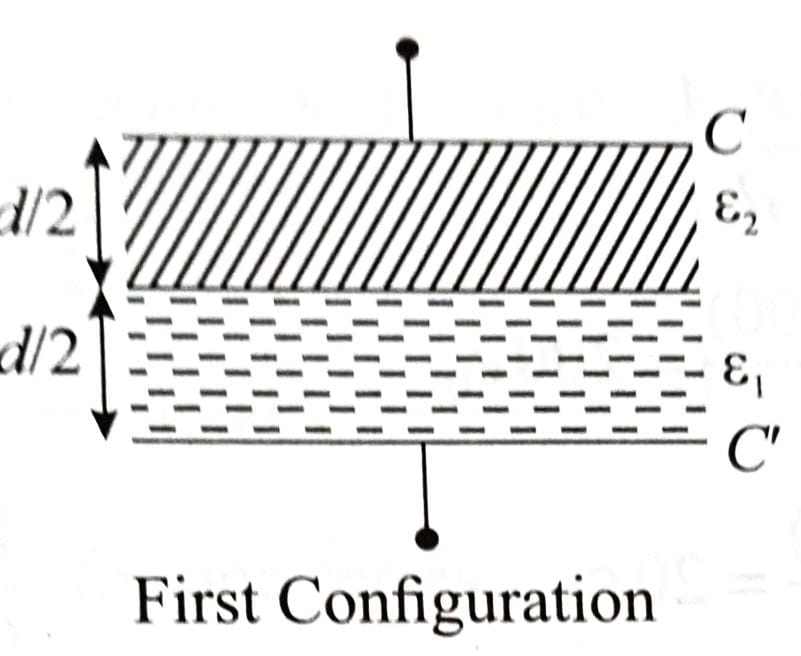
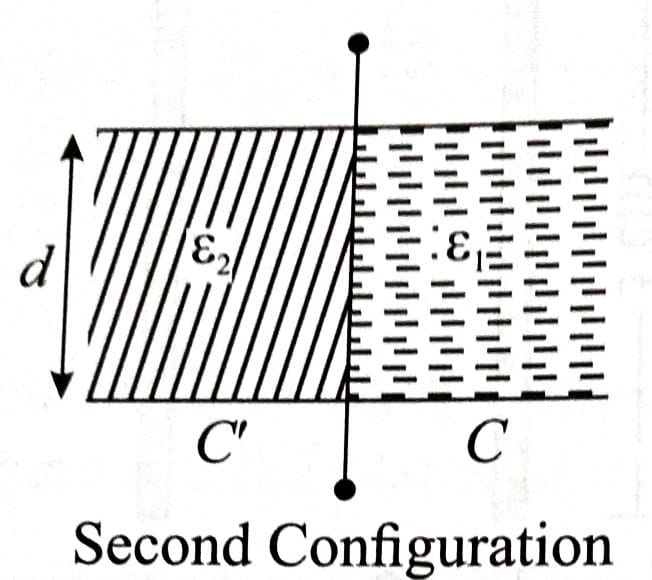
A parallel plate capacitor is filled equally (half) with two dielectrics of dielectric constant $ε_{1}$ and $ε_{2}$ , as shown in figures. The distance between the plates is d and area of each plate is A. If capacitance in first configuration and second configuration are $C_{1}$ and $C_{2}$ respectively, then $\frac{C_{1}}{C_{2}}$ is [2025]

$\frac{ε_{1} ε_{2}^{2}}{{(ε_{1} + ε_{2})}^{2}}$
$\frac{4 ε_{1} ε_{2}}{{(ε_{1} + ε_{2})}^{2}}$
$\frac{ε_{1} ε_{2}}{ε_{1} + ε_{2}}$
$\frac{ε_{0} (ε_{1} + ε_{2})}{2}$

1

2

3

4

(2)

$C_{1} = \frac{ε_{0} A}{\frac{d}{2 ε_{1}} + \frac{d}{2 ε_{2}}} = \frac{ε_{0} A}{d} {\frac{1}{\frac{ε_{2} + ε_{1}}{2 ε_{1} ε_{2}}}}$

$C_{2} = \frac{ε_{0}}{d} (ε_{1} \frac{A}{2} + ε_{2} \frac{A}{2}) = \frac{ε_{0} A}{d} {\frac{ε_{1}}{2} + \frac{ε_{2}}{2}}$

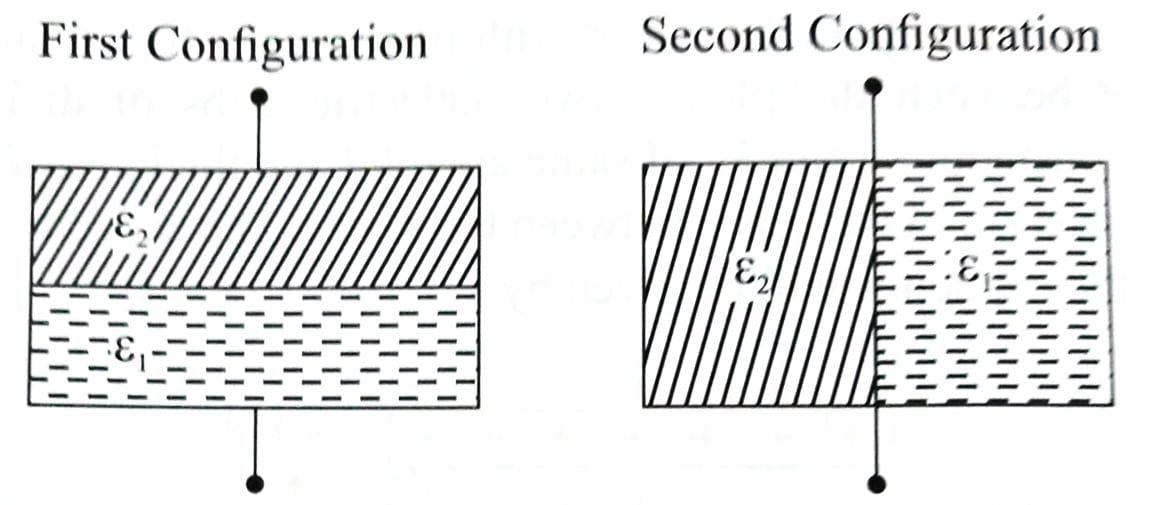
$\frac{C_{1}}{C_{2}} = \frac{2 ε_{1} ε_{2}}{(ε_{2} + ε_{1}) \frac{(ε_{1} + ε_{2})}{2}} = \frac{4 ε_{1} ε_{2}}{{(ε_{1} + ε_{2})}^{2}}$

Q 9 :

Three parallel plate capacitors $C_{1}, C_{2}$ and $C_{3}$ each of capacitance 5 $μ$ F are connected as shown in figure. The effective capacitance between points A and B, when the space between the parallel plates of $C_{1}$ capacitor is filled with a dielectric medium having dielectric constant of 4, is [2025]

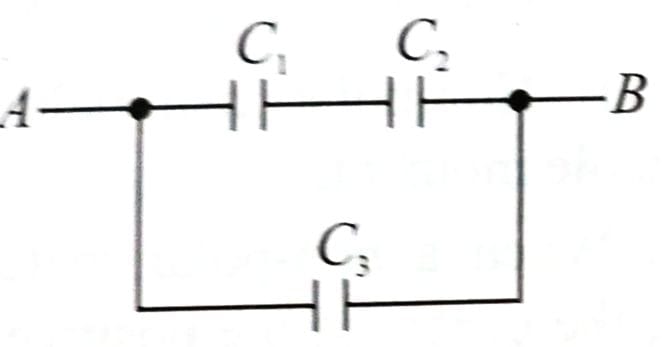
22.5 $μ F$
7.5 $μ F$
9 $μ F$
30 $μ F$

1

2

3

4

(3)

$C_{eq} = (\frac{K C \cdot C}{K C + C}) + C = (\frac{K}{K + 1}) C + C$

$\Rightarrow C_{eq} = \frac{4}{5} \times 5 + 5 = 9 μ F$

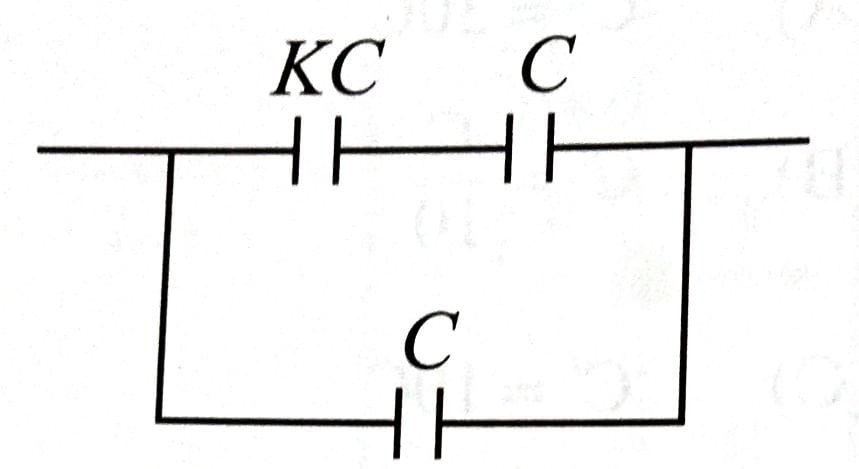
Q 10 :

A parallel plate capacitor has charge $5 \times 10^{- 6} C$ . A dielectric slab is inserted between the plates and almost fills the space between the plates. If the induced charge on one face of the slab is $4 \times 10^{- 6} C$ then the dielectric constant of the slab is __________ [2025]

(5)

$E_{net} = E_{0} - E_{i n}$

$E_{in} = E_{0} (1 - \frac{1}{k})$

$Q_{in} = Q_{0} (1 - \frac{1}{k})$

$4 \times 10^{- 6} = 5 \times 10^{- 6} (1 - \frac{1}{k}) \Rightarrow k = 5$

Q 11 :

A parallel plate capacitor has plate area $40 {cm}^{2}$ and plate separation 2 mm. The space between the plates is filled with a dielectric medium of thickness 1 mm and dielectric constant 5. The capacitance of the system is [2023]

$\frac{10}{3} ε_{0} F$
$\frac{3}{10} ε_{0} F$
$24 ε_{0} F$
$10 ε_{0} F$

1

2

3

4

(1)

$A = 40 \times 10^{- 4} m^{2} = 4 \times 10^{- 3} m^{2}$

$d = 2 \times 10^{- 3} m, k = 5, t = 10^{- 3} m, C = ?$

$C = \frac{ε_{0} A}{d - t + \frac{t}{k}}$ $= \frac{ε_{0} \times 4 \times 10^{- 3}}{(2 - 1) \times 10^{- 3} + \frac{10^{- 3}}{5}}$ $= \frac{4 ε_{0} \times 5}{6} = \frac{10 ε_{0}}{3} F$

Q 12 :

The distance between two plates of a capacitor is $d$ and its capacitance is $C_{1}$ , when air is the medium between the plates. If a metal sheet of thickness $\frac{2 d}{3}$ and of the same area as the plates is introduced between the plates, the capacitance of the capacitor becomes $C_{2}$ . The ratio $\frac{C_{2}}{C_{1}}$ is [2023]

2 : 1
4 : 1
3 : 1
1 : 1

1

2

3

4

(3)

$K_{metal sheet} = \infty, t = \frac{2 d}{3}$

$C_{1} = \frac{ε_{0} A}{d}$

$C_{2} = \frac{ε_{0} A}{d - t + \frac{t}{k}} = \frac{ε_{0} A}{d - \frac{2 d}{3} + 0} = 3 C_{1}$

$\frac{C_{2}}{C_{1}} = 3$

Q 13 :

A parallel plate capacitor with air between the plates has a capacitance of 15 pF. The separation between the plates becomes twice and the space between them is filled with a medium of dielectric constant 3.5. Then the capacitance becomes $\frac{x}{4} pF$ . The value of $x$ is _________. [2023]

(105)

$C_{0} = \frac{ε_{0} A}{d} = 15 pF$

$C = \frac{K ε_{0} A}{2 d} = \frac{3.5}{2} \times 15 pF = \frac{105}{4} pF$

Q 14 :

A capacitor has capacitance $5 μ F$ when its parallel plates are separated by air medium of thickness $d$ . A slab of material of dielectric constant 1.5 having area equal to that of the plates but thickness $\frac{d}{2}$ is inserted between the plates. The capacitance of the capacitor in the presence of the slab will be _________ $μ F$ . [2023]

(6)

$\frac{ε_{0} A}{d} = 5 μ F$

$C_{new} = \frac{ε_{0} A}{\frac{(\frac{d}{2})}{1.5} + \frac{(\frac{d}{2})}{1}}$

$= \frac{ε_{0} A}{(\frac{d}{3} + \frac{d}{2})} = \frac{6 ε_{0} A}{5 d} = \frac{6}{5} \times 5 μ F = 6 μ F$

Q 15 :

Two parallel plate capacitors $C_{1}$ and $C_{2}$ , each having capacitance of $10 μ F$ , are individually charged by a 100 V D.C. source. Capacitor $C_{1}$ is kept connected to the source and a dielectric slab is inserted between its plates. Capacitor $C_{2}$ is disconnected from the source and then a dielectric slab is inserted in it. Afterwards, the capacitor $C_{1}$ is also disconnected from the source and the two capacitors are finally connected in parallel combination. The common potential of the combination will be ____ V. (Assuming dielectric constant =10) [2023]

(55)

$Charge on C_{1} = K C E$

$and charge on C_{2} = C E$

When they are connected in parallel, charge will be equally divided, so charge on one capacitor is

$q = \frac{K + 1}{2} C V$

$So$ $V = \frac{q}{K C} = \frac{K + 1}{2 K} = 55 V$

Q 16 :

A parallel plate capacitor with plate area $A$ and plate separation $d$ is filled with a dielectric material of dielectric constant K = 4. The thickness of the dielectric material is $x$ . where $x < d$ .

Let $C_{1}$ and $C_{2}$ be the capacitance of the system for $x = \frac{1}{3} d$ and $x = \frac{2 d}{3}$ respectively. If $C_{1} = 2 μ F$ , the value of $C_{2}$ is ________ $μ F$ . [2023]

(3)

$For x = \frac{d}{3}$

$C_{1} = \frac{ε_{0} A}{(\frac{d / 3}{k} + \frac{2 d}{3})} = \frac{ε_{0} A}{\frac{d}{12} + \frac{2 d}{3}} = \frac{ε_{0} A}{d} \times \frac{12}{9}$

$C_{1} = \frac{4}{3} \frac{ε_{0} A}{d} = 2 μ F$

$For x = \frac{2 d}{3}$

$C_{2} = \frac{ε_{0} A}{(\frac{2 d / 3}{k} + \frac{d}{3})} = \frac{ε_{0} A}{d} \times 2 = \frac{6}{4} \times 2 = 3 μ F$

Q 17 :

A parallel plate capacitor has capacitance C, when there is vacuum within the parallel plates. A sheet having thickness ${(1 / 3)}^{rd}$ of the separation between the plates and relative permittivity K is introduced between the plates. The new capacitance of the system is : [2026]

$\frac{C K}{2 + K}$
$\frac{3 C K^{2}}{{(2 K + 1)}^{2}}$
$\frac{3 K C}{2 K + 1}$
$\frac{4 K C}{3 K - 1}$

1

2

3

4

(3)

$C_{1} = \frac{3 A ε_{0}}{2 d}$ $C_{2} = \frac{3 A ε_{0} \times K}{d}$

$C_{1} = \frac{3}{2} C$ $C_{2} = 3 K C$

$C_{eq} = \frac{C_{1} C_{2}}{C_{1} + C_{2}} = \frac{\frac{3}{2} C \times 3 K C}{\frac{3}{2} C + 3 K C}$

$C_{eq} = \frac{\frac{9}{2} K C^{2}}{\frac{3}{2} C (2 K + 1)} = \frac{3 K C}{2 K + 1}$

Q 18 :

Identify the correct statements:

A. Effective capacitance of a series combination of capacitors is always smaller than the smallest capacitance of the capacitor in the combination.

B. When a dielectric medium is placed between the charged plates of a capacitor, displacement of charges cannot occur due to insulation property of dielectric.

C. Increasing of area of capacitor plate or decreasing of thickness of dielectric is an alternate method to increase the capacitance.

D. For a point charge, concentric spherical shells centered at the location of the charge are equipotential surfaces.

Choose the correct answer from the options given below: [2026]

A, C and D Only
B and D Only
C and D Only
A, B and C Only

1

2

3

4

(1)

For series combination

$\frac{1}{C_{eq}} = \frac{1}{C_{1}} + \frac{1}{C_{2}}$

$∴$ $C_{eq} is less than C_{1} & C_{2} .$

Note: In statement C, capacitor is assumed to be completely filled with dielectric then on decreasing thickness of dielectric capacitance will increase.

Q 19 :

A parallel plate capacitor with plate separation 5 mm is charged by a battery. On introducing a mica sheet of 2 mm and maintaining the connections of the plates with the terminals of the battery, it is found that it draws 25% more charge from the battery. The dielectric constant of mica is __________. [2026]

2.0
1.5
1.0
2.5

1

2

3

4

(1)

$c_{eq} = \frac{C_{1} C_{2}}{C_{1} + C_{2}} = \frac{\frac{ε_{0} A}{3} \times \frac{K ε_{0} A}{2}}{\frac{ε_{0} A}{3} + \frac{K ε_{0} A}{2}}$

$c_{eq} = \frac{{(ε_{0} A)}^{2} (\frac{K}{6})}{ε_{0} A (\frac{2 + 3 K}{6})} \Rightarrow C_{eq} = \frac{K ε_{0} A}{2 + 3 K}$

$1.25 \times \frac{ε_{0} A}{5} = \frac{K ε_{0} A}{2 + 3 K} \Rightarrow 0.25 (2 + 3 K) = K$

$2 + 3 K = 4 K \Rightarrow K = 2$

Topic Question Set

Q 4 :

Consider a parallel plate capacitor of area A (of each plate) and separation $' d'$ between the plates. If E is the electric field and $ε_{0}$ is the permittivity of free space between the plates, then potential energy stored in the capacitor is [2025]

Q 6 :

A parallel plate capacitor of capacitance 1 $μ$ F is charged to a potential difference of 20 V. The distance between plates is 1 $μ$ F. The energy density between plates of capacitor is : [2025]

Q 10 :

A parallel plate capacitor has charge $5 \times 10^{- 6} C$ . A dielectric slab is inserted between the plates and almost fills the space between the plates. If the induced charge on one face of the slab is $4 \times 10^{- 6} C$ then the dielectric constant of the slab is __________ [2025]

Q 11 :

A parallel plate capacitor has plate area $40 {cm}^{2}$ and plate separation 2 mm. The space between the plates is filled with a dielectric medium of thickness 1 mm and dielectric constant 5. The capacitance of the system is [2023]

Q 17 :

A parallel plate capacitor has capacitance C, when there is vacuum within the parallel plates. A sheet having thickness ${(1 / 3)}^{rd}$ of the separation between the plates and relative permittivity K is introduced between the plates. The new capacitance of the system is : [2026]

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 4 : Consider a parallel plate capacitor of area A (of each plate) and separation 'd' between the plates. If E is the electric field and ε0 is the permittivity of free space between the plates, then potential energy stored in the capacitor is [2025]

Q 6 : A parallel plate capacitor of capacitance 1 μF is charged to a potential difference of 20 V. The distance between plates is 1 μF. The energy density between plates of capacitor is : [2025]

Q 9 : Three parallel plate capacitors C1,C2 and C3 each of capacitance 5 μF are connected as shown in figure. The effective capacitance between points A and B, when the space between the parallel plates of C1 capacitor is filled with a dielectric medium having dielectric constant of 4, is [2025]

Q 10 : A parallel plate capacitor has charge 5×10–6C. A dielectric slab is inserted between the plates and almost fills the space between the plates. If the induced charge on one face of the slab is 4×10–6C then the dielectric constant of the slab is __________ [2025]

Q 11 : A parallel plate capacitor has plate area 40 cm2 and plate separation 2 mm. The space between the plates is filled with a dielectric medium of thickness 1 mm and dielectric constant 5. The capacitance of the system is [2023]

Q 13 : A parallel plate capacitor with air between the plates has a capacitance of 15 pF. The separation between the plates becomes twice and the space between them is filled with a medium of dielectric constant 3.5. Then the capacitance becomes x4 pF. The value of x is _________. [2023]

Q 17 : A parallel plate capacitor has capacitance C, when there is vacuum within the parallel plates. A sheet having thickness (1/3)rd of the separation between the plates and relative permittivity K is introduced between the plates. The new capacitance of the system is : [2026]

Want Us to Email you About Special Offers & Updates?

Q 4 :

Consider a parallel plate capacitor of area A (of each plate) and separation $' d'$ between the plates. If E is the electric field and $ε_{0}$ is the permittivity of free space between the plates, then potential energy stored in the capacitor is [2025]

Q 6 :

A parallel plate capacitor of capacitance 1 $μ$ F is charged to a potential difference of 20 V. The distance between plates is 1 $μ$ F. The energy density between plates of capacitor is : [2025]

Q 10 :

A parallel plate capacitor has charge $5 \times 10^{- 6} C$ . A dielectric slab is inserted between the plates and almost fills the space between the plates. If the induced charge on one face of the slab is $4 \times 10^{- 6} C$ then the dielectric constant of the slab is __________ [2025]

Q 11 :

A parallel plate capacitor has plate area $40 {cm}^{2}$ and plate separation 2 mm. The space between the plates is filled with a dielectric medium of thickness 1 mm and dielectric constant 5. The capacitance of the system is [2023]

Q 17 :

A parallel plate capacitor has capacitance C, when there is vacuum within the parallel plates. A sheet having thickness ${(1 / 3)}^{rd}$ of the separation between the plates and relative permittivity K is introduced between the plates. The new capacitance of the system is : [2026]