In an ac circuit, the instantaneous current is zero, when the instantaneous voltage is maximum. In this case, the source may be connected to
a) pure inductor
b) pure capacitor
c) pure resistor
d) combination of an inductor and capacitor
Choose the correct answer from the options given below [2024]
b, c and d only
a, b and d only
a and b only
a, b and c only
(2)
This is possible when phase difference is between current and voltage.
A series LCR circuit is subjected to an ac signal of 200 V, 50 Hz. If the voltage across the inductor (L = 10 mH) is 31.4 V, then the current in this circuit is [2024]
68 A
63 A
10 A
10 mA
(3)
Voltage across inductor
Given below are two statements:
Statement I: In an LCR series circuit, current is maximum at resonance.
Statement II: Current in a purely resistive circuit can never be less than that in a series LCR circuit when connected to same voltage source.
In the light of the above statements, choose the correct from the options given below: [2024]
Statement I is true but Statement II is false
Statement I is false but Statement II is true
Both Statement I and Statement II are false
Both Statement I and Statement II are true
(4)
Statement-I
Impendence is minimum therefore is maximum at resonance.
Statement-II
A LCR circuit is at resonance for a capacitor C, inductance L and resistance R. Now the value of resistance is halved keeping all other parameters the same. The current amplitude at resonance will be now [2024]
halved
Zero
same
double
(4)
At resonance: Z = R
So
In series LCR circuit, the capacitance is changed from C to 4C. To keep the resonance frequency unchanged, the new inductance should be [2024]
reduced to L/4
increased by 2L
reduced by L/3
increased to 4L
(1)
For a given series LCR circuit it is found that maximum current is drawn when value of variable capacitance is 2.5 nF. If resistance of 200 and 100 mH inductor are being used in the given circuit.
The frequency of ac source is _________ Hz. (given ) [2024]
(10) For maximum current, circuit must be in resonance.
At resonance,
A series LCR circuit with , and , is connected across an ac source of 220V, 50 Hz supply. The power factor of the circuit would be _________ . [2024]
(1)
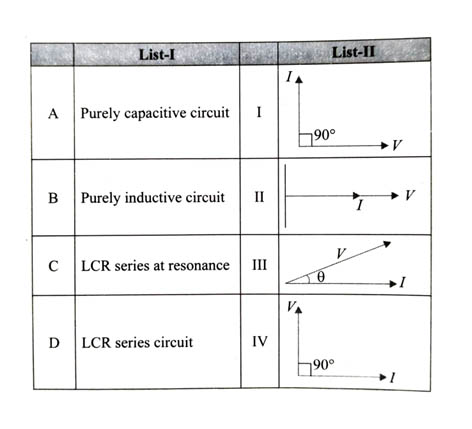
Match List-I with List-II [2024]
Choose the correct answer from the options given below
A-IV, B-I, C-II, D-III
A-I, B-IV, C-III, D-II
A-I, B-IV, C-II, D-III
A-IV, B-I, C-III, D-II
(3)
A - V lags by 90° from I, hence option (I) is correct.
B - V lead by 90° from I, hence option (IV) is correct.
C - In LCR resonance . Hence circuit is purely resistive, so option (II) is correct.
D - In LCR series V is at some angle from I hence (III) is correct.
An AC source is connected in a given series LCR circuit. The RMS potential difference across the capacitor of 20 is ________ V. [2024]
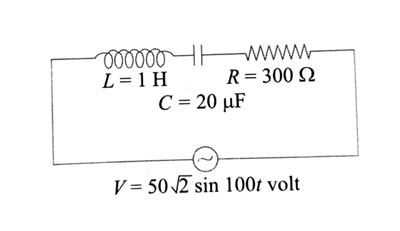
(50)
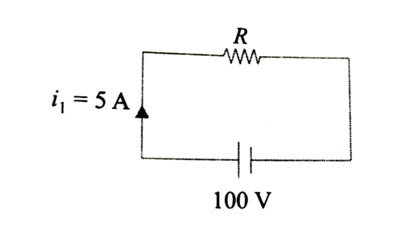
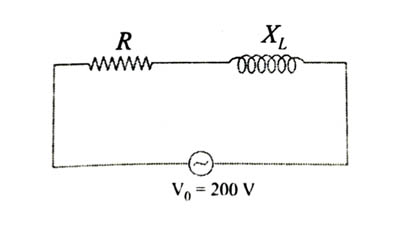
When a DC voltage of 100 V is applied to an inductor, a DC current of 5 A flows through it. When an AC voltage of 200 V peak value is connected to the inductor, its inductive reactance is found to be . The power dissipated in the circuit is ______ W. [2024]
(250)