A force displaces a body from to Work done by this force is __________ J. [2024]
(58)
A body of mass 50 kg is lifted to a height of 20 m from the ground in the two different ways as shown in the figures. The ratio of work done against the gravity in both the respective cases, will be [2024]

2 : 1
1 : 2
3 : 2
1 : 1
(4)
Work done by gravity is path independent, it depends only on vertical displacement, so same work is done in both paths.
A block is simply released from the top of an inclined plane as shown in the figure above. The maximum compression in the spring when the block hits the spring is [2024]
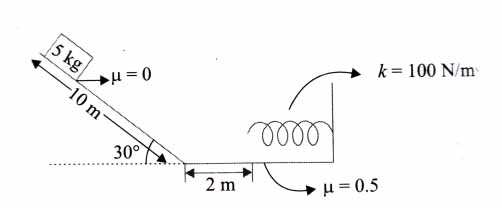
1 m
m
m
2 m
(4)
At maximum compression
using Work-Energy Theorem,
Nearest answer is 2m.
A block of mass 100 kg slides over a distance of 10 m on a horizontal surface. If the coefficient of friction between the surfaces is 0.4, then the work done against friction (in J) is [2024]
4200
3900
4000
4500
(3)
Given
Friction force,
Now
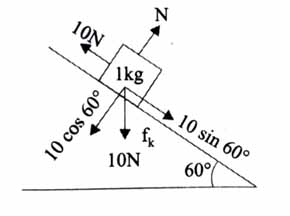
A block of mass 1 kg is pushed up a surface inclined to horizontal at an angle of 60 by a force of 10 N parallel to the inclined surface as shown in the figure. When the block is pushed up by 10 m along the inclined surface, the work done against frictional force is [2024]
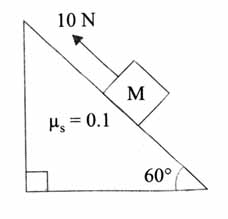
J
5 J
J
10 J
(2)
A force is applied on a particle and it undergoes a displacement . What will be the value of b, if work done on the particle is zero. [2025]
0
2
(2)
Given, W = 0
A force acts on an object in the x-direction. The work done by the force is 5 J when the object is displaced by 1 m. If the constant = 1 N then will be [2025]
(3)
Work done
Given = 1
So,
A block of mass 25 kg is pulled along a horizontal surface by a force at an angle 45° with the horizontal. The friction coefficient between the block and the surface is 0.25. The block travels at a uniform velocity. The work done by the applied force during a displacement of 5 m of the block is: [2025]
970 J
735 J
245 J
490 J
(3)
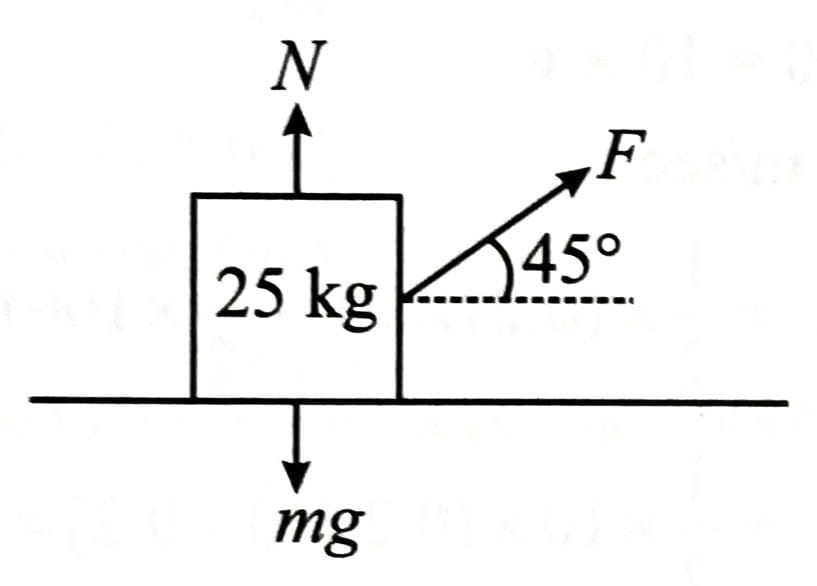
Block travels with uniform velocity
.
A force acts on a particle in a plane x + y = 10. The work done by this force during a displacement from (0, 0) to (4 m , 2 m) is _____ Joule (round off to the nearest integer) [2025]
(152)
y = 10 – x
.