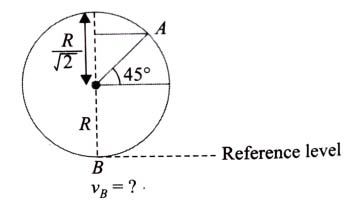
A body of m kg slides from rest along the curve of a vertical circle from point A to B in a frictionless path. The velocity of the body at B is ______.
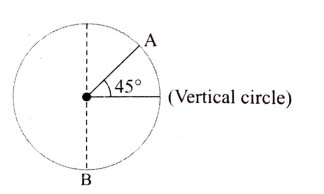
[2024]
21.9 m/s
10.6 m/s
19.8 m/s
16.7 m/s
(1)
By conservation of mechanical energy, decrease in P.E. = increase in K.E.
A ball suspended by a thread swings in a vertical plane so that its magnitude of acceleration in the extreme position and lowest position are equal. The angle (θ) of thread deflection in the extreme position will be ______. [2024]
(2)
Loss in kinetic energy = Gain in potential energy
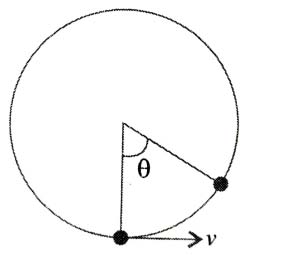
Acceleration at lowest point
Acceleration at extreme point
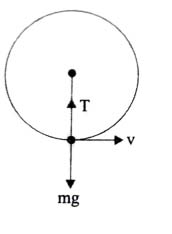
A stone of mass 900 g is tied to a string and moved in a vertical circle of radius 1 m, making 10 rpm. The tension in the string when the stone is at the lowest point is (if = 9.8 and g = 9.8 ) [2024]
17.8 N
8.82 N
97 N
9.8 N
(4)
Given that,
A bob of mass 'm' is suspended by a light string of length 'L'. It is imparted a minimum horizontal velocity at the lowest point A such that it just completes a half-circle, reaching the topmost position B. The ratio of kinetic energies is ______. [2024]
3 : 2
5 : 1
2 : 5
1 : 5
(2)
Apply energy conservation
So,
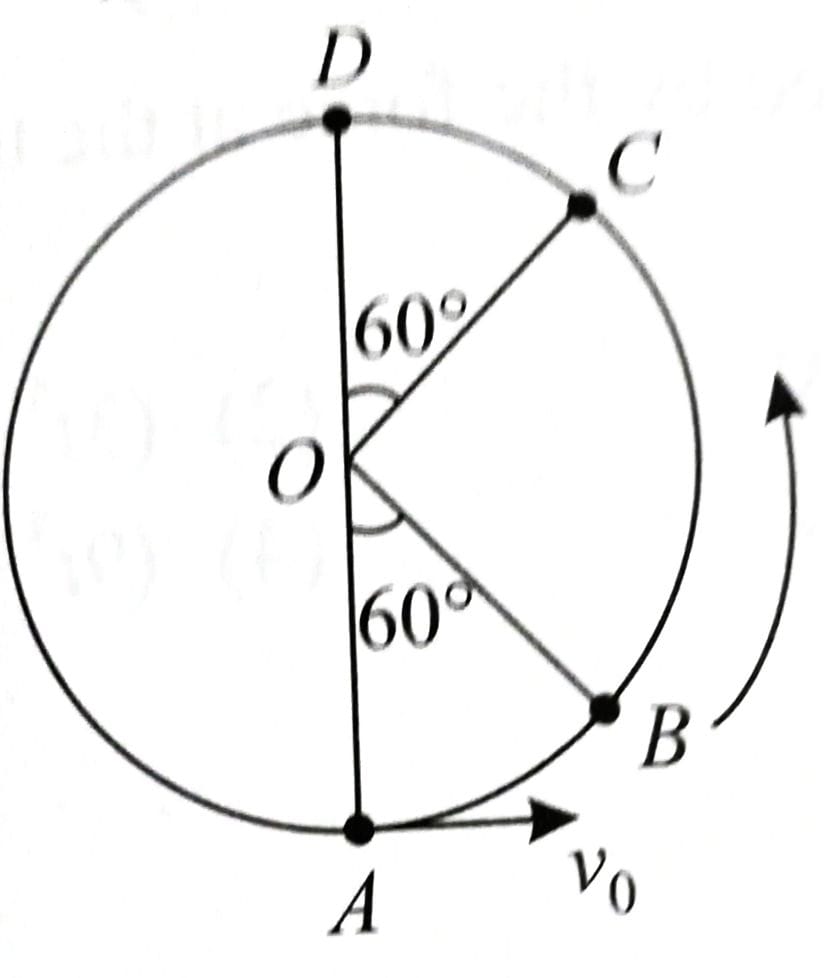
A bob of mass m is suspended at a point O by a light string of length and left to perform vertical motion (circular) as shown in figure. Initially, by applying horizontal velocity at the point 'A'. The string becomes slack when, the bob reaches at the point 'D'. The ratio of the kinetic energy of the bob at the points B and C is [2025]
2
1
4
3
(1)
Applying conservation of mechanical energy,
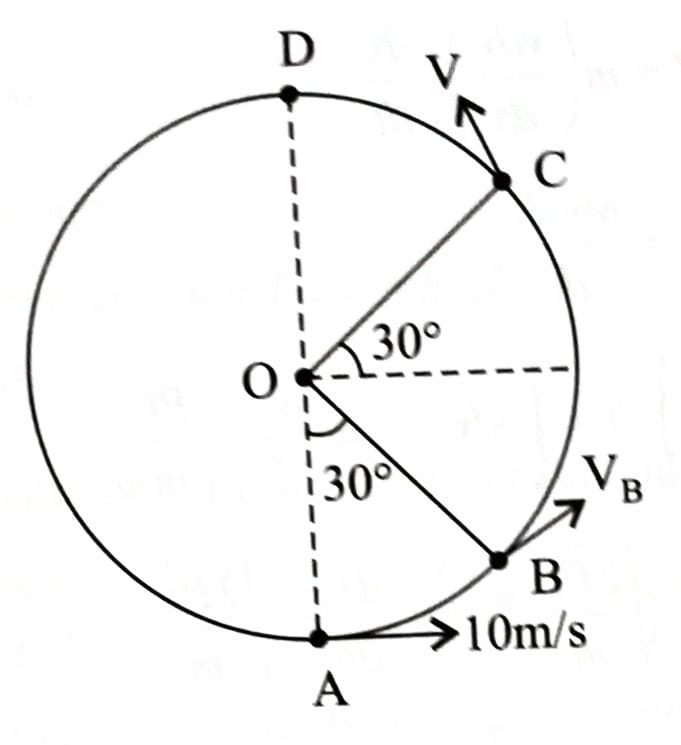
A body of mass 100 g is moving in circular path of radius 2 m on vertical plane as shown in figure. The velocity of the body at point A is 10 m/s. The ratio of its kinetic energies at point B and C is: [2025]

(Take acceleration due to gravity as 10 )
(3)
...(i)
A body of mass 'm' connected to a massless and unstretchable string goes in verticle circle of radius 'R' under gravity g. The other end of the string is fixed at the center of circle. If velocity at top of circular path is , where , then ratio of kinetic energy of the body at bottom to that at top of the circle is [2025]
(4)
