Topic Question Set
Q 1
:
Let be a real valued function. If and are respectively the minimum and the maximum values of then is equal to [2024]
42
44
24
38
(1)
Now,
Q 2
:
If the function attains the maximum value at then [2024]
(3)
Q 3
:
If the function has a local maximum at and a local minimum at then and are the roots of the equation : [2024]
(4)
Q 4
:
A variable line passes through the point (3, 5) and intersects the positive coordinate axes at the points and . The minimum area of the triangle where is the origin, is: [2024]
35
40
25
30
(4)
Equation of line
Since, it passes through (3, 5)
Area of triangle
So for minimum area,
Q 5
:
The maximum area of a triangle whose one vertex is at (0, 0) and the other two vertices lie on the curve at points and where is [2024]
108
122
88
92
(1)
Let ABC be the required triangle whose area is A.
For critical point,
Now,
Maximum area,
Q 6
:
[2024]
600
392
108
608
(4)
Given, ...(i)
Differentiate (i), w.r.t. we get
For maxima / minima,
Now find the value of (i) at
Maximum value,
Minimum value,
Hence,
Q 7
:
Let a rectangle ABCD of sides 2 and 4 be inscribed in another rectangle PQRS such that the vertices of the rectangle ABCD lie on the sides of the rectangle PQRS. Let and be the sides of the rectangle PQRS when its area is maximum. Then is equal to: [2024]
80
60
64
72
(4)
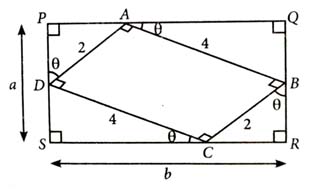
In , we have
Similarly, in we have
Similarly,
Area of
Area is maximum, when
and
Now,
Q 8
:
Let . The number of points of local maxima of in the interval is: [2024]
2
3
4
1
(1)
Critical points are
Sign of
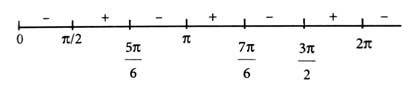
Points of local maxima
Q 9
:
The function has [2024]
exactly one point of local minima and no point of local maxima
exactly two points of local maxima and exactly one point of local minima
exactly one point of local maxima and no point of local minima
exactly one point of local maxima and exactly one point of local minima
(4)
We have,
For critical points,
The sign scheme of is

Q 10
:
Let A be the region enclosed by the parabola and the line . Then the maximum area of the rectangle inscribed in the region A is _____. [2024]
(128)

Area of the rectangle,
Now,
Hence, for maximum area,
Hence, maximum area

