Heating Effect of Current and Electrical Measuring Instruments | Physics JEE previous year questions with Solutions

Q 1 :
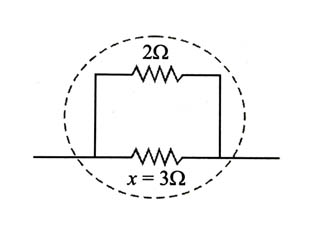
The value of unknown resistance (x) for which the potential between B and D will be zero in the arrangement shown, is [2024]

$6 Ω$
$9 Ω$
$42 Ω$
$3 Ω$

1

2

3

4

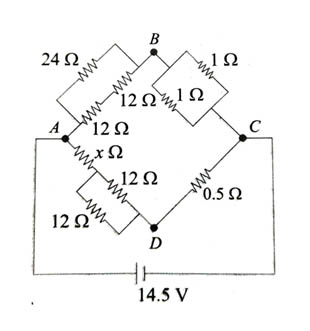
(1)

Potential difference between B and D will be zero in a balanced

Wheatstone Bridge

In case of balanced Wheatstone Bridge,

$\frac{V_{A B}}{V_{A D}} = \frac{V_{B C}}{V_{C D}} \Rightarrow \frac{12}{6 + x} = \frac{0.5}{0.5} \Rightarrow x = 6 Ω$

Q 2 :
The resistance per centimeter of a meter bridge wire is r, with $X Ω$ resistance in left gap. Balancing length from left end is at 40 cm with $25 Ω$ resistance in right gap. Now the wire is replaced by another wire of $2 r$ resistance per centimeter. The new balancing length for same settings will be at [2024]

20 cm
10 cm
80 cm
40 cm

1

2

3

4

(4)

$\frac{25}{r ℓ_{2}} = \frac{X}{r ℓ_{1}}$ ...(i)

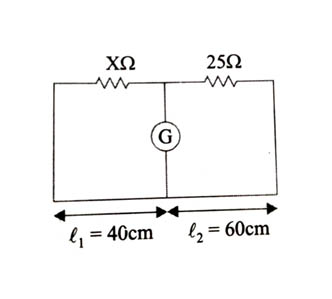
$\frac{25}{2 r ℓ'_{2}} = \frac{X}{2 r ℓ'_{1}}$ ...(ii)

From (i) and (ii) $ℓ'_{2} = ℓ_{2} = 40 c m$

Q 3 :
To measure the temperature coefficient of resistivity $α$ of a semiconductor, an electrical arrangement shown in the figure is prepared. The arm BC is made up of the semiconductor. The experiment is being conducted at 25 °C and resistance of the semiconductor arm is 3 $m Ω$ . Arm BC is cooled at a constant rate of 2° C/s. If the galvanometer G shows no deflection after 10 s, then $α$ is [2024]

$- 1 \times 10^{- 2}$ ${}^{o}{C^{- 1}}$
$- 2 \times 10^{- 2}$ ${}^{o}{C^{- 1}}$
$- 2.5 \times 10^{- 2}$ ${}^{o}{C^{- 1}}$
$- 1.5 \times 10^{- 2}$ ${}^{o}{C^{- 1}}$

1

2

3

4

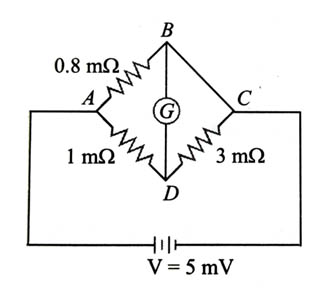
(1)

For no. deflection $= \frac{0.8}{1} = \frac{R}{3}$

$R = 2.4 m Ω$

$Δ R = R α Δ T$

$\Rightarrow α = \frac{Δ R}{R Δ T} = \frac{- 0.6}{3 \times 20} = - 10^{- 2}$ ${}^{o}{C^{- 1}}$

Q 4 :
In a metre-bridge when a resistance in the left gap is $2 Ω$ and unknown resistance in the right gap, the balance length is found to be 40 cm. On shunting the unknown resistance with $2 Ω$ , the balance length changes by [2024]

22.5 cm
20 cm
62.5 cm
65 cm

1

2

3

4

(1)

Given for $l_{1} = 40$ cm
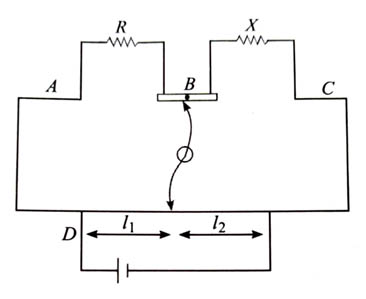
$\frac{R}{l_{1}} = \frac{X}{l_{2}} \Rightarrow \frac{2}{40} = \frac{X}{60}$

$x = 3 Ω$

After shunting

$R_{eq} = \frac{2 \times 3}{3 + 2} = \frac{6}{5} Ω$

$\frac{R}{l} = \frac{R_{eq}}{100 - l} \Rightarrow \frac{2}{l} = \frac{6}{5 (100 - l)}$

$\Rightarrow 10 (100 - l) = 6 l$

$500 - 5 l = 3 l$

$500 = 8 l$

$l = \frac{500}{8} = \frac{125}{2} = 62.5 cm$

Balance length change

by $= 62.5 - 40 = 22.5 cm$

Topic Question Set

Q 1 :
The value of unknown resistance (x) for which the potential between B and D will be zero in the arrangement shown, is [2024]

Q 4 :
In a metre-bridge when a resistance in the left gap is $2 Ω$ and unknown resistance in the right gap, the balance length is found to be 40 cm. On shunting the unknown resistance with $2 Ω$ , the balance length changes by [2024]

Want Us to Email you About Special Offers & Updates?

Topic Question Set

Q 1 : The value of unknown resistance (x) for which the potential between B and D will be zero in the arrangement shown, is [2024]

Q 4 : In a metre-bridge when a resistance in the left gap is 2Ω and unknown resistance in the right gap, the balance length is found to be 40 cm. On shunting the unknown resistance with 2Ω, the balance length changes by [2024]

Want Us to Email you About Special Offers & Updates?

Q 1 :
The value of unknown resistance (x) for which the potential between B and D will be zero in the arrangement shown, is [2024]

Q 4 :
In a metre-bridge when a resistance in the left gap is $2 Ω$ and unknown resistance in the right gap, the balance length is found to be 40 cm. On shunting the unknown resistance with $2 Ω$ , the balance length changes by [2024]