The width of one of the two slits in a Young’s double slit experiment is 4 times that of the other slit. The ratio of the maximum to the minimum intensity in the interference pattern is [2024]
1 : 1
16 : 1
9 : 1
4 : 1
(3)
So,
In Young's double slit experiment, light from two identical sources are superimposing on a screen. The path difference between the two lights reaching at a point on the screen is 7λ/4. The ratio of intensity of fringe at this point with respect to the maximum intensity of the fringe is [2024]
(4)
Two wavelengths and are used in Young's double slit experiment. = 450 nm and = 650 nm. The minimum order of fringe produced by which overlaps with the fringe produced by is . The value of is ____. [2024]
(9)
In Young’s double slit experiment, carried out with light of wavelength 5000 , the distance between the slits is 0.3 mm and the screen is at 200 cm from the slits. The central maximum is at = 0 cm. The value of for the third maxima is __________ mm. [2024]
(10)
Two slits are 1 mm apart and the screen is located 1 m away from the slits. A light of wavelength 500 nm is used. The width of each slit to obtain 10 maxima of the double slit pattern within the central maximum of the single slit pattern is ___ × m. [2024]
(2)
In a Young’s double slit experiment, the intensity at a point is of the maximum intensity. The minimum distance of the point from the central maximum is _____ µm.
(Given: ) [2024]
(200)
Monochromatic light of wavelength 500 nm is used in Young's double slit experiment. An interference pattern is obtained on a screen when one of the slits is covered with a very thin glass plate (refractive index = 1.5), the central maximum is shifted to a position previously occupied by the 4th bright fringe. The thickness of the glass plate is _____ µm. [2024]
(4) When glass plate is inserted, then the extra path difference will be
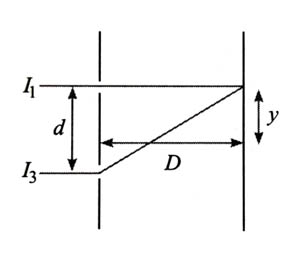
In a double slit experiment shown in figure, when light of wavelength 400 nm is used, dark fringe is observed at P. If D = 0.2 m the minimum distance between the slits and is ____ mm. [2024]

(0.20)
Path difference for minima at P
In Young’s double slit experiment, monochromatic light of wavelength 5000is used. The slits are 1.0 mm apart and the screen is placed at 1.0 m away from slits. The distance from the centre of the screen where intensity becomes half of the maximum intensity for the first time is ____ . [2024]
(125)
Let intensity of light on screen due to each slit is
So, intensity at centre of screen is
...(i)
Let intensity of light on screen due to each slit is
So intensity at centre of screen is
From (i):