Let
where [t] denotes greatest integer function. Then, [2023]
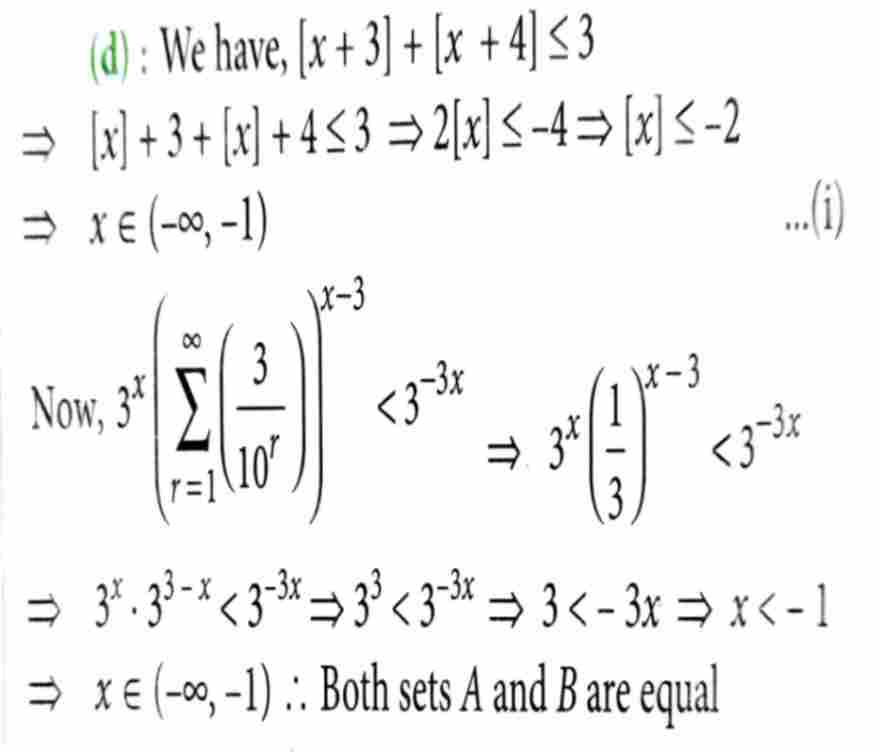
Let the sets A and B denote the domain and range respectively of the function , where denotes the smallest integer greater than or equal to x. Then among the statements
and
[2023]
only (S1) is true
both (S1) and (S2) are true
only (S2) is true
neither (S1) nor (S2) is true

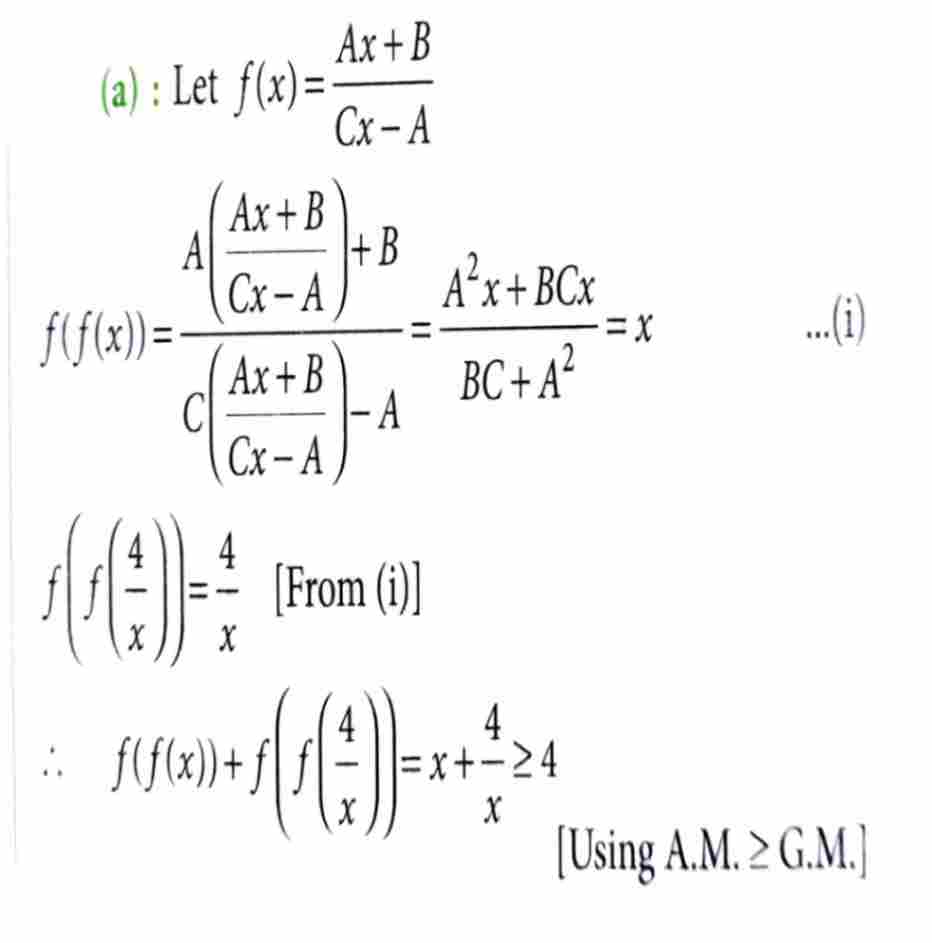
If then the least value of is [2023]
4
2
0
8
The number of integral solutions x of is [2023]
6
8
5
7
The domain of the function is (where [x] denotes the greatest integer less than or equal to x). [2023]
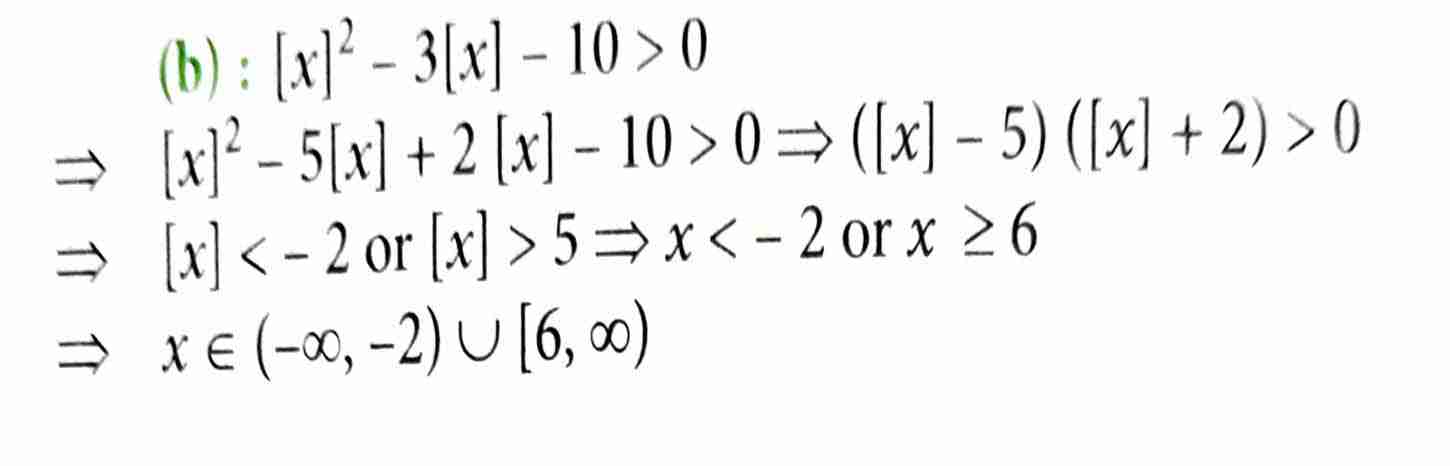
For , two real valued functions and are such that, and Then is equal to [2023]
5
1
0
- 3

Let be a function such that Then is equal to [2023]
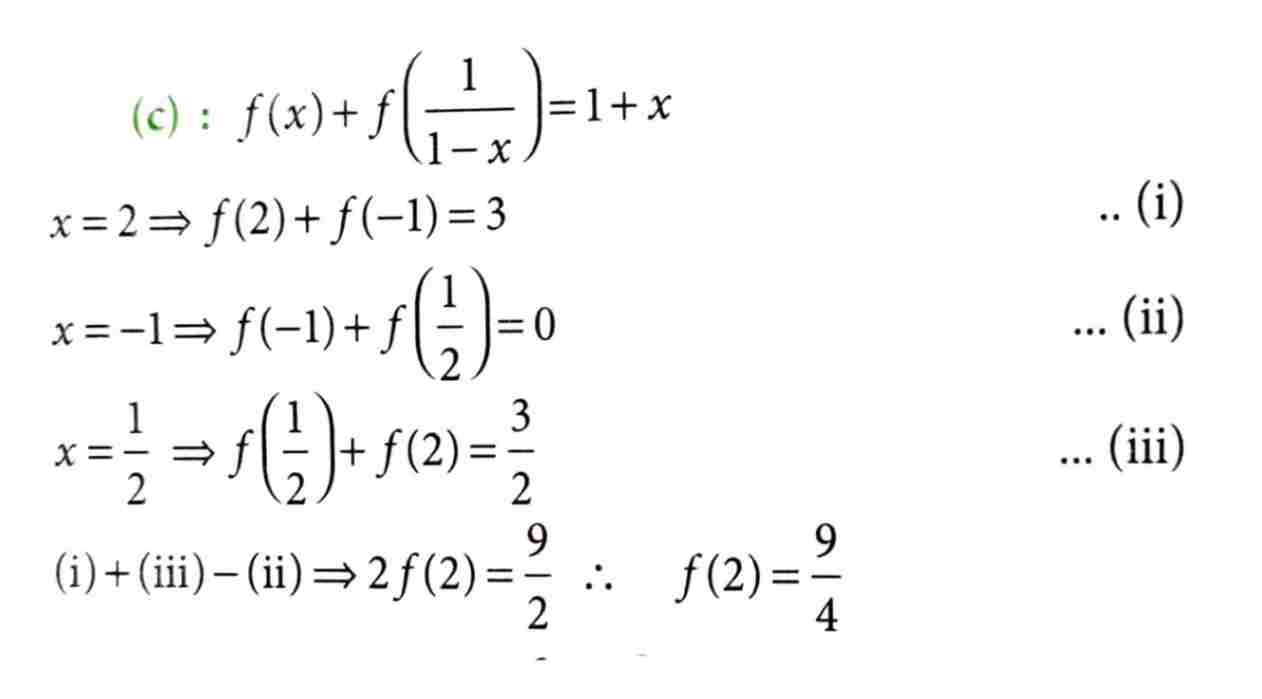
The equation where [x] denotes the greatest integer function, has [2023]
no solution
exactly two solutions in
a unique solution in
a unique solution in
(D)
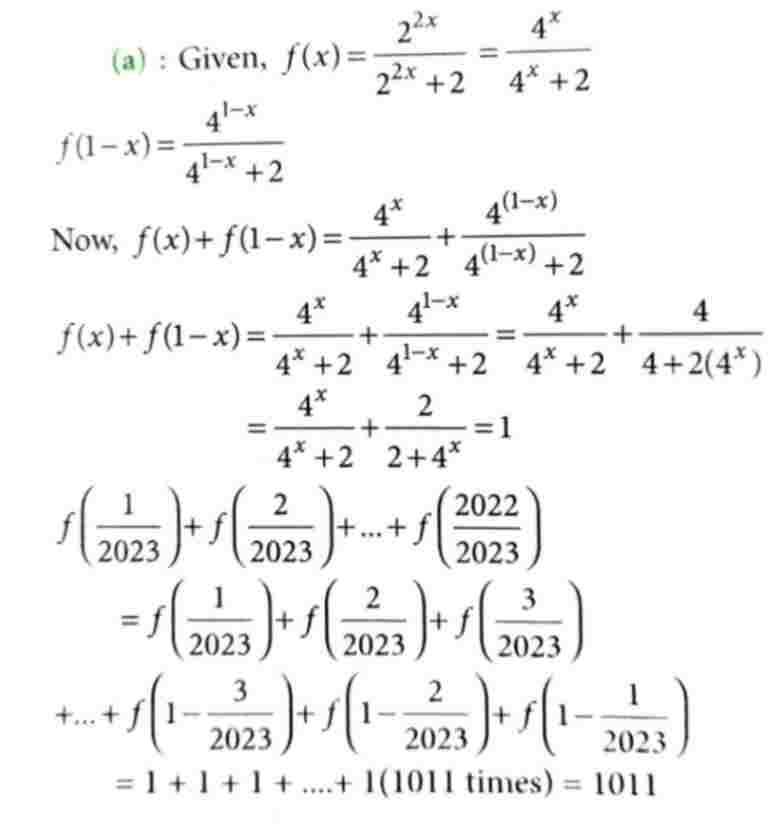
If then
is equal to [2023]
1011
2010
1010
2011
Let be a function such that for all If and then the value of n is [2023]
8
6
7
9
