An electric field is given by The electric flux through a surface area lying in YZ-plane (in SI unit) is [2024]
180
90
150
60
(1)
Electric flux,
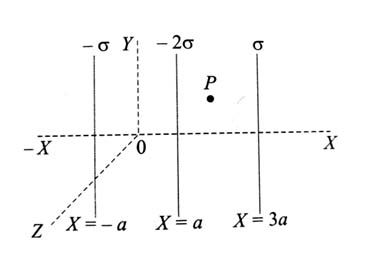
Three infinitely long charged thin sheets are placed as shown in figure. The magnitude of electric field at the point P is . The value of is _____ (all quantities are measured in Sl units). [2024]
(2)
A charge q is placed at the center of one of the surfaces of a cube. The flux linked with the cube is [2024]
(1)
Let us enclose the charge with an identical cube as shown,

Five charges +q, +5q, −2q, +3q and −4q are situated as shown in the figure. The electric flux due to this configuration through the surface S is [2024]
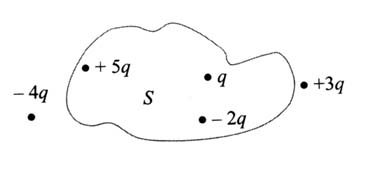
(2)
As per Gauss' theorem,
Two charges of 5Q and −2Q are situated at the points (3a,0) and (−5a,0) respectively. The electric flux through a sphere of radius 4a having center at origin is [2024]
(2)
If we draw the sphere, the charge -2Q will be outside of sphere and 5Q charge is inside the spherical region
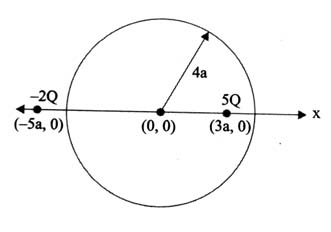
The flux through sphere
and are two hollow concentric cubes enclosing charges 2Q and 3Q respectively as shown in the figure. The ratio of electric flux passing through and is [2024]
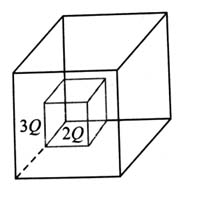
2 : 5
5 : 2
2 : 3
3 : 2
(1)
Flux through ,
Flux through ,
An infinite plane sheet of charge having uniform surface charge density is placed on the plane. Another infinitely long line charge having uniform linear charge density is placed at m plane and parallel to the y-axis. If the magnitude values then at point the ratio of magnitudes of electric field values due to sheet charge to that of line charge is . The value of is ______ . [2024]
(16)

An electric field, passes through the surface of area having unit vector The electric flux for that surface is ____ Vm. [2024]
(12)
An electric field exists in space. A cube of side 2 m is placed in the space as per figure given below. The electric flux through the cube is _____ [2024]
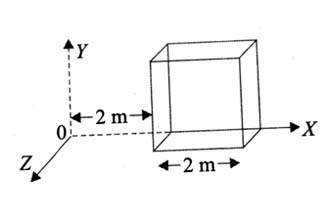
(16)
Given
