Topic Question Set
Q 1
:
A wire of length and radius is clamped at one end. If its other end is pulled by a force , its length increases by . If the radius of the wire and the applied force both are reduced to half of their original values keeping original length constant, the increase in length will become [2024]
3 times
4 times
times
2 times
(4)
Young's modulus,
In case I : ...(i)
In case II : ...(ii)
From (i) and (ii),
Q 2
:
Young's modulus of material of a wire of length 'L' and cross-sectional area A is Y. If the length of the wire is doubled and cross-sectional area is halved then Young's modulus will be [2024]
(3)
Young's modulus depends on the material not on length and cross-sectional area. So young's modulus remains same.
Q 3
:
With rise in temperature, the Young's modulus of elasticity [2024]
changes erratically
decreases
increases
remains unchanged
(2)
as increases, decreases
Q 4
:
A wire of cross-sectional area A, modulus of elasticity and length 2 m is stretched between two vertical rigid supports. When a mass of 2 kg is suspended at the middle, it sags lower from its original position, making an angle radian on the points of support. The value of A is _____ (consider ). [2024]
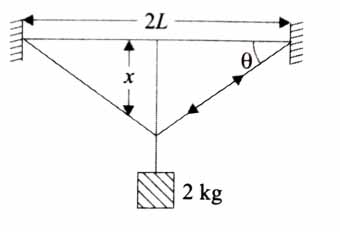

(1)
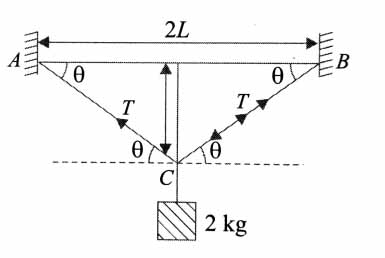
Change in length,
Q 5
:
Two persons pull a wire towards themselves. Each person exerts a force of 200 N on the wire.
Young’s modulus of the material of the wire is . Original length of the wire is 2 m and the area of cross-section is 2 . The wire will extend in length by _____ μm. [2024]
(20)

Q 6
:
Two metallic wires P and Q have the same volume and are made up of the same material. If their area of cross-sections are in the ratio 4:1 and force is applied to P, an extension of is produced. The force required to produce the same extension in Q is . The value of is _____. [2024]
(16)
Y and V is same for both the wires
Q 7
:
Each of three blocks P, Q, and R shown in the figure has a mass of 3 kg. Each of the wires A and B has a cross-sectional area of 0.005 and Young’s modulus . Neglecting friction, the longitudinal strain on wire B is _____ . (Take g = 10 ). [2024]
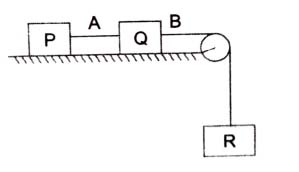

(2)
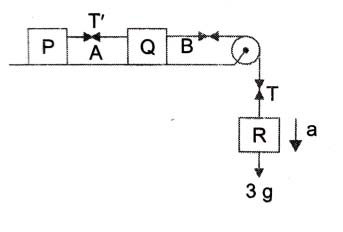
Q 8
:
Two blocks of mass 2 kg and 4 kg are connected by a metal wire going over a smooth pulley as shown in the figure. The radius of the wire is m and Young’s modulus of the metal is . The longitudinal strain developed in the wire is . The value of is _______ .
[Use g = 10 ] [2024]


(12)
Q 9
:
One end of a metal wire is fixed to a ceiling, and a load of 2 kg hangs from the other end. A similar wire is attached to the bottom of the load, and another load of 1 kg hangs from this lower wire. Then the ratio of longitudinal strain of the upper wire to that of the lower wire will be _____.
[Area of cross-section of wire = 0.005 , Y = and g = 10 ] [2024]
(3)
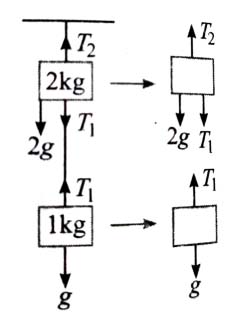
...(i)
Q 10
:
Two wires A and B are made of same material having ratio of lengths and their diameters ratio . If both the wires are stretched using same force, what would be the ratio of their respective elongations? [2025]
1 : 6
1 : 12
3 : 4
1 : 3
(2)
and
Given and

