If ABC ~ EDF and ABC is not similar to DEF, then which of the following is not true ?
BC. EF = AC. FD
AB. EF = AC. DE
BC. DE = AB. EF
BC. DE = AB. FD
(3)
Since, ABC ~ EDF
Therefore, the ratio of their corresponding sides is proportional.
In the ABC, DE BC and AD = 3x − 2, AE = 5x − 4, BD = 7x − 5, CE = 5x − 3, then find the value of x
1
7/10
both (a) & (b)
none of these
(3)
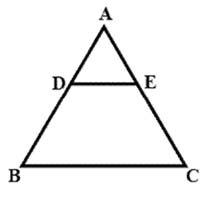
Given that, AD = 3x − 2, AE = 5x − 4, BD = 7x − 5, CE = 5x − 3
By Basic Proportionality theorem, we have
In the given figure, DE BC, AE = a units, EC = b units, DE = x units and BC = y units. Which of the following is true?
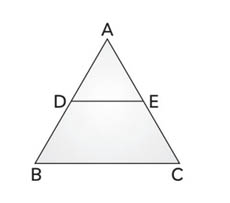
(3)
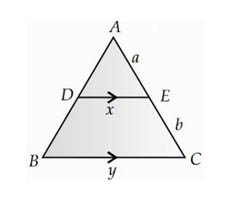
As
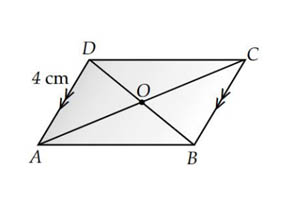
ABCD is a trapezium with ADBC and AD = 4cm. If the diagonals AC and BD intersect each other at O such that AO/OC = DO/OB =1/2, then BC =
6 cm
7 cm
8 cm
9 cm
(3)
and
. If AM and PN are altitudes of and respectively and = 4 : 9, then AM : PN =
3 : 2
16 : 81
4 : 9
2 : 3
(4)
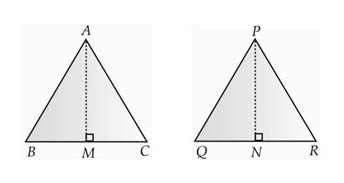
We have,
But
i.e.,
Hence,
The perimeters of two similar triangles ABC and PQR are 56 cm and 48 cm respectively. PQ/AB is equal to
7/8
6/7
7/6
8/7
(2)
The ratio of the corresponding sides of similar triangles is same as the ratio of their perimeter
In the given figure, if M and N are points on the sides OP and OS respectively of , such that MNPS, then the length of OP is :

6.8 cm
17 cm
15.3 cm
9.6 cm
(3) 15.3 cm
In the given figure is shown. DE is parallel to BC. If AD = 5 cm, DB = 2.5 cm and BC = 12 cm, then DE is equal to
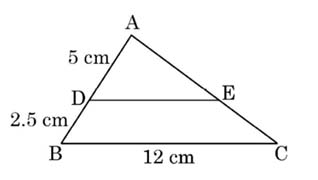
10 cm
6 cm
8 cm
7.5 cm
(3)