Topic Question Set
Q 1
:
The co-ordinates of a particle moving in plane is given by
The motion of the particle is [2024]
uniform motion along a straight line
uniformly accelerated having motion along a parabolic path
non-uniformly accelerated
uniformly accelerated having motion along a straight line
(2)
Given, and
constant, hence
and also,
the motion will be uniformly accelerated motion.
Also,
This is a quadratic equation so path will be parabola, parabolic path.
Q 2
:
Position of an ant (S in metres) moving in Y-Z plane is given by (where is in second). The magnitude and direction of velocity of the ant at s will be [2024]
16 m/s in -direction
4 m/s in -direction
9 m/s in -direction
4 m/s in -direction
(4)
Q 3
:
A particle starts from origin at with a velocity m/s and moves in plane under action of a force which produces a constant acceleration of . If the x-coordinate of the particle at that instant is 84 m, then the speed of the particle at this time is The value of is ____________ . [2024]
(673) Given, and
Q 4
:
A cyclist starts from the point P of a circular ground of radius 2 km and travels along its circumference to the point S.
The displacement of the cyclist is ____. [2024]
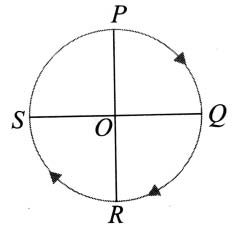

4 km
6 km
km
8 km
(3)


