If the range of is then the sum of the infinite G.P., whose first term is 64 and the common ratio is is equal to _______. [2024]
(96)
We have,
Now,
When
Sum of infinite G.P. with first term 64 and common ratio
If three successive terms of a G.P. with common ratio are the lengths of the sides of a triangle and denotes the greatest integer less than or equal to then is equal to ______. [2024]
(1)
Let and be the three sides of the triangle.
Now,
So,
If then the value of is _______ . [2024]
(9)
...(i)
Multiplying both sides by we get ...(ii)
Subtracting (ii) from (i), we get
for infinite geometric series
Let the coefficient of in the expansion of be If then the value of equals ______ . [2024]
(25)
We have,
Let ABC be an equilateral triangle. A new triangle is formed by joining the middle points of all sides of the triangle ABC and the same process is repeated infinitely many times. If P is the sum of perimeters and Q is the sum of areas of all the triangles formed in this process, then: [2024]
(3)
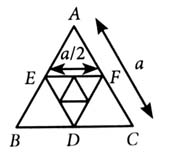
Now, is made by joining midpoints of the sides of
Now,
Let be a G.P. of increasing positive numbers. If and , then is equal to [2025]
131
129
128
130
(2)
Let be the first term and be the common ratio of GP respectively.
Given,
... (i)
and
... (ii)
Now, divide equation (i) by equation (ii), we get
[ G.P. is an increasing series]
Substitute = 6 in equation (ii), we get
Now,
= 3(43) = 129.
Let be in a geometric progression. If 2, 7, 9, 5 are subtracted respectively from , then the resulting numbers are in an arithmetic progression. Then the value of is : [2025]
36
216
72
18
(2)
Given, be in a G.P.
According to question
are in A.P.
So, ... (i)
... (ii)
Solving (i) and (ii), we get r = 2, a = – 3
Product
The value of .
If the sum of the second, fourth and sixth terms of a G.P. of positive terms is 21 and the sum of its eighth, tenth and twelfth terms is 15309, then the sum of its first nine terms is : [2025]
760
755
750
757
(4)
We have,
... (i)
and ... (ii)
Divide equation (ii) by (i), we get
Now,
.
Let be a G.P. of increasing positive terms. If and , then is equal to : [2025]
812
526
784
628
(3)
Here, r > 0 and as G.P. has increasing positive terms.
(Given)
... (i)
Also,
... (ii)
From (i) and (ii), we get
.
Let be a sequence such that and . Then is equal to . [2025]
(2)
We have, and
Let
Put n = 0; 0 = A + B
.