If are two distinct complex number such that , then [2024]
lies on a circle of radius and lies on a circle of radius 1.
either lies on a circle of radius 1 or lies on a circle of radius
both and lie on the same circle.
either lies on a circle of radius or lies on a circle of radius 1.
(2)
We have,
Let and respectively be the modulus and amplitude of the complex number then is equal to [2024]
(2)
We have,
So,
Let the complex numbers and lie on the circles and respectively, where Then, the value of is ________. [2024]
(20)
Given ...(i)
...(ii)
lies on (i),
...(iii)
lies on (ii)
...(iv)
From (iii) and (iv), we get
If denotes the number of solutions of and where then the distance of the point from the line is __________ [2024]
(3)
We have,
and where
and
Now, the distance of point from the line is given by
The area (in sq. units) of the region
is [2024]
(2)
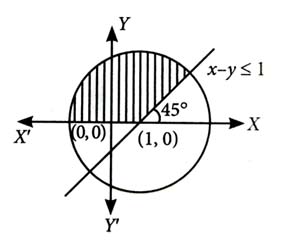
Let , and Then the area of the region is : [2024]
(1)
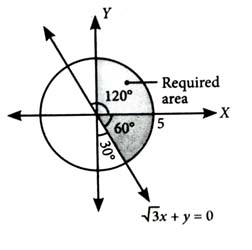
...(i)
...(ii)
...(iii)
The sum of the square of the modulus of the elements in the set is ____________. [2024]
(9)
Let z be a complex number such that |z| = 1. If , then the maximum distance of from the circle |z – (1 + 2i)| = 1 is : [2025]
3
2
(3)
We have,
[]
[]
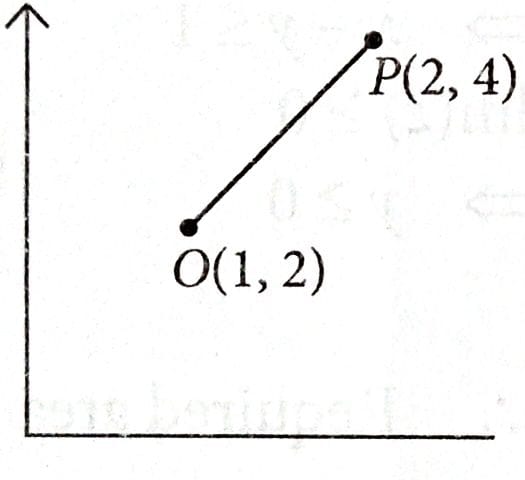
The centre of the given circle is (1, 2) and its radius is 1.
Now, .
Maximum distance = .
If are the vertices of an equilateral triangle, whose centroid is , then is equal to [2025]
0
i
– i
1
(1)
Centroid of triangle is , then
[ For equilateral ]
Now,
.
If the locus of , such that , is a circle of radius r and center (a, b), then is equal to: [2025]
12
16
24
18
(4)
We have,
Let z = x + iy
.