(11)

We have, $\frac{dy}{dx}=\frac{x+y–2}{x–y}$

Substitute x = X + h and y = Y + k

$\Rightarrow \frac{dY}{dX}=\frac{X+Y+h+k–2}{X–Y+h–k}$

Put h + k – 2 = 0 and h – k = 0

$\Rightarrow$ h = 1 and k = 1

$\therefore \frac{dY}{dX}=\frac{X+Y}{X–Y}$

Now, put Y = vX $\Rightarrow \frac{dY}{dX}=v+X\frac{dv}{dX}$

$\therefore v+X\frac{dv}{dX}=\frac{X+vX}{X–vX}=\frac{1+v}{1–v}$

$\Rightarrow X\frac{dv}{dX}=\frac{1+v}{1–v}–v=\frac{1+v–v+v^2}{1–v}=\frac{1+v^2}{1–v}$

$\Rightarrow \int \frac{1–v}{1+v^2}dv=\int \frac{dX}{X} \Rightarrow \int \frac{1}{1+v^2}dv–\int \frac{v}{1+v^2}dv=\int \frac{dX}{X}$

$\Rightarrow {\tan }^{–1}v–\frac{1}{2}{\log }_e|1+v^2|=\log \left|X\right|+c$

$\Rightarrow {\tan }^{–1}\left(\frac{y–1}{x–1}\right)–\frac{1}{2}{\log }_e$$|1+{\left(\frac{y–1}{x–1}\right)}^2|$$=\log$$|x–1|$$+c$          ... (i)

Equation (i) passing through (2, 1), then

${\tan }^{–1}\left(\frac{1–1}{2–1}\right)–\frac{1}{2}{\log }_e$$|1+{\left(\frac{1–1}{2–1}\right)}^2|$$={\log }_e(2–1)+c$

$\Rightarrow {\log }_{e}1+c=0 \Rightarrow c=0$

$\therefore {\tan }^{–1}\left(\frac{y–1}{x–1}\right)–\frac{1}{2}{\log }_e|1+{\left(\frac{y–1}{x–1}\right)}^2|={\log }_e|x–1|$

By comparing with

${\tan }^{–1}\left(\frac{y–1}{x–1}\right)–\frac{1}{\beta }{\log }_e$$|\alpha +{\left(\frac{y–1}{x–1}\right)}^2|$$={\log }_e$$|x–1|$

$\beta =2, \alpha =1$

$\therefore 5\beta +\alpha =5\times 2+1=11$.

(3)

We have, $(1+y^2)(1+{\log }_e x)dx+xdy=0$

$\Rightarrow \frac{dy}{1+y^2}=–\frac{(1+{\log }_e x)dx}{x}$

Integrating both sides, we get

${\tan }^{–1}y=–\frac{1}{2}{(1+{\log }_e x)}^2+C$                        ...(i)

At (1, 1), $\frac{\mathrm{\pi }}{4}=\frac{–1}{2}+C \Rightarrow C=\frac{\mathrm{\pi }}{4}+\frac{1}{2}$

$\therefore y=\tan \{\frac{\mathrm{\pi }}{4}+\frac{1}{2}[1–{(1+{\log }_e x)}^2\left]\right\}$

$\Rightarrow y\left(e\right)=\tan (\frac{\mathrm{\pi }}{4}+\frac{1}{2}–2)$

$=\tan [\frac{\mathrm{\pi }}{4}–\frac{3}{2}]=\frac{\tan {\displaystyle \frac{\mathrm{\pi }}{4}}–\tan {\displaystyle \frac{3}{2}}}{1+\tan \frac{\mathrm{\pi }}{4}\tan \frac{3}{2}}=\frac{1–\tan {\displaystyle \frac{3}{2}}}{1+\tan \frac{3}{2}}$

So, $\alpha$ = 1 and $\beta$ = 1

$\therefore \alpha +2\beta =3$.

(2)

$f\left(x\right)=\sqrt{\underset{r\to x}{\lim }\{\frac{2r^2\left(\right(f{\left(r\right))}^2–f\left(x\right)f\left(r\right))}{r^2–x^2}–r^3{e}^{f\left(r\right)/r}\}}$

Using L'Hospital rule, we have

$f\left(x\right)=\sqrt{\underset{r\to x}{\lim }\{\frac{2r^2[2f\left(r\right)·f\text{'}(r)–f(x)·f\text{'}(r\left)\right]+[f{\left(r\right)}^2–f(x)·f(r\left)\right]·4r}{2r}–r^3{e}^{\frac{f\left(r\right)}{r}}\}}$

$=\sqrt{\frac{2x^2[2f\left(x\right)·f\text{'}(x)–f(x)·f\text{'}(x\left)\right]+0}{2x}–x^3{e}^{\frac{f\left(x\right)}{x}}}$

$\therefore f\left(x\right)=\sqrt{xf\left(x\right)·f\text{'}\left(x\right)–x^3·e^{\frac{f\left(x\right)}{x}}}$

Let y = f(x)

$y^2=xy·\frac{dy}{dx}–x^3{e}^{\frac{y}{x}} \Rightarrow \frac{dy}{dx}=\frac{y^2+x^3{e}^{y/x}}{xy}$

Put $y=vx \Rightarrow \frac{dy}{dx}=v+x\frac{dv}{dx}$

$\Rightarrow \frac{v^2{x}^2+x^3{e}^{{\displaystyle \frac{vx}{x}}}}{x·vx}=v+x\frac{dv}{dx} \Rightarrow \frac{v^2+x{e}^v}{v}–v=x\frac{dv}{dx}$

$\Rightarrow \frac{e^v}{v}=\frac{dv}{dx} \Rightarrow dx=v{e}^{–v}dv$

$\Rightarrow \int dx=\int v·e^{–v}dv \Rightarrow x+c=–e^{–v}(v+1)$

$\Rightarrow x+c=–e^{–y/x}(\frac{y}{x}+1)$          ... (i)

$\because y\left(1\right)=1$

$\Rightarrow 1+c=–e^{–1}\left(2\right)$

$\Rightarrow c=–1–\frac{2}{e}$          ... (ii)

Now, f(a) = 0

$\therefore a+c=–e^{–0/a}(\frac{0}{a}+1)$          [Using (i)]

a + c = –1

$a=–1–c=–1+1+\frac{2}{e}=\frac{2}{e}$          [Using (ii)]

$\therefore$ ea = 2.

(97)

We have, $(1–x^2)dy=[xy+(x^3+2\left)\sqrt{3(1–x^2)}\right]dx$

$\Rightarrow \frac{dy}{dx}=\frac{x}{(1–x^2)}y+\frac{(x^3+2)\sqrt{3–3x^2}}{(1–x^2)}$

$\Rightarrow \frac{dy}{dx}–\frac{x}{1–x^2}y=\frac{(x^3+2)\sqrt{3–3x^2}}{1–x^2}$

$\mathrm{I}.\mathrm{F}.=e^{\int \frac{–x}{1–x^2}dx}$

Put $1–x^2=t \Rightarrow –xdx=\frac{dt}{2}$

$\Rightarrow \mathrm{I}.\mathrm{F}. = e^{\int \frac{dt}{2t}}=e^{\frac{1}{2}\ln t}=e^{\frac{1}{2}\ln (1–x^2)}$

$=e^{\ln {(1–x^2)}^{\frac{1}{2}}}={(1–x^2)}^{\frac{1}{2}}=\sqrt{1–x^2}$

$\therefore y\sqrt{(1–x^2)}=\int \frac{(x^3+2)\sqrt{3(1–x^2)}}{(1–x^2)}\sqrt{1–x^2}dx$

$\Rightarrow y\sqrt{1–x^2}=\sqrt{3}\int (x^3+2)dx$

$\Rightarrow y\sqrt{1–x^2}=\sqrt{3}[\frac{x^4}{4}+2x]+c$

Since, y(0) = 0, so $0=c \Rightarrow y=\sqrt{\frac{3}{1–x^2}}(\frac{x^4}{4}+2x)$

Now, $y\left(\frac{1}{2}\right)=\sqrt{\frac{3}{1–{\displaystyle \frac{1}{4}}}}(\frac{{\left({\displaystyle \frac{1}{2}}\right)}^4}{4}+2\times \frac{1}{2})=2(\frac{1}{64}+1)$

$=2\left(\frac{65}{64}\right)=\frac{65}{32}=\frac{m}{n}$

$\therefore$ m = 65, n = 32 $\Rightarrow$ m + n = 65 +32 = 97.

(20)

We have, curve : Y = y(x)

Line : Y – y = Y'(x)(X – x)

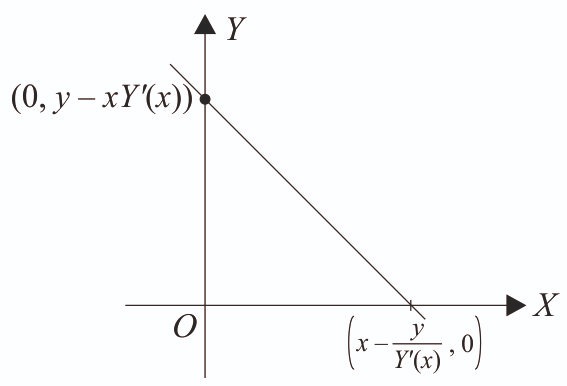
Area = $\frac{1}{2}(x–\frac{y}{Y\text{'}\left(x\right)})(y–xY\text{'}(x\left)\right)$

$\Rightarrow 1–\frac{y^2}{2Y\text{'}\left(x\right)}=\frac{(xY\text{'}(x)–y)(y–xY\text{'}(x\left)\right)}{2Y\text{'}\left(x\right)}$

$\Rightarrow \frac{2Y\text{'}\left(x\right)–y^2}{2Y\text{'}\left(x\right)}=\frac{(xY\text{'}(x)–y)(y–xY\text{'}(x\left)\right)}{2Y\text{'}\left(x\right)}$

$\Rightarrow 2Y\text{'}\left(x\right)–y^2=xy Y\text{'}\left(x\right)–x^2{[Y\text{'}(x\left)\right]}^2–y^2+xy Y\text{'}\left(x\right)$

$\Rightarrow x^2{[Y\text{'}(x\left)\right]}^2+Y\text{'}\left(x\right)[2–xy–xy]=0$

$\Rightarrow Y\text{'}\left(x\right)[x^{2}Y\text{'}(x)+2–2xy]=0$

$\Rightarrow x^{2}Y\text{'}\left(x\right)+2–2xy=0 \mathrm{or} Y\text{'}\left(x\right)=\frac{2xy–2}{x^2}$

$\Rightarrow \frac{dy}{dx}=\left(\frac{2}{x}\right)y–\frac{2}{x^2} \Rightarrow \frac{dy}{dx}–\left(\frac{2}{x}\right)y=\frac{–2}{x^2}$

$\mathrm{I}.\mathrm{F}.=e^{–\int \frac{2}{x}dx}=e^{–2 \log x}=\frac{1}{x^2}$

Solution is

$y·\frac{1}{x^2}=\int \frac{–2}{x^2}\times \frac{1}{x^2}dx=–2\int \frac{1}{x^4}dx \mathrm{or} \frac{y}{x^2}=\frac{2}{3x^3}+c$

$y=\frac{2}{3x}+c{x}^2$          ... (i)

$1=\frac{2}{3}+c \Rightarrow c=\frac{1}{3}$

At x = 2,

$\therefore y=\frac{2}{3\times 2}+\frac{1}{3}\times 4=\frac{1}{3}+\frac{4}{3}=\frac{5}{3} \Rightarrow 12y=20$.

(9)

We have, ${\sec }^{2}xdx+(e^{2y}{\tan }^{2}x+\tan x)dy=0$

Put $\tan x=t$

$\Rightarrow {\sec }^{2}x\frac{dx}{dy}=\frac{dt}{dy}$

Now, ${\sec }^{2}x\frac{dx}{dy}+(e^{2y}{\tan }^{2}x+\tan x)=0$

$\Rightarrow \frac{dt}{dy}+e^{2y}{t}^2+t=0 \Rightarrow \frac{dt}{dy}+t=–t^2{e}^{2y}$

$\frac{1}{t^2}\frac{dt}{dy}+\frac{1}{t}=–e^{2y}$

Again put $\frac{1}{t}=u \Rightarrow \frac{–1}{t^2}\frac{dt}{dy}=\frac{du}{dy}$

$\therefore –\frac{du}{dy}+u=–e^{2y} \Rightarrow \frac{du}{dy}–u=e^{2y}$

$\therefore \mathrm{I}.\mathrm{F}.=e^{–\int dy}=e^{–y}$

$\therefore u{e}^{–y}=\int e^{–y}{e}^{2y}dy$

$\Rightarrow u{e}^{–y}=\int e^{y}dy \Rightarrow \frac{1}{t}{e}^{–y}=e^y+c$

$\Rightarrow \frac{1}{\tan x}{e}^{–y}=e^y+c$          ... (i)

When, $x=\frac{\mathrm{\pi }}{4}$, y = 0

$\therefore \frac{1}{\tan {\displaystyle \left(\frac{\mathrm{\pi }}{4}\right)}}{e}^{–0}=e^0+c \Rightarrow 1=1+c \Rightarrow c=0$

Also, when $x=\frac{\mathrm{\pi }}{6}, y=\alpha$

$\therefore$  From (i), we have

$\frac{1}{\tan {\displaystyle \frac{\mathrm{\pi }}{6}}}{e}^{–\alpha }=e^{\alpha }+0 \Rightarrow \sqrt{3}=e^{2\alpha } \Rightarrow {\left(e^{2\alpha }\right)}^4={\left(\sqrt{3}\right)}^4$

$\Rightarrow e^{8\alpha }=9$.