Two circles touch each other externally at C and AB is common tangent of circles, then is
70°
60°
100°
90°
(4)
Draw CM perpendicular to AB.
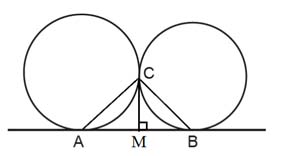
Now, AM = MC and MB = MC (tangents drawn from external point are equal).
⇒ AM = MC
⇒ = = 45°
(Since Δ AMC is right triangle)
∴ Also, MB = MC ⇒ = = 45° (Since Δ MBC is right angle triangle)
∴ = + = 45° + 45° = 90° ⇒ = 90°
If the circumference of a circle and the perimeter of a square are equal, then
Area of the circle = Area of the square
Area of the circle > Area of the square
Area of the circle < Area of the square
Nothing definite can be said about the relation between the areas of the circle and square.
(2)
Area of the circle > Area of the square
Assertion (A): The tangents drawn at the end points of a diameter of a circle, are parallel.
Reason (R): Diameter of a circle is the longest chord.
Both, Assertion (A) and Reason (R) are true and Reason (R) is correct explanation of Assertion (A).
Both, Assertion (A) and Reason (R) are true but Reason (R) is not correct explanation for Assertion (A).
Assertion (A) is true but Reason (R) is false.
Assertion (A) is false but Reason (R) is true.
(2)
Assertion: Two parallel tangents always lie at the end points of the diameter of the circle.
Reason: Diameter is the longest chord of a circle which passes through centre joining the two points on the circumference of a circle.
Both, Assertion (A) and Reason (R) are true but Reason (R) is not correct explanation for Assertion (A).
The area of the circle that can be inscribed in a square of 6cm is
(4)
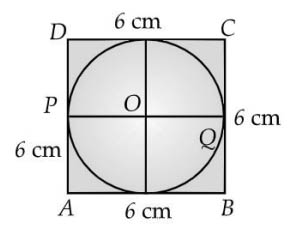
ABCD is a square of side 6 cm. PQ is a diameter of the given circle such that
If two tangents inclined at an angle of are drawn to a circle of radius 3cm, then the length of each tangent is equal to
(4)
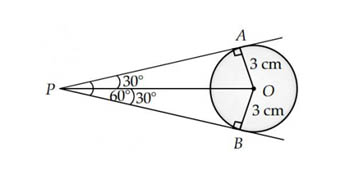
Angle between two tangents = (given)
Tangents are equally inclined to each other
and
(Tangent is perpendicular to the radius)
In
Hence, the length of each tangent is cm
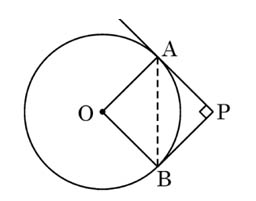
In the given figure, tangents PA and PB to the circle centred at O, from point P are perpendicular to each other. If PA = 5 cm, then length of AB is equal to
cm
cm
cm
cm
(2)
PA = PB (Tangents from an external point P)
is a Right angle Triangle
In the given figure, PA and PB are two tangents drawn to the circle with centre O and radius 5 cm. If , then the length of PA is :

(2)
AB and CD are two chords of a circle intersecting at P. Choose the correct statement from the following:
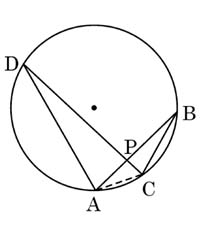
(4)
[vertically opposite angle]
[Angle subtends on the same segment]
(by AA similarity)
In the given figure, AT is tangent to a circle centred at O. If , then is equal to
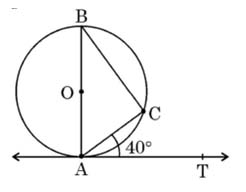
70°
50°
65°
40°
(4)
Given,
Assertion (A): TA and TB are two tangents drawn from an external point T to a circle with centre ‘O’. If then .
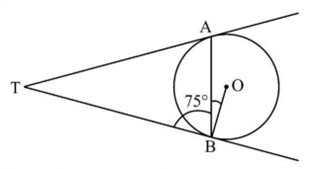
Reason (R): The tangent drawn at any point of a circle is perpendicular to the radius through the point of contact.
Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of the Assertion (A).
Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of the Assertion (A).
Assertion (A) is true, but Reason (R) is false.
Assertion (A) is false, but Reason (R) is true.
(4) Assertion (A) is false, but Reason (R) is true.