The pair of linear equations 2x + 3y = 5 and 4x + 6y = 10 is
inconsistent
consistent
dependent consistent
none of these
(3)
Dependent Consistent
If the system of equations is inconsistent, then k =
-1
0
1
2
(4)
...(i)
and ...(ii)
Comparing eq. (i) with and eq. (ii)
with we get
and
Since, system is inconsistent, then
Hence, the value of is 2.
In the given figure, graphs of two linear equations are shown. The pair of these linear equations is:
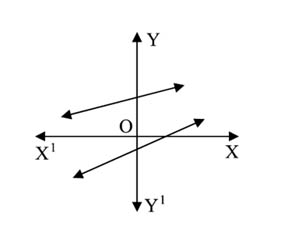
consistent with unique solution.
consistent with infinitely many solutions.
inconsistent.
inconsistent but can be made consistent by extending these lines.
(4) inconsistent but can be made consistent by extending these lines.
The pair of equations x = 2a and y = 3b graphically represents straight lines which are :
coincident
parallel
intersecting at (2a, 3b)
intersecting at (3b, 2a)
(3) intersecting at (2a, 3b)
The value of k for which the system of equations 3x – y + 8 = 0 and 6x – ky + 10 = 0 has infinitely many solutions, is
– 2
2
1/2
− 1/2
(2)
Given equation are and
Here,
For Infinite many solution,